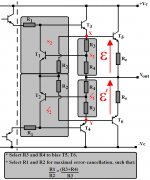
Given the former, further insight in the remarkable linearity of Hawksford/Cordell may be gained by comparing the basic block diagram with the nominal practical implementation given below.
Clearly the linearity of summer S1 is beyond reproach since it consists entirely of resistors! 🙂
Summer S2, on the other hand, consists of an active element, T1, and two resistors, R1 and R2.
The presence of T1 may at first appear dubious in respect of linearity, but this notion is quickly dispelled by the realisation that its non-linearity is significantly ameliorated by the heavy local feedback provided by emitter degenerative resistor R2. Indeed, in Bob's circuit this transistor is only required to generate a nominal gain of 2.
To my mind, the foregoing explains the remarkable practical performance obtained by Bob's circuit.
Nevertheless, it may be taken as axiomatic that residual summer non-linearity in the correction loop is entirely due to transistor T1.
This, of course, also applies to the negative polarity arrangement of transistor T2.
Clearly the linearity of summer S1 is beyond reproach since it consists entirely of resistors! 🙂
Summer S2, on the other hand, consists of an active element, T1, and two resistors, R1 and R2.
The presence of T1 may at first appear dubious in respect of linearity, but this notion is quickly dispelled by the realisation that its non-linearity is significantly ameliorated by the heavy local feedback provided by emitter degenerative resistor R2. Indeed, in Bob's circuit this transistor is only required to generate a nominal gain of 2.
To my mind, the foregoing explains the remarkable practical performance obtained by Bob's circuit.
Nevertheless, it may be taken as axiomatic that residual summer non-linearity in the correction loop is entirely due to transistor T1.
This, of course, also applies to the negative polarity arrangement of transistor T2.
Attachments
Bob wrote:
🙂 Earlier in this thread you were saying how great it was to be able to tune the distortion to a minimum. I agree with what you say...once you've tuned it you can replce the pot with a precision resistor. The tuning is necessary in the first place because the PFB is so sensitive to resistor values. I can't see why you would choose to have this step *if* you were able to use an integrator in the circuit.
mikeks said:
I am deadly serious Michael, please review.
If someone else here coincides that in a linear (constant or at most frequency dependent coeficients) representation of a system where errors / disturbances are introduced as external perturbations, system gain is possibly dependent on the magnitude of this perturbations, then please share.
Rodolfo
powerbecker said:
The old paint-copy-paste trick, but I suspect the actual log file (plain text) is also dumped on disk, did not check.
Rodolfo
ingrast said:
Hi Rodolfo,
Perhaps this is yet another case of misunderstanding.
I have reviewed your figure 3 below extracted from here, with 1/A' set to unity.
Now, noting that S must be unity at balance, what is S2's output when A is exactly unity?
Otherwise, what happens to your major-loop gain when A is exactly unity?
Attachments
Bob wrote:
I share Nixie's experiences. There have been many "low THD" designs on the market that have not satisfied sonically and vice versa. I have followed a process of measure, listen, improve the measure, improve the circuit, listen, improve the measure... This process has taught me that basic THD is only half the story.
mikeks said:
I bet it is misunderstanding.
By the very nature of the error correction approach, output of S2 when A=A' (which is the same to say unity gain plant and bypass of 1/A') is null. This is only to be expected and lends to Jan's insight, no error ->no feedback. It mus be understood the fact there is no error signal does not mean at all there is no gain or whatever.
What brings confussion to this simplified approach is the fact the inner loop embodies in fact a singularity, making simplified calculations including balance from the outset to fall appart.
I was from the start cautious to not include directly the cancellation condition, for doing so is what brings chaos. Further, recognizing at least one element in the inner loop chain must be active (I considered natural S(s)) the singularity automatically disappears except for s=0.
An please, it was you who was pulling my leg with the dimensions issue, weren't you?
Rodolfo
traderbam said:
Brain, Mike,
In practise, the 'gain' of the ec transistors in Bob's circuit is actually Ic/Ie multiplied by the resistor ratio mentioned by Mike. Since Ic/Ie is probably much less deviation from '1' than 1%, using 1% resistors effectively make the (non)linearity of the transistors a secondary effect. Especially when they are biased at a DC current which probably is larger than any practical error current variations: they work in class A. No?
Jan Didden
janneman said:
Not really. See:
http://www.diyaudio.com/forums/showthread.php?postid=1071612#post1071612
and
http://www.diyaudio.com/forums/showthread.php?postid=1073471#post1073471
traderbam said:
Brian, it's just so much fun to tune the circuit and watch the error go through zero!
Cheers,
Bob
Bob Cordell said:
Of interest, Bob, over what bandwidth does this effect extend in your design?
The effect itself is perhaps not surprising considering the high linearity of the loop.
ingrast said:
The small-signal gains within the EC loop(s) are largely independent of the amplitude of the errors, as long as clipping is not approached. Rodolfo is correct.
Bob
Bob Cordell said:
Some misunderstanding here.
The individual ''small-signal gains within the EC loop(s)'' are certainly ''largely independent of the amplitude of the errors, as long as clipping is not approached'', as i have pointed out here.
However, this was not the issue referred to by Rodolfo and i, here, here and here.
Clearly the matter was about the major loop, and not individual ''small-signal gains''.
On this basis, therefore, i fail to see how Rodolfo could possibly be correct.
powerbecker said:
I change some parts: THD20 is now 0.000006%
Yes, I know...it´s only a simulation...
The amp is so simple that it fits to the NP-forum, but I´m afraid not for the sound 😉
Heinz!
powerbecker said:
Thank you, Heinz!
I like your circuit, very much.
I added a notch filter to my original circuit's ec loop, and was then able to increase the gain enough to get .000119% THD-20. But, also, the step response is still very good (after carefully configuring the filter). The new circuit should be attached. Otherwise, it should be able to be downloaded from http://www.fullnet.com/~tomg/ec_amp-tg2.asc.txt .
I would like to try to convert it into a similar topology as yours, but still keep the good step response.
Sorry about all of the parasitic capacitors, et al. It's just a habit, now.
Thanks again.
- Tom Gootee