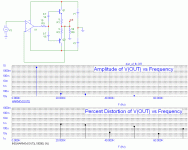
What was the question? Oh, how much negative feedback? I don't know, BUT as little as possible.
john curl said:
Hi John,
You REALLY don't know the amount of NFB in your JC-1????
I'm guessing 20 dB at 20 kHz, with a 4 kHz open-loop bandwidth, resulting in 34 dB at low frequencies, and I'm guessing an essentially dominant pole rolloff. This based on some of the speculation by others. Please correct me if I am wrong.
Bob
john curl said:
john curl said:
Linearizing the VAS might be good, but not all of the measures Mr Curl mentions are certain to actually increase "feedback" as measured by Bode's T, particularly with grounded shunt compensation of the VAS
if however an increase of loop gain is achieved - which by the way allows an increase in bandwidth with higher order compensation - we have several published papers pointing to a decrease in the harmonic distortion components once the loop gain is above a very modest level;
Cherry puts the point at which the seventh order term in the output begins to decrease at 5.5 loop gain around a 2nd order nonlinearity "Estimates of Nonlinear Distortion in Feedback Amplifiers" JAES V48 #4 p299-312 april 2000
the very simple sim below shows feedback reducing the harmonics in output stages with 10% 2nd or 3rd order nonlinearities - and the many harmonics in the output created by feedback around single nonlinear terms
left fft is just the harmonics of the output (1KHz is 0 dB) of the 2nd order nonlinear term looped by gain that steps from 20 dB to 60 dB in 10 dB steps
the right fft plot is the same except the exponent has been changed to 3 in the BV source nonlinear term
the seventh harmonic of the 0 dB, 1 KHz excitation at 7 KHz decreases with each gain increment, starting from a very modest loop gain of 10 (see the gain step, trace color id insets lower right)
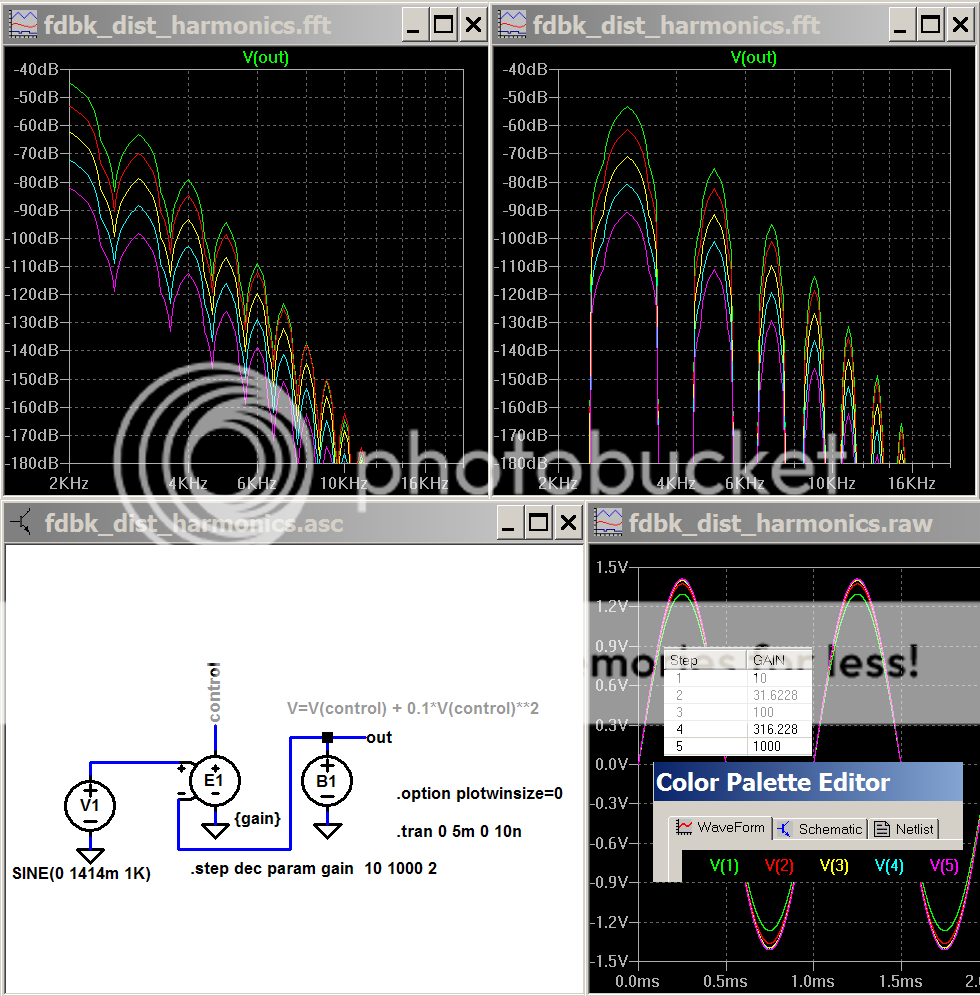
It is possible to observe the decrease of individual higher harmonics in more complicated feedback amplifiers at specific (low) loop gain factors as the nonlinear terms from differing stages may result in specific harmonics with matching levels but opposite phase, the matching conditions on nonlinear terms would appear to be sensitive to hard to control parameters, and it can only occur for a very few (one?) harmonic
As a general distortion reduction principle that applies to all audible harmonics high loop gain seems the far more robust choice, particularly after you've already made the choice to get "a little bit pregnent"
Interesting posts. To put a little more oil on the fire 😉 and to support that Baxandall graph posted earlier (change of harmonic structure with nfb factor), I have scanned a 1978 WW article and put it on my home page.
"Negative feedback and non-linearity - Exploring the fallacy that nfb reduces all harmonics equally - Cathode Ray, Wireless World Oct 1978".
An interesting part in the article addresses the abrubt increase in 7th harmonics under certain conditions, something that John Curl has mentioned several times as one of his "yard sticks".
Jan Didden
"Negative feedback and non-linearity - Exploring the fallacy that nfb reduces all harmonics equally - Cathode Ray, Wireless World Oct 1978".
An interesting part in the article addresses the abrubt increase in 7th harmonics under certain conditions, something that John Curl has mentioned several times as one of his "yard sticks".
Jan Didden
Very good article!
Thank you very much.
So, an weight factor of 2 is enough to approximate audibility of high order harmonics?
Thank you very much.
So, an weight factor of 2 is enough to approximate audibility of high order harmonics?
roender said:
Well, that is one opinion. From the article I gather that the BBC favoured a quadratic ratio: i.e. the 4th harmonic should be weighted 16 times...
That seems excessive to me, though.
Jan Didden
Hope I am not much OT. I have my own disc with 2nd, 3rd, 4rd, 5th H of different levels. Also 2 + 3, ... 2 + 3 + 4 + 5 .... in different ratios and levels. It is more than interesting.
Solid state and valve-like distortion test signals as well.
Starting at 4th, the harmonics are easibly audible and one hears them as separated tones. 2nd, and even 3rd tend to sound like coloration. Depends on levels, off course.
P.S.: contrary to what we learnt, 1% of the 2nd harmonic is well audible.
Solid state and valve-like distortion test signals as well.
Starting at 4th, the harmonics are easibly audible and one hears them as separated tones. 2nd, and even 3rd tend to sound like coloration. Depends on levels, off course.
P.S.: contrary to what we learnt, 1% of the 2nd harmonic is well audible.
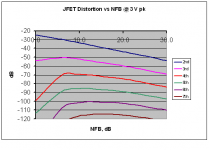
Distortion vs NFB
Hi JCX,
Those results are really nice! Great minds think alike. You beat me to the punch! For the last week or so I've been running simulations to answer the same question. I'll try to post my plots this afternoon, but here is a summary of what I did and found.
I first took the approach of duplicating Baxendall's results for the JFET in simulation. I wanted to duplicate the results to give confidence in the simulation approach. I was not able to find SPICE models for his exact JFET, but the one I used appears to be very similar, and the behavior of the circuit is like that of Baxandall's results.
The second harmonic distortion was down 25 dB from the fundamental (about 6%). Third through 7th harmonics start out very small without NFB, then increase as NFB is applied and increased, then beyond some key value of NFB they begin to decrease. That key value of NFB at which distortion peaks increases with the order of the harmonic. Third peaks at 6 dB NFB, while 7th peaks at 15 dB NFB (to put it in perspective, this seventh "peaks" at -115 dB).
Having verified that Baxandall's results could be duplicated with confidence in simulation, I then asked the question of whether his results were generally applicable. After all, he just did it for a well-behaved square-law device (and just a single stage).
Would it be the same for a BJT with an exponential characteristic, recognizing that the BJT would start with significant distortion products at all harmonics, even without NFB?
Would it be the same for a BJT differential pair? This is very relevant because it is a balanced circuit that is representative of so many complementary amplifier topologies used these days.
So I then did the simulation using a single-ended BJT instead of the JFET. The results were dramatic. There was no enhancement of harmonics as NFB was applied and increased. ALL distortion components decreased monotonically from the get-go as NFB was applied and increased. This really brings into question the relevance of the baxandall results in the general case in the real world.
Baxandall was brilliant, and there is nothing wrong with what he did. It's just too bad he was not able to take it further to more general cases. Of course, this is understandable because the analysis quickly becomes intractable much beyond a second-order nonlinearity.
I next did the simulation using a BJT differential pair. Pretty much the same, except that one can see a little bit of enhancement of the more benign even-order products that were very low to begin with. All odd-order products decreased monotonically as NFB was applied and increased.
The next thing I'm trying is to see what the application of NFB does to the distortion components of an output stage in regard to its crossover distortion. Stay tuned.
Because the Baxanall results are a key underpinning of the concern about NFB expressed by feedback-averse designers, these results cast some new light on the controversy. All that can be said now is that if one builds a single stage, single ended amplifier with a JFET, one needs to take into account the Baxanall results. Anything else is likely to be a different kettle of fish.
Cheers,
Bob
Hi JCX,
Those results are really nice! Great minds think alike. You beat me to the punch! For the last week or so I've been running simulations to answer the same question. I'll try to post my plots this afternoon, but here is a summary of what I did and found.
I first took the approach of duplicating Baxendall's results for the JFET in simulation. I wanted to duplicate the results to give confidence in the simulation approach. I was not able to find SPICE models for his exact JFET, but the one I used appears to be very similar, and the behavior of the circuit is like that of Baxandall's results.
The second harmonic distortion was down 25 dB from the fundamental (about 6%). Third through 7th harmonics start out very small without NFB, then increase as NFB is applied and increased, then beyond some key value of NFB they begin to decrease. That key value of NFB at which distortion peaks increases with the order of the harmonic. Third peaks at 6 dB NFB, while 7th peaks at 15 dB NFB (to put it in perspective, this seventh "peaks" at -115 dB).
Having verified that Baxandall's results could be duplicated with confidence in simulation, I then asked the question of whether his results were generally applicable. After all, he just did it for a well-behaved square-law device (and just a single stage).
Would it be the same for a BJT with an exponential characteristic, recognizing that the BJT would start with significant distortion products at all harmonics, even without NFB?
Would it be the same for a BJT differential pair? This is very relevant because it is a balanced circuit that is representative of so many complementary amplifier topologies used these days.
So I then did the simulation using a single-ended BJT instead of the JFET. The results were dramatic. There was no enhancement of harmonics as NFB was applied and increased. ALL distortion components decreased monotonically from the get-go as NFB was applied and increased. This really brings into question the relevance of the baxandall results in the general case in the real world.
Baxandall was brilliant, and there is nothing wrong with what he did. It's just too bad he was not able to take it further to more general cases. Of course, this is understandable because the analysis quickly becomes intractable much beyond a second-order nonlinearity.
I next did the simulation using a BJT differential pair. Pretty much the same, except that one can see a little bit of enhancement of the more benign even-order products that were very low to begin with. All odd-order products decreased monotonically as NFB was applied and increased.
The next thing I'm trying is to see what the application of NFB does to the distortion components of an output stage in regard to its crossover distortion. Stay tuned.
Because the Baxanall results are a key underpinning of the concern about NFB expressed by feedback-averse designers, these results cast some new light on the controversy. All that can be said now is that if one builds a single stage, single ended amplifier with a JFET, one needs to take into account the Baxanall results. Anything else is likely to be a different kettle of fish.
Cheers,
Bob
No, he did this also for BJT in the last paper of the series, Feb next year
Please check your simulution as Baxandall _measured_ results for BJT exhibit enhancement of harmonics as NFB was applied
Further reading:
www.its.caltech.edu/~musiclab/ feedback-paper-acrobat.pdf
Re: Distortion vs NFB
Hello Bob,
Fascinating! You may want to review an article by M Scroggie ("exploring the fallacy that nfb reduces all harmonics equally") who looked at the same issue as you did but from a different (numerical) perspective. Unfortunately, he did mention the two cases of a quadratic transfer curve and a log one, but only worked the quadratic one...
Jan Didden
Bob Cordell said:
Hello Bob,
Fascinating! You may want to review an article by M Scroggie ("exploring the fallacy that nfb reduces all harmonics equally") who looked at the same issue as you did but from a different (numerical) perspective. Unfortunately, he did mention the two cases of a quadratic transfer curve and a log one, but only worked the quadratic one...
Jan Didden
