Mathematical theory tends to have a much longer half-life than technology. That's part of the reason theory is taught in college, most of it is expected to be of value for a very long time, and often it will be applicable to changing technologies.
True... but 'use it or loose it' still applies.
I still have all my math books from college... algebra, trig thru calc. Cant rely only on memory for some seldom used math.
Now we can buy math software to do most calc for us in any field. And SIM software which uses a lot of math under the skin. Math programmers have saved the day of tedious calculations.
yes, Dadod.... knowledge optimization.
-RM
Last edited:
Its good to review some things once in a while.
Or, how about finding somebody to mentor? Some people find nothing makes one learn an academic subject better than having to teach it (at least if one wants to be able to answer any student questions fully and accurately).
Or, how about finding somebody to mentor? Some people find nothing makes one learn an academic subject better than having to teach it (at least if one wants to be able to answer any student questions fully and accurately).
Last edited:
And, mentoring is really great way to speed things along for the one being mentored. Right now at my stage in life, I can wake up dead tomorrow. I have my student coming from Nepal, she needs a lot of mentoring in many areas. It will be fun to explore the new world with her. Show her and give her new experiences to enrich her life for years to come.
-Richard
-Richard
The other thing about remembering seldom used math, is to start by refreshing memory of notation.
I have bought a fair amount of books and plan to buy more. Even though everything is freely available (illegally). It´s how we support knowledge.
Despite academia being riddled with cultural marxism (a bit less for STEM), I still believe in this form of education. I believe in its recovery.
I also strongly believe in other forms like self-teaching.
Despite academia being riddled with cultural marxism (a bit less for STEM), I still believe in this form of education. I believe in its recovery.
I also strongly believe in other forms like self-teaching.
Last edited:
Thankfully!
But academia is still needed to produce the knowledge and the books.
No matter how much time you spend in school....BS, MS, PHd. It is little read and learned compared to the knowledge gained during the rest of your life. Compare 4-8-10 years in college/university to additional continuous study for 50 years+ Just when you really have a wide range of knowledge, you retire or die. Or both. All that knowledge and experience gone... and you did not write a single book yourself.....its just lost knowledge and experience to the world
Unfortunately, unlike pure mathematics, most of the technical details you learned was long ago obsolete and now useless knowledge taking up space in your brains' memory. But still, one can read an awful lot of books and testing in a life time.
THx-RNMarsh
Unfortunately, unlike pure mathematics, most of the technical details you learned was long ago obsolete and now useless knowledge taking up space in your brains' memory. But still, one can read an awful lot of books and testing in a life time.
THx-RNMarsh
Last edited:
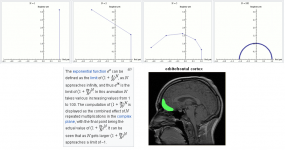
Stanford mathematician, Keith Devlin wrote - "The most beautiful equation"
e (raised to the i*pi) +1 = 0
Why is Euler's formula so breath-taking? And what does it even mean?
Thx-RNMarsh
'
e (raised to the i*pi) +1 = 0
Why is Euler's formula so breath-taking? And what does it even mean?
Thx-RNMarsh
'
Last edited:
Richard Feynman called the equation "our jewel" and "the most remarkable formula in mathematics". Euler's formula - Wikipedia
I was told the same by my maths teacher when I was 16 but have not found a mathematician who can coherently explain why its so wonderful!
It’s a matter of what stimulus your orbitofrontal cortex is most sensitive to.
🙂
Euler's identity - Wikipedia
George
Attachments
It puts together, in one formula, calculus (the e number), geometry (the pi number), algebra (the i number) and arithmetic (the 1 number). Hence, this formula illustrates the unity of mathematics.
See I view that as con to make it sound good. Lots of equations have a 1 in them. Doesn't make them magically beautiful! I would put Maxwell's equations, despite their chewyness much higher on the list.
Clearly my orbitofrontal cortex is bust 🙂
Clearly my orbitofrontal cortex is bust 🙂
In my book, Maxwell equations are not pure mathematics, but applied mathematics, here for modelling the EM field. It's rather easy to visualize gradient, divergence, curl (called rotor here) operators, inner products, line and surface integrals, potentials, etc... and the solutions are, for the most part, rather intuitive.
Euler equation is pure mathematics, it's as abstract as you can get, I don't see a way to visualize it.
Last edited:
Maths for the sake of maths I don't have brain space for. Enough of the useful stuff keeps falling out. Anyway, Euler's formula is used pretty much anywhere you are dealing with a sinusoidal function, so very useful. His identity is just the brain equivalent of a delicious amuse-bouche so is either considered wondeful or 'meh'.
- Status
- Not open for further replies.