I don’t consider an OB a betetr enclosure, just an indicator that a lot of peopel build poor boxes. I couldn’t live with whatever every OB i have heard does wrong.
dave
dave
"IME, neither. I don't use MDF, because I hate working with it. I don't use visco-elastic damping because I've not found it worth the bother. I do use plywood, very well braced. I'd use visco-elastic and MDF if I found either worked better, but I've tried both and found them not to work any better than well braced 1/2 inch plywood. I've gone so far as to build similar designs using as thin as 1/8" plywood versus 3/4" MDF and found response to measure the same. The only major difference in the design stage is that to use thinner than 3/8" plywood the panels must be bent.
As for 'looking at the architectural parallels', I have plenty of experience as a general contractor to bring into play, but found that the best experience I had to prepare me for designing speakers was building balsa model airplanes as a kid. Just like speakers aircraft require very high strength, and they must do so with light weight. So I don't build speakers like I would a house, I build them like an airplane or boat. One builder of my designs has noted that they closely resemble what he designs for a living, at Boeing." _BillFitz
I found this comment while searching for info regarding my current build.
As for 'looking at the architectural parallels', I have plenty of experience as a general contractor to bring into play, but found that the best experience I had to prepare me for designing speakers was building balsa model airplanes as a kid. Just like speakers aircraft require very high strength, and they must do so with light weight. So I don't build speakers like I would a house, I build them like an airplane or boat. One builder of my designs has noted that they closely resemble what he designs for a living, at Boeing." _BillFitz
I found this comment while searching for info regarding my current build.
Interesting discussion....I will say from my many hours of modelling my perspective on resonance is that the higher the resonance the smaller the resonance...lower resonances have more energy, concluded by the impedance peak. Higher frequency energy is more efficient, so possibly the the lowered impedance peak as we move the resonance up, is a reflection of this, and lets say that without dampening, the resonance is equally perceived on the loudness scale...dampening material is going to make short work of the higher resonances...while the lower ones are harder to dampen away...how about that?
Performing music requires a SOUL.
Today you can buy a SOUL for ~$1,500 per speaker.
For about 10 years, Quad electrostatic dipole speakers were used in the SF Opera rehearsal rooms. I still remember hearing Kathleen Battle performances in SF and Berkeley with my wife ... a gift from God.
---------
Spend your money to listen to live performances.
Research how to bring some of that magic into your listening room.
---------
Dr. Geddes has given us hope that new controlled directivity horn speakers can mimic some of the old large room sound stage magic in our new smaller homes. Today, you can buy a SOUL for $1500/speaker! ($600 DCX464 coaxial driver + $210 Faital 12FH520 midrange + two sealed side-side, counter-force, low-vibration $170 15" Dayton RSS390HO-4 woofers)
-------
VIVID research proved counter-force woofer construction nulls woofer vibration
_____
B&W research proved matrix bracing dramatically reduces random cabinet deep+mid bass vibratrion
______
B&W tapered rear transmission lines reduce resonances with limited stuffing. Proven again...and again over midbass frequencies
______
Spend money to listen to live performances.
Research how to bring some of that magic into your listening room.
Today you can buy a SOUL for under $1,500 per speaker
Today you can buy a SOUL for ~$1,500 per speaker.
For about 10 years, Quad electrostatic dipole speakers were used in the SF Opera rehearsal rooms. I still remember hearing Kathleen Battle performances in SF and Berkeley with my wife ... a gift from God.
---------
Spend your money to listen to live performances.
Research how to bring some of that magic into your listening room.
---------
Dr. Geddes has given us hope that new controlled directivity horn speakers can mimic some of the old large room sound stage magic in our new smaller homes. Today, you can buy a SOUL for $1500/speaker! ($600 DCX464 coaxial driver + $210 Faital 12FH520 midrange + two sealed side-side, counter-force, low-vibration $170 15" Dayton RSS390HO-4 woofers)
-------
VIVID research proved counter-force woofer construction nulls woofer vibration
_____
B&W research proved matrix bracing dramatically reduces random cabinet deep+mid bass vibratrion
______
B&W tapered rear transmission lines reduce resonances with limited stuffing. Proven again...and again over midbass frequencies
______
Spend money to listen to live performances.
Research how to bring some of that magic into your listening room.
Today you can buy a SOUL for under $1,500 per speaker
It is an opinion. Somebody else might say there is no hifi without horn. People like different things 🙂
I hope this thread doesn't end up being a fight about opinions and views how things should be done. OP has some engineering background, it would be cool to data instead of opinions here 🙂
I hope this thread doesn't end up being a fight about opinions and views how things should be done. OP has some engineering background, it would be cool to data instead of opinions here 🙂
Last edited:
Took my eye of this and things have run away without me 😀
The nautilus shape interests me, I think I can make this shape and if its ideal then thats the shape I'll be choosing for the mid enclosure most likely. But why isn't a common shape to adopt if its such a good shape?
If you wanted strength in all directions why not choose a more homogeneous material? The reason you want to use a material with directional strength is if you want to optimise the stiffness for a certain direction. My guess would be there isn't much lateral stiffness required in a speaker as the pressure waves move back and forth but it would be useful for a more experienced mind to advise me on that.
I work in a more special purpose design area so its a little easier, we're not a production line, people come to us if they need a specialist inspection or process if that makes sense. The machinists would help me out no problem but the additive manufacturing unit is a little different as you might imagine.
But if you think about it potential energy in the system is entirely to do with the stiffness of the cabinet. So stiffness determines them maximum amplitude vibration for a given energy input. Damping determines how quickly that energy dissipates. So I suppose the question is are higher frequencies more audible?
So gentle curves? I suppose such as the nautilus since I keep bringing it up.
But if you had a low resonant frequency it would still resonate in the higher frequency ranges due to the harmonics. So you make a floppy cabinet in order to push the resonant frequencies down to the lower range but it would still vibrate at its harmonics in the higher frequencies. Also are we really THAT much less sensitive in the lower frequency ranges? An accurately produced bass line is one of my most desired features of a speaker, most speakers dont do it well and I love the bass as an instrument.
I'll have to read the rest later
The nautilus shape interests me, I think I can make this shape and if its ideal then thats the shape I'll be choosing for the mid enclosure most likely. But why isn't a common shape to adopt if its such a good shape?
If you wanted strength in all directions why not choose a more homogeneous material? The reason you want to use a material with directional strength is if you want to optimise the stiffness for a certain direction. My guess would be there isn't much lateral stiffness required in a speaker as the pressure waves move back and forth but it would be useful for a more experienced mind to advise me on that.
I work in a more special purpose design area so its a little easier, we're not a production line, people come to us if they need a specialist inspection or process if that makes sense. The machinists would help me out no problem but the additive manufacturing unit is a little different as you might imagine.
Sorry yeah that's a very simple solution, I dont know why I didnt think of that aha
I've been trying to think about this one alot, not sure why I cant get my head around it, I tried solving the equations of motion for energy but I've not had to do dynamics in so long I've actually just forgotten how to do maths!
But if you think about it potential energy in the system is entirely to do with the stiffness of the cabinet. So stiffness determines them maximum amplitude vibration for a given energy input. Damping determines how quickly that energy dissipates. So I suppose the question is are higher frequencies more audible?
So are you arguing that a square cabinet is fine and all these fancy shapes such as the nautilus is just marketing? Although personally see no harm in striving for an ideal in the design, especially for a DIY project where time is less of a factor.
So gentle curves? I suppose such as the nautilus since I keep bringing it up.
But if you had a low resonant frequency it would still resonate in the higher frequency ranges due to the harmonics. So you make a floppy cabinet in order to push the resonant frequencies down to the lower range but it would still vibrate at its harmonics in the higher frequencies. Also are we really THAT much less sensitive in the lower frequency ranges? An accurately produced bass line is one of my most desired features of a speaker, most speakers dont do it well and I love the bass as an instrument.
I'll have to read the rest later
when the original wave loses its shape ( it gets squished & squashed ) trough the horn, it's not anymore similar, so that's not hi-fi-
Opinions?
when the original wave loses its shape ( it gets squished & squashed ) trough the horn, it's not anymore similar, so that's not hi-fi-
Opinions?
That’s a shot more so at compression drivers not horns in general...with that mindset any vented enclosure that had a terminus CSA smaller than the woofer fails your qualifications for hifi as well. If I understand you correctly, the speaker would have to take on the shape of the reproduced source to follow this path. This makes me think of some interesting concepts...concave diaphragms compress the signal in comparison to a dome diaphragm...hmmm
Last edited:
If what it takes for the wave to traverse the box is short compared to the wave itself, pressure builds up in the box.
Increasing stiffness reduces movement only if there are no resonances present. That is, we are in what an engineer would call the stiffness controlled region and not the mass controlled region at very high frequencies or the inbetween region containing largely discrete resonances. At a resonance the force due to stiffness is wholly cancelled by the inertia force leaving only the damping force to oppose movement. The ratio of stiffness and mass determine the frequency but not the magnitude.
The goal is not no movement (not viable) but movement that is small enough to be inaudible in the presence of sound from the driver.
Because it is a large awkward shape that performs no better in practice than simpler more convenient shapes when stuffing is present. The companies that use such things are almost certainly doing so primarily to have a marketing feature to positively distinguish their products in the eyes of potential customers. This is a perfectly good solid reason but it isn't to do with engineering.
I have been working off-and-on on a desktop transmission line design for the desk in my study. My motivation is not because I think it is the optimum engineering solution but because it is a challenge and if I can get it to work well it should match a more rational design albeit in a larger more expensive package. I am also motivated by fond(ish) memories of a small TL speaker I built in the 70s with a small woofer. It was SPL limited for a room but would likely have been sufficient for a desktop. Now regret chucking them in a skip when pressed for time and space in a house move.
Yes they are as shown by the audibility threshold in Figure 12 here. I seem to recall a few years ago seeing results from a similar study by a different group which came up with a slightly different threshold but cannot recall where. Anyone?
Yes but those higher order mode shapes require greater deformation of the structure compared to the lower order mode shapes (they are more "wiggly") and this deforms the damping material more creating more damping. This increased damping at the higher frequencies where the ear is more sensitive is the motivation for the approach although this isn't always appreciated by those that promote it.
Low frequency sound quality in the home is usually more to do with room control/treatment and less to do with the details of speaker design assuming adequate clean SPL. Again there will no doubt be some disagreement between an engineer's view based on engineering evidence and many speaker enthusiasts views based on more casual evidence. It's not just cabinet design.
It is splitting hairs to label an ideal discussion as unrealistic. We probably don't need to discuss how resonance works, but rather what to do with it.
Andy - on this point I tend to agree with you, although I am open to the possibility that these hard-to-make shapes could have some small effect when used on the very highest performance speaker systems. But if there is an effect, it does not seem measurable with current measurement techniques. If there is an effect, I have not been able to hear it... i.e. I have heard great sounding speakers that both use and not use this technique. Some of the finest speakers I have ever heard have been shaped like a box.
Every loudspeaker has many compromises. The set of compromises tha best suits a particular listener’s needs are the best speakers for that person.
This is one of the reasons we see so many different kinds of loudspeakers that people are happy with.
dave
How about sand filled baffle like Wharfedale? I wonder sand filled between double wall speaker box would be nonsense or not.
Wharfedale SFB/3 | Inner Magazines
Wharfedale SFB/3 | Inner Magazines
So here's the question I have for those who know more than I do. I've gone as far as finding the right equations to calculate the theoretical natural panel resonances and their stiffness factors but I can't figure out the relationship between energy and resonance amplitude.
So say you have 3 examples of the same material in different panel sizes and with different thicknesses but with the same resonant frequencies. Using the engineering formula for panel resonances for a panel with 4 edges simply supported, here's what I mean:
Case 1: a 4" x 6" x 1/2" thick panel with resonant frequency = 1400Hz and stiffness (K) = 1923 lb/sq in
Case 2: a 5" x 14" x 1" thick panel with resonant frequency = 1400Hz and stiffness (K) = 4093 lb/sq in
Case 3: a 6" x 21" x 1.5" thick panel with resonant frequency = 1400Hz and stiffness (K) = 6284 lb/sq in
So my question is, given the obviously different mass and stiffness of each panel but the same approximate resonant frequency, if each panel receives the same amount of energy, will the resonances produced occur at the same or different SPL levels? Or to put it another way, will case 3, the panel with higher stiffness and mass, require more energy input for it to ring as loudly as case 1 and 2?
So say you have 3 examples of the same material in different panel sizes and with different thicknesses but with the same resonant frequencies. Using the engineering formula for panel resonances for a panel with 4 edges simply supported, here's what I mean:
Case 1: a 4" x 6" x 1/2" thick panel with resonant frequency = 1400Hz and stiffness (K) = 1923 lb/sq in
Case 2: a 5" x 14" x 1" thick panel with resonant frequency = 1400Hz and stiffness (K) = 4093 lb/sq in
Case 3: a 6" x 21" x 1.5" thick panel with resonant frequency = 1400Hz and stiffness (K) = 6284 lb/sq in
So my question is, given the obviously different mass and stiffness of each panel but the same approximate resonant frequency, if each panel receives the same amount of energy, will the resonances produced occur at the same or different SPL levels? Or to put it another way, will case 3, the panel with higher stiffness and mass, require more energy input for it to ring as loudly as case 1 and 2?
Dave,
I take this as applicable in the midrange. In this context, what does "sustained" mean? How many cycles can a note be sustained without exciting a resonance? A 1kHz tone cycles 100 times in a tenth of a second, would that not be enough to excite a resonance?
How far off of the midrange resonance peak must the signal be to completely avoid excitation? In a bass reflex cabinet the port response is not "on" at a single frequency and otherwise "off", it might be down 10 dB an octave above or below relative to the resonant peak. I would expect cabinet resonances to be have similarly. Does a bell only ring if I tap it at its resonant frequency?
My own, probably imperfect understanding of the problem as a whole is that when the enclosure is 'small' compared to the wavelengths radiated (e.g. bass cabinets), stiffness is the most important factor. When the enclosure must be physically large enough for panels to resonate at the radiated frequencies, some level of damping will be desired to reduce sound radiation from the cabinet walls. When the wavelengths are large enough compared to the size of the baffle, the influence of cabinet geometry on the polar pattern becomes an important concern. Balancing all of that in a single box is difficult. From that standpoint, separating the mids and highs from the bass has some advantages.
As for internal standing waves, IIRC Dr. Geddes noted that internal geometry can only change the resonant frequencies, but it cannot eliminate them. Irregular or tapered cabinet volumes will still have standing waves (though the shapes might be harder to predict or visualize), which are best dealt with by including some internal damping material.
Bill
How many cycles depends a lot on the material & the Q. How likely are you to see a sustained 100 cycles at 1K, or 500K, or higher, in music.
The energy needed to excite a resonance has to fall within the envelope of the (potential) resonance. A hi Q resonance has a narrower bandwidth so will only be affected by a narrower band of frequencies.
Damp a panel and the Q lowers and the bandwidth increases making for more potential energy to be loaed into it.
dave
While i was looking for the picture (which i did not find) i found some other useful stuff;
post &
Discussion on what materials to build speakers out of
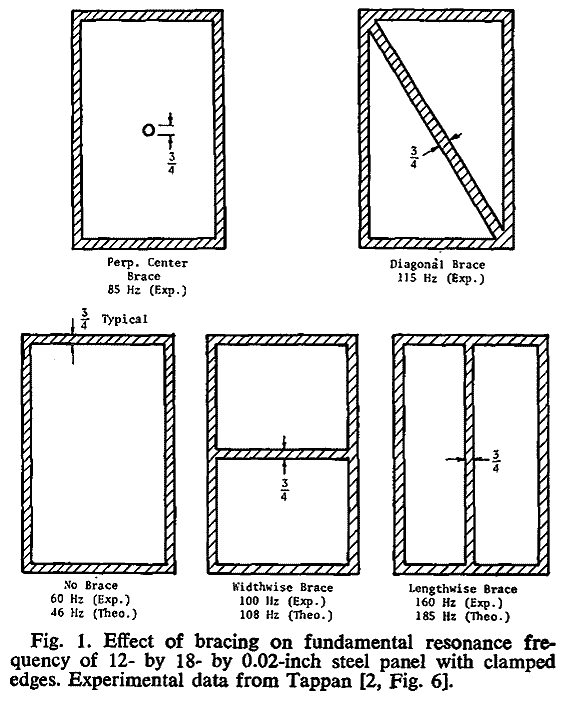
Tappan:
Ceramic enclosures: Ceramic speaker enclosures
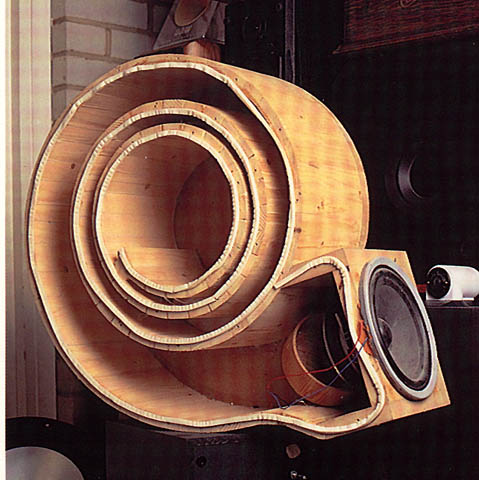
One of the B&W NAutilus prototypes:
The energy needed to excite a resonance has to fall within the envelope of the (potential) resonance. A hi Q resonance has a narrower bandwidth so will only be affected by a narrower band of frequencies.
Damp a panel and the Q lowers and the bandwidth increases making for more potential energy to be loaed into it.
dave
While i was looking for the picture (which i did not find) i found some other useful stuff;
post &
Discussion on what materials to build speakers out of
Tappan:
Ceramic enclosures: Ceramic speaker enclosures
One of the B&W NAutilus prototypes:
Indeed, also what are the effects of the radiating surface being different in size.