Since I started building sealed boxes equalized using the Linkwitz transform ( a 2nd order asymmetric shelf filter ) I can have any F3 and Q that I desire and switch between them with the miniDSP remote control. I find the bass sounds really great when I EQ it to something like an F3 of 20 Hz and Q = 0.5 or lower. Of course before you do that you need to select woofers that have the displacement ( Xmax * Sd ) sufficient to produce the SPL you plan to listen to. As most music has zero content below 30 Hz this works perfectly and it produces very high quality bass without any boom. With this setup the box tuning is canceled out and hardly matters. WinISD actually includes the Linkwitz filter so it is very easy to model a system before building it to see exactly how it works. Look at the max SPL and group delay plots while you're in there.
A white pair of servosounds were the first pair of speakers I considered buying in the early 70s. They were unreliable and a new pair was going cheap in a local hi-fi shop because one channel had failed before they could get them out the door. I was impressed by how small and loud the functioning channel was by the standards of the time. Common sense was imposed on me and I built some DIY speakers instead.
PS Servosound was Belgian and their approach was a bit cruder than the one Philips later pursued. Some discussion here.
You are missing the point and wasting your time solving something that is not a problem.
Choose a solution and put it to test. Then decide if it's worth keeping.
There have been many good answers in there.
Time to choose a driver ( i sugest a cheap car audio sub optimised for medium/small volume box), make some sawdust and implement a Linkwitz Transform ( eq plug in or software on computer) and make your own pov as Lojsek suggested.
If possible make it easily movable and try it in different acoustic environnement.
Time to choose a driver ( i sugest a cheap car audio sub optimised for medium/small volume box), make some sawdust and implement a Linkwitz Transform ( eq plug in or software on computer) and make your own pov as Lojsek suggested.
If possible make it easily movable and try it in different acoustic environnement.
Step response isn't wildly different between Qtc=0.5 and 0.7 All sealed step responses have some overshoot. Who cares if your box rings for a partial cycle at 30 Hz when your room rings for seconds at MULTIPLE frequencies, All these critically damped nuts are going after is an engineering goal or a goal that they have been told is optimmum and then convincing themselves that it sounds right. If you look at John k's presentation, you can see that lower Q boxes don't necessarily follow tonebursts better, so there is nothing there that is saying anything about steady state.
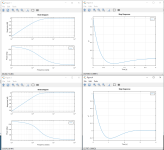
Below are bode plots and step response plots for 0.5 (top) and 0.7 (bottom) note different time scale on the 0.7 step response..
Step overshoot is 0.14 for the "critically damped" box, and 0.2 for the maximally flat box.
Attachments
There is theory, then real world.
So it is a balance of hearing what it really does
compared to assumptions looking at graphs.
Having a thing for live sound paper cone 15" speakers in my younger years.
Going all out and building a large .5 cabinet the first time over the weekend.
Heard the same drivers in many styles of smaller boxes.
Also at mild to wild SPL levels.
Starred at many sims of that driver as well, and built many of them.
Finally get to really connect and understand a lot of assumptions
on the computer screen. To What it really did and does in real life.
Many assumptions were wrong. Then now know what it " Looks"
like on a screen, to what it actually sounds like.
EQ changes song to song and there is nothing weak about 15" speakers.
Specially in some real big boy cabinets.
Excessive EQ in a small box has more turn downs more than anything.
So it is a balance of hearing what it really does
compared to assumptions looking at graphs.
Having a thing for live sound paper cone 15" speakers in my younger years.
Going all out and building a large .5 cabinet the first time over the weekend.
Heard the same drivers in many styles of smaller boxes.
Also at mild to wild SPL levels.
Starred at many sims of that driver as well, and built many of them.
Finally get to really connect and understand a lot of assumptions
on the computer screen. To What it really did and does in real life.
Many assumptions were wrong. Then now know what it " Looks"
like on a screen, to what it actually sounds like.
EQ changes song to song and there is nothing weak about 15" speakers.
Specially in some real big boy cabinets.
Excessive EQ in a small box has more turn downs more than anything.
Then you should be able to recall what some of them are, care to share? I don't know how theoretical it is to say a system is critically, over or under damped. There is large 0.5 cabinet. Q is the result of a system of things, cabinet size is another variable in the formula resulting in the final Q. There are other benefits to a large cabinet that Effect SQ that are separate from Q
Step overshoot below the 0 line is a feature of all of these 2nd order highpass pressure response functions. It even occurs for a Q of 0.1 You are talking about the rebound after the overshoot.
If you want to talk about why Q=0.5 gets its name, it has to do with another type of response function that doesn't correlate with anything we hear 😉 Can you guess what function that is?
I am not saying that anyone who strives for Q=0.5 is foolish, to each their own, there is just a lot of misinformation out there in the audiophile press that lauds it as something that it is not. It is one of your options, weigh the pros and cons. Is the increased box size and reduced power handling worth the tiny incremental improvement in transient response? Not in my book.
Low Qtc (e.g. 0.5) maybe worth if one wants to boost the low-end, because it don't requires as much Wattage or room-gain to get a flat response with it to the deepest frequencies.
But then maybe a vented box is a better option for that.
But then maybe a vented box is a better option for that.
In my anecdotal experience, the psychoacoustic problem presented by critically damped bass systems is that it tends to cause bass energy to audibly start rolling off too early. To prevent that, the bass system needs to remain flat to a lower frequency before it starts a low Q roll off. That places a double demand on increasing woofer volume, because while a sealed box enclosure (for illustration) needs a greater volume to provide a lower Q, it additionally requires even greater volume than that to maintain both bass flatness AND provide critical damping.
I am not talking about that because it has to be always present since it is not possible to apply static pressure to a usual listening space by the use of a speaker. Also both areas below and above zero do have to be equal.
What I meant is happening at about 2.8 ms where the higher Q case crosses the zero line again while the one for the lower Q case nears the zero line asymptotically.
But as I said the difference is minumal.
One advantage of an aperiodically tuned box is the fact that the EQ circuits for the active tunig of the cutoff frequency are the simplesrt possible: Shelving filters.
Regards
Charles
what should not be forgotten is that for good transient response the ideal loudspeaker should have a mellow surround and an infinite stiff cone. Besides travel linearity of the motor.
There was another whole thread only about Q factor ignoring the other parameters.
There was another whole thread only about Q factor ignoring the other parameters.
I already addressed that in my comment #30 by talking about rebound. You didn't answer my question though. There is a response function for which the Q=0.5 speaker is critically damped. Few people are likely to know it.
So what order are aperiodic boxes? 😉 ...and how would you prove it?
What was the low-frequency cut-off frequency of these low-Q subwoofers? If the original recording was dry, would it need to be embellished (by bloom or ringing) to make it more palatable for a listener? That somehow seems counter to high-fidelity sound reproduction.
Electro-dance music relies on large quantities of low-frequency bass. Unfortunately, when considering many low-Q low-frequency (sealed) enclosure designs, their intrinsic premature low-frequency roll-off directly encroaches on the quantity of bass that can be produced. It seems that, in broad terms, it is the lowering of bass levels that is what makes such designs less successful for electro-dance music applications, and not the fact that it is "low-Q".
There was an interesting analysis of room gain in Audio Xpress, "Simulation Techniques: Room Gain", written by René Christensen.
I'm not sure why step response will matter so much. I fully recognize that it can be used as a means of assessing the low-frequency transient response of a dynamic system, but it is quite an unusual signal. For loudspeakers, which generally have zero response at DC, it seems to be a somewhat poor choice of test signal. Maybe because it's so easy to apply it has sometimes been used, but I doubt that it would appear in music all that often.
Would not a tone-burst provide more information? Or even a shaped pulse-like test signal at an appropriate test frequency?
Keep in mind that the measurement of the low-frequency response allows us to infer the behavior of the step response, so why even bother with using a step?
I am not so sure about the room effect. After all, there is a noticeable difference in the response function of these two systems. In the absence of the room, the system with Q=0.7071 will be −3dB at fc, its resonance frequency, but the one with Q=0.50 will be −6dB at fc.
If we input a half-sine pulse or a short tone-burst with main frequency equal to fc, then the Q=0.50 system will likely sound "tighter" because its bass output has been reduced by 3dB. That will be the dominant effect. If we amplify the output of the Q=0.50 system by 3dB (i.e., turn up the gain), then it will be very difficult to discern any difference between the Q=0.50 and the Q=0.7071 system.
Looking at the frequency response differences between a Q=0.50 and Q=0.7071 system, that is an entirely reasonable conclusion to have reached. It is borne out by the measurements.
Would you be able to create a simulation of a 1/2-cycle sine-wave pulse whose frequency is placed at the resonance frequency of the high-pass filter? The results might be insightful.