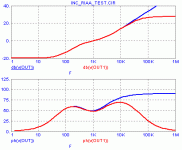
SPICE transfer function for RIAA testing

[Onvinyl]

spice transfer function for riaa testing

Hi,
recently, someone posted a 'voltage source' that simulates an inverse riaa for the spice simulation software. Unfortunately, I can't find it anymore. Anyone remembers where it is?
Rüdiger

 

[burbeck]

try this link

http://waltjung.org/PDFs/A_High_Accuracy_Inverse_RIAA_Network.pdf

or http://www.hagtech.com/pdf/riaa.pdf

regards
bob

 

[Ghianni]

Hi all,

The attachment is a theoritical inverse riaa for simulation taking into account that the 1kHz gain is about 40 dB. I copied it from AudioXpress magazine by Gary Galo.

Ghianni.

 

[jackinnj]

if your SPICE machine allows it the most acurate method is to use Laplace transform -- search under LAPLACE on the DIYAUDIO forum and you can cut and paste the equations into a component model.

 

[Onvinyl]

I use LTSpice, but, being a novice with spice, I have not found out how to incorporate a laplace transform.
I have, however, found the corresponding formula that I searched:

http://www.diyaudio.com/forums/showthread.php?postid=849619#post849619
thanks,
Rüdiger

 

[jackinnj]

Marshall Leach has an article using SPICE and the LAPLACE transform -- the article deals with horn loudspeakers but from it you should be able to glean how to incorporate the LAPLACE function.

http://users.ece.gatech.edu/~mleach/papers/hornmod.pdf

 

[Pjotr]

Hi,

I am not familiar with LT-Spice (I am using Microcap 7) but the generic PSpice syntax is:

LAPLACE:

E|G{name} {+node} {-node} LAPLACE {expression} {s expression}

Example:

E_LOPASS 4 0 LAPLACE {V(1)} {10 / (s/6800 + 1)}

So if your Pspice version does not provide a dependant Laplace source as a schematic entry you can make a sub circuit yourself for the Laplace expression and put it in your library.

A good link for Pspice syntax:

http://www.ecircuitcenter.com/SPICEsummary.htm at http://www.ecircuitcenter.com

Cheers 😉

 

[ashok]

...........theoritical inverse riaa...........

Or just RIAA .

BeigeBag Software A/D V4.x and higher can do this.
The equations can be included in the voltage source or at the output of the circuit . Eliminates any errors that real world components can cause.

Look for "resources " at www.beigebag.com
There is an RIAA example with all details and examples that yoiu can run.
You can also download a free time limited version of A/D V4.x and
run the simulations yourself. Very easy after the first try.

Cheers,
Ashok.

 

[Onvinyl]

Hi,
I'm lost. The formula as spice command:
E_RIAA In Out LAPLACE {V(Out)}={(1+0.00318*S)*((1+0.000075*S)/(1+0.000318*S))}
does something, but it's wrong and I don't know what happens.
If someone could confirm whether the formula in itself is senseless or should work at all?
The nodes 'in' and 'out' are just that...
Rüdiger

 

[Onvinyl]

Hi,
that was deadly wrong, after reading w.jung again I would suppose
E_RIAA out 0 LAPLACE {1117*V(in)}={(1+0.00318*S)*((1+0.000075*S)/(1+0.000318*S))}
to work, but it doesn't!
attached is the circuit and the plot,
Rüdiger

 

[Onvinyl]

...the plot

edit: bad pic, well, the range is -30dB at 1Hz till +77 dB at 20kHz. It rather should be at 0dB
Rüdiger

 

[ashok]

Did you check the "resources tab" at the Beige Bag web site ?
www.beigebag.com

The formula is given in that article about the RIAA correction.
Application might be slightly different in that GUI but you get the idea.

 

[Onvinyl]

Hi,
The formula itself seems to be ok, my problem is the actual implementation. I'm sure, the beigebag-spice software is good, but I shall get it working with ltspice,
Rüdiger

 

[Pjotr]

Hi Rudiger,

In plain pspice text as a sub circuit this works for me:

.SUBCKT I_RIAA IN OUT PARAMS: tau_1=3180u tau_2=318u tau_3=75u
+tau_4=3.18u
*
E1 OUT 0 LAPLACE {V(IN)}={(1+tau_1*S)*(1+tau_3*S)
+/((1+tau_2*S)*(1+tau_4*S))}
R1 OUT 0 1meg
*
.ENDS

For a normal invers RIAA make tau_4=0 and for a corrected RIAA incorporating the 3.16 us time constant, make tau_4=3.18u

Maybe that works in your pspice?

Cheers 😉

 

[Pjotr]

E_RIAA out 0 LAPLACE {1117*V(in)}={(1+0.00318*S)*((1+0.000075*S)/(1+0.000318*S))}

The plus for extending the expression on the next line is missing. It must be:

E_RIAA out 0 LAPLACE +{1117*V(in)}={(1+0.00318*S)*(1+0.000075*S)/(1+0.000318*S)}

Btw. Why that factor "1117"?

😉

 

[Onvinyl]

The plus for extending the expression on the next line is missing. It must be:

E_RIAA out 0 LAPLACE +{1117*V(in)}={(1+0.00318*S)*((1+0.000075*S)/(1+0.000318*S))}

😉

Pjotr,
there is no next line. however, the expression computes without error warning, but shows strange behaviour. This is not related to the gain modifier (1117*)
I give up for today,
Rüdiger

 

[Pjotr]

This is what I get with the sub circuit mentioned.

😉

 

[jackinnj]

In beigebag and multisim there is a transfer function utility -- just multiply and apply the constants:

 

[Pjotr]

Hi,

That sub circuit should work in any standard pspice compliant simulator. Since we are most time interested in the gain at 1 kHz I have modified the model so it gives 0 dB gain at 1 kHz:

.SUBCKT I_RIAA IN OUT PARAMS: tau_1=3180u tau_2=318u
+tau_3=75u tau_4=3.18u CORR=9.897
E1 OUT 0 LAPLACE {V(IN)}={(1+tau_1*S)*(1+tau_3*S)
+/((1+tau_2*S)*(1+tau_4*S)*CORR)}
R1 OUT 0 1meg
.ENDS

Cheers 😉

 

[nuvistor]

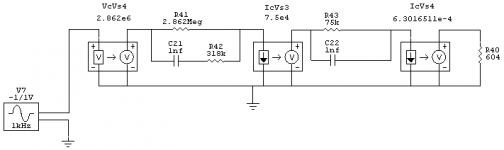
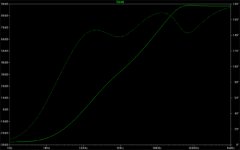
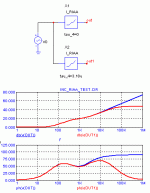
Here's an LTSpice example of Laplace for inverse RIAA. This inverse RIAA includes the "Neumann" 3.18us or 50kHz lead in addition to the usual 50/2122Hz lags and 500Hz lead. The laplace works well in LTSpice only for AC analysis, for transient analysis you should use an RC network. Of course you need the RC network or a test record for frequency response verification if you built something.

The example uses the "El Cheapo" feedback network with an ideal 1-pole opamp, results are similar to OPA637 model.

I have built and tested frequency response of both tube and solidstate phono preamps modeled in LTSpice, agreement between simulation and measurement from 100Hz and 10kHz normalized at 1kHz is usually within 0.2dB, 0.7 to 1dB from 20Hz to 20kHz. I doubt it is possible to verify frequency response this closely with a test record and phono cartridge, if you have done this please let me know.

The E source with the Laplace formula used adds 19.9dB gain at 1kHz, so for 5mV on the preamp input the AC source (V3 in this example) should be 505.8uV.

The example circuit for Laplace: