As far as I know it's similar to a cascode (except for the partition noise): distortion not very dependent on load impedance as long as you don't drive it into or close to clipping, but distortion similar to that of a triode with a low load resistance - which may be acceptable in the first stage.
It depends on how many valves you are prepared to use. My amplifier with active RIAA correction follows the RIAA curve within +0 dB/-0.3 dB from 20 Hz to 20 kHz without any tweaking, but it uses two triodes, a pentode and a triode-connected pentode per channel. It has a part with flat gain in front of the equalizing stage.
Passive EQ also requires tweaking from calulated values as the calcs are based on 0 drive Z and no load. Both impractical using valves. All EQ must be tweaked either by measurement (good luck with that) or using a sim. I use the the sim method which is fairly simple once up to speed with SPICE.
In defense of pentodes (in general, and for small signals):
One could take the F-Word position that higher gain can always be traded for lower distortion. Not always strictly possible in something as fine-tuned as a phono equalizer, but somewhat true.
Triodes have predominantly second order errors and pentodes third order, so their errors increase with signal level at different rates. The famous quotation is Fig 12.16A from RDH4 p.511 comparing IM distortion of a type 6SJ7 pentode and its triode connected self. Under tested conditions (reasonably typical) IM distortion as a pentode is very much lower than as a triode below an output level of 10V RMS, and remains lower until a level of about 34V RMS, above which roles reverse.
OTOH, is there an active device with as low distortion as a lightly loaded ECC83?
All good fortune,
Chris
One could take the F-Word position that higher gain can always be traded for lower distortion. Not always strictly possible in something as fine-tuned as a phono equalizer, but somewhat true.
Triodes have predominantly second order errors and pentodes third order, so their errors increase with signal level at different rates. The famous quotation is Fig 12.16A from RDH4 p.511 comparing IM distortion of a type 6SJ7 pentode and its triode connected self. Under tested conditions (reasonably typical) IM distortion as a pentode is very much lower than as a triode below an output level of 10V RMS, and remains lower until a level of about 34V RMS, above which roles reverse.
OTOH, is there an active device with as low distortion as a lightly loaded ECC83?
All good fortune,
Chris
Thanks. I'm allergic to lossy networks and to direct coupling (in a phono equalizer? - they never hear of warps I guess), so I'm not the target audience. Still, interesting in a strange but not Japanese or 1930s way, and unique.
Much thanks,
Chris
Much thanks,
Chris
I use the same basic topology (VCCS -> passive EQ -> voltage gain stage) with a lot of discrete bipolar transistors. It is interesting to see a tube version.
Ed
Actually, if you use a low enough Zout from the input stage (for instance using a source follower or cathode follower, or a 6DJ8 or similar input tube with relatively low Zout) and a large enough value series resistor, things seem to come out well. The difficulties happen when you use the output from the plate of a 12AX7 with high Zout that changes as the tube ages, and too small of a series resistor at the input of the RIAA EQ network. That's very common.
I've been using TCJ RIAA to get initial values (it lets you define the Rout of the previous stage) and then entering the results into LTspice, with tweaking from there. I don't have anything more accurate than an inverse RIAA (KAB) and an oscilloscope to measure, but a friend of mine measured a couple of my designs with a PC based test setup and the designs came out very close to flat, within <0.5dB or so.
The Trafomatic Phono looks cool. DC-coupled from input stage to output stage. It uses the input pentode as an anode follower with the RIAA EQ in the feedback loop. I'm not sure what happens as the EF86 ages, though. Do variations in gm between different EF86 tubes bring channel imbalance issues? Maybe not. Perhaps not any more so than differences in mu and gm between sections of a 6N6P.
Last edited:
This is EF86 RIAA with active feedback loop from plate to grid https://commons.wikimedia.org/wiki/File:1950s_EF86_RIAA_phono_stage.png , trafomatic is just passive . It is clever for avoiding the usual series resistor in a passive filter , voltage divider for the useful audio signal .
Last edited:
When calculating the RIAA values, set the smallest RIAA capacitor at a value that is large enough
to make second stage circuit parasitics small, say around 1000pF. Then determine the other RIAA components.
From the calculated series RIAA resistor, subtract the source resistance of the input tube circuit (which includes
the grid resistor to ground). Any further RIAA trimming should only be with this resistor, not the other RIAA parts.
On second look, you're correct! (oops)
I never considered putting the passive filter network in the plate load of the input stage.
I was thinking that makes the output impedance of the EF86 stage go all over the place, but the DC-coupling to a triode with extremely high input impedance would make that a non-issue. Very clever.
Thanks for the correction.
This is where having extra gain, and trading-off gain for consistent performance, is useful. I said in another thread that a good preamp requires more than two transistors. That can also apply to tubes.
Ed
I've heard so many times where folk think they can calculate the values for RIAA EQ and be done because they can calculate output Z and put that into the formula. This approach ignores the variable load the EQ places on the driver and unless there's a massive difference between the load and drive Z, which almost never happens with valves, the calc'd values are off esp in the LF. Mid to HF is easy. Unless you sim it or measure it, you won't see what I mean unless +/- 0.5dB is OK for you then that might work. I don't know as I aim for much tighter tolerances. Each to their own of course.
Like I wrote in post #22, my amplifier was within +0 dB/-0.3 dB with respect to the ideal RIAA curve without any tweaking, neither in simulation (I didn't simulate it at all) nor in reality. That's measured performance, worst of two channels, 20 Hz to 20 kHz with respect to the gain at 1 kHz, with active RIAA correction (this thread is about active RIAA correction after all) and using second-hand valves.
The passive RIAA network is indeed a voltage divider, that is exactly how it works.
Unless the first stage coupling capacitor is too small, or the second stage Miller capacitance loading is too large,
there is no need or reason to adjust the calculated RIAA component values.
Just subtract the source resistance of the first stage from the calculated value of the series RIAA resistor.
This completely accounts for the said variable loading of the RIAA on the first stage.
That is a simplistic approach and won't, for the most part, give an accurate result due to the variable loading on the driver unless it has a super low Z compared to the EQ. I wouldn't feed the EQ with a cap, IMO. The variable loading affects the LF. Simple calculators don't take into account this loading.
Don't take my word for it. Sim it easily or spend ages calulating it and you'll see.
I'm afraid Stephen is right about the effect of an AC coupling capacitor in a passive RIAA design. Correcting for the resistance of the first stage is easy, just subtract it from the resistance of the first resistor, correcting for the coupling capacitor is not.
Simplified case: replace the passive RIAA correction network with one capacitor and pretend there is no resistor to ground needed after the AC coupling. It's then immediately obvious that the series connection of the AC coupling capacitor and the filter capacitor sets the time constant, rather than the filter capacitor on its own. If you allow 1 % error, the AC coupling capacitor has to be about 100 times as large as the filter capacitor.
The mathematics are more complicated for a real RIAA correction network, but I would expect the trend to be the same: both poles sensitive to the AC coupling capacitance, especially the lowest pole. Either make the AC coupling capacitance 100 times larger than the sum of the values of the RIAA correction capacitors or treat the whole thing as a third-order filter network. The zero will just stay where it is supposed to be.
To make matters worse, when the first stage is a triode with decoupled cathode resistor, the cathode decoupling also affects the first stage's output reactance.
I'll try to come up with an analytical solution with AC coupling capacitor included (but without cathode decoupling) for the network of this thread: https://www.diyaudio.com/community/...a-calculator-formula-help.401437/post-7405500 It has the more conventional network as a special case.
Simplified case: replace the passive RIAA correction network with one capacitor and pretend there is no resistor to ground needed after the AC coupling. It's then immediately obvious that the series connection of the AC coupling capacitor and the filter capacitor sets the time constant, rather than the filter capacitor on its own. If you allow 1 % error, the AC coupling capacitor has to be about 100 times as large as the filter capacitor.
The mathematics are more complicated for a real RIAA correction network, but I would expect the trend to be the same: both poles sensitive to the AC coupling capacitance, especially the lowest pole. Either make the AC coupling capacitance 100 times larger than the sum of the values of the RIAA correction capacitors or treat the whole thing as a third-order filter network. The zero will just stay where it is supposed to be.
To make matters worse, when the first stage is a triode with decoupled cathode resistor, the cathode decoupling also affects the first stage's output reactance.
I'll try to come up with an analytical solution with AC coupling capacitor included (but without cathode decoupling) for the network of this thread: https://www.diyaudio.com/community/...a-calculator-formula-help.401437/post-7405500 It has the more conventional network as a special case.
Last edited:
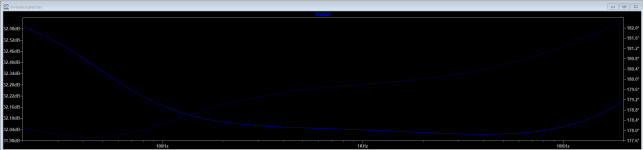
A simple example and fairly typical. D3a driving EQ with 20k series R. This graph is just the load on the D3a anode. Simple calculators don't take this into account. IOW, the drive Z varies with frequency coz EQ in parallel with driver anode load. As Marcel says. there's also the stage bypass cap to consider and if you use one, the cathode bypass and the cap to the next stage (not part of the above graph). That's quite a bit of frequency dependent calculating. Sure it can be done but why not just sim it. This for passive EQ of course.
Last edited:
An exact solution for a passive RIAA network with AC coupling got too complicated for me, but I did find an approximate solution with the coupling capacitor included, see https://www.diyaudio.com/community/...a-calculator-formula-help.401437/post-7439558 In a numerical example I checked with a pole-zero extraction program, the largest RIAA time constant (the one that's most affected) was off by about 0.207 %.