You have to consider transistor's hFe too, it varies with temperature.
For instance, an un-cascoded VAS will have huge instantaneous power dissipation variations translating into temperature changes. Since (assuming a current mirror above the input pair -> VAS a la Self) the important parameter for the VAS gain is hFe, which appears in the open loop gain, it follows that the OL gain of the whole amp is dependent on the temperature of the VAS transistor.
I think we'll be hand-waving until we can get simulations.
Now the question is, where to get models.
For instance, an un-cascoded VAS will have huge instantaneous power dissipation variations translating into temperature changes. Since (assuming a current mirror above the input pair -> VAS a la Self) the important parameter for the VAS gain is hFe, which appears in the open loop gain, it follows that the OL gain of the whole amp is dependent on the temperature of the VAS transistor.
I think we'll be hand-waving until we can get simulations.
Now the question is, where to get models.
Hi Sébastien,
No, by this way you will measure something different. You need 1kHz carrier, modulated by 3Hz envelope and you should restore this 3Hz modulation and measure its distortion.
anli said:BTW, why must we try to measure MD in terms of spectrum? Why must we do long, hard and not intuitive job of such concepts convertingOut ears have nothing common with spectrum-meter.
To the contrary, they do. The ear is a real-time spectrum analyzer, or at least something similar. So it is probably very appropriate to do such measurements.
Sure, until we know enough about something and know exactly what is relevant to meausre, it is preferrable to measure everything that might possibly matter. The problem is that I still think it is hard to measure MD and be sure if it is MD we measure, ecxcept for using something like the dual-transistor method I suggested.
Christer said:
I have not read any psicho-acoustics results proving it. Have you some public links to such results?
Can you explain it in more details? I have missed something... 🙂Christer said:
I think these are the patents pertaining to the Kinergetics Research techniques.
United States Patent 4,622,660
Cowans, et al. November 11, 1986
Systems and methods for signal compensation
The distortion effects introduced in a complex multifrequency wave by parametric variations in individual active elements arising from signal and power supply variations in a circuit, such as an audio amplifier, are compensable through the use of replicas of the active elements, and the generation of a feedback signal incorporating comparable distortion. By high gain amplification of the feedback signal in a differential amplifier receiving an input signal that is not comparably distorted a comparison signal is derived containing distortion components. The comparison signal is used in a feedforward path which includes the circuit that is subjected to parametric variations in a sense to cancel the introduced distortions.
United States Patent 4,549,146
Cowans, et al. October 22, 1985
Systems and methods for compensating for element nonlinearities in electronic circuits
The distortion effects introduced in a complex multifrequency wave by parametric variations in individual active elements arising from signal and power supply variations in a circuit, such as an audio amplifier, are compensable through the use of replicas of the active elements, and the generation of a feedback signal incorporating comparable distortion. By high gain amplification of the feedback signal in a differential amplifier receiving an input signal that is not comparably distorted a comparison signal is derived containing distortion components. The comparison signal is used in a feedforward path which includes the circuit that is subjected to parametric variations in a sense to cancel the introduced distortions.
United States Patent 4,426,552
Cowans, et al. January 17, 1984
Speaker distortion compensator
Regards
James
anli said:
It is rather physiology than psychoacoustics. I am absolutely no expert on this, but essentially the cochlea is a narrowing spiral. As far as I understand one can think of it has having successively higher resonance frequency, the narrower it gets, that is, the further into the cochlea we get. The hair cells that detect sounds, or rather movements in the fluid in the ear, are distributed along the whole cochlea and they respond to different frequencies, corresponding to the local resonance frequency of the cochlea at the point of the cell. This is an oversimplification and probably not quite correct in detail, but I think it is basically right. What this means is that the brain probably gets a feed of instantaneous spectrum samples, rather than amplitude samples or similar.
There was a discussion about this on the forum some time ago, and somebody mentioned very recent research which had given further understanding. I think it turned out that the hair cells did not quite respond to frequency, but wave propagation speed, or something simliar, which made it possible to detect transients while they occur.
I suggested it in one of my first posts in this thread. The idea was to use a dual single-die transistor, like the MAT-02, or something cheaper. Then make two identical amplifier stages, using one of transistors for each stage. Since the transistors are tightly matched the two amplifiers should have almost identical Q conditions. Then feed one of the amplifiers with an input signal of whatever kind you wish, sines, short spikes, Beethovens 9th symphony or whatever. Keep the input to the other amplifier grounded. Since the transistors reside on the same die they are also tightly coupled thermally, so the thermal effects that arise in the first amplifier should affect the Q point not only of itself but also of the other amplifier. Since this other amplifier has no input signal, the only output from it will be the Q point variations which should mimic those of the other amplifier, that is the output of the second amplifier is thermal error in the first amplifier (not exactly, but probably very close to it). This would provide a method to measure the thermal error more directly than by measuring the output of a working amplifier. As far as I can see there shouldn't be much coupling between the two transistors except for thermal coupling, which on the other hand should be a very tight one. Hence this seems to me a much more accurate method to measure the thermal effects.
You see, too many new ideas in these area. It is distinct sign there isn't adequate theory, I think 🙂 For me, it is more intriguing a concept treating our "ear system" as (also) a comparator of sequential time-fragments with length about 50-200mS. Such fragments must be strongly correlated in blameless audio chain. This the chain time-stability idea is very close to MD, I think.Christer said:
Christer said:
Aha-aha, I see (have downloaded MTA02 data-sheet 🙂 ). I'm absolutely beyond transistors technology, so I can not judge, if all or some only thermo time constants will be shared in such device.
anli said:
Well, it is the same die and one of reasons for using such transistors, except that they are matched, is that they are thermally coupled so leakage currents etc. drift equally in both. It is most certainly not 100 % coupling between them so we won't get an exact measurement of the thermal effects in the actice transistor, but I expect it should be fairly close so we see what the thermal distorsion signal looks like and get also get an approxmiate measurement of its magnitude (say within a factor 2 at least). Also the phase may lag a bit compared to the other transistor. So even if we don't ger a perfect quantitative reading, it will be rather good and free of other influencing factors (as far as I can see).
If I understand English terms well, die is a part where a crystal lives. So, using MTA02, we'll loose most "fast" thermo-transition, i.e., crystal -> die (others are die -> case, case -> ambient). So, results will be "low-passed-filtered". Correct me, if I'm wrong, please.
anli said:
The die is the actual piece of silicon that is the transistors. Maybe I use the term wrong, but I think die is correct. One could also call it a chip, but I think that is more commonly used for ICs than transistors. Or maybe I am just confused by the terminology? 🙂
Anyway, Both transistors are on the same little piece of silicon so there should be a much tighter coupling between the transistors than from transistor to case. Of course there will be some delay between the transistors, though. So it is not a perfect measurement method.
why did you forget about the SMTE IMD distortion test - much more sensitive to thermal effects than 2nd harmonic
I have shown that it is possible to have huge IMD that can only be excited by multiple input test tones - with a single tone this sim shows -74 db thd, and 23% IMD with 2 tones!
http://www.diyaudio.com/forums/showthread.php?postid=492499#post492499
http://www.diyaudio.com/forums/showthread.php?postid=492928#post492928
as I point out thermal modulation could be expected to behave similarly to the sim - requiring multi-tone tests
and "the formula" for practically eliminating thermal distortion in the input diff pair is simple:
cascode - bootstrapped to the common mode input V
and high loop gain after the diff pair to reduce diff signal - easily down to uW
I have shown that it is possible to have huge IMD that can only be excited by multiple input test tones - with a single tone this sim shows -74 db thd, and 23% IMD with 2 tones!
http://www.diyaudio.com/forums/showthread.php?postid=492499#post492499
http://www.diyaudio.com/forums/showthread.php?postid=492928#post492928
as I point out thermal modulation could be expected to behave similarly to the sim - requiring multi-tone tests
and "the formula" for practically eliminating thermal distortion in the input diff pair is simple:
cascode - bootstrapped to the common mode input V
and high loop gain after the diff pair to reduce diff signal - easily down to uW
Hej Christer
It is vice-versa the basilar membrane is sensitive for the high frequencies at its beginning and for low frequencies at its (narrow) end.
Regards
Charles
Hello Guys,
We could conclude that MD has much significance in power amps...but upto how much extent it bears its significance....
We could conclude that MD has much significance in power amps...but upto how much extent it bears its significance....
Check this documents on pulsed transistor measurements.
http://home.peufeu.com/nik/sischka_hicws03.pdf
http://home.peufeu.com/nik/pulsed transistor measurements.pdf
AH ! I found it. So, the new models we need to investigate (rather than messing with subcircuits) are VBIC and MEXTRAM : both handles self-heating.
http://www.ece.uci.edu/docs/hspice/hspice_2001_2-110.html
http://www.ece.uci.edu/docs/hspice/hspice_2001_2-112.html#96481
http://home.peufeu.com/nik/sischka_hicws03.pdf
http://home.peufeu.com/nik/pulsed transistor measurements.pdf
AH ! I found it. So, the new models we need to investigate (rather than messing with subcircuits) are VBIC and MEXTRAM : both handles self-heating.
http://www.ece.uci.edu/docs/hspice/hspice_2001_2-110.html
http://www.ece.uci.edu/docs/hspice/hspice_2001_2-112.html#96481
And about THD :
http://home.peufeu.com/nik/steadystatedisto.png
This circuit shows good steady-state measurements... once the cap is charged to the peak test sine voltage, it almost disappears... but try to run music through it.
http://home.peufeu.com/nik/steadystatedisto.png
This circuit shows good steady-state measurements... once the cap is charged to the peak test sine voltage, it almost disappears... but try to run music through it.
Hi TVI,
Thanks for your links. I've been searching these patents for a long time.
Hi Dimitri,
===I propose another method. Have a DC power supply which output of is modulated by a 3 Hz sine amplitude of some volts. The device under test is submitted to a very classical but very sensible THD test at, say, 1V 1000 Hz. If the output has some temperature dependence, the THD value will show variations at a rate of 3 Hz.===
---No, by this way you will measure something different.---
What will I get ?
I find Christer's idea (input transistors of two different amplifiers on a same die) a good way to concretely show the real influence of thermal effects. If I remember well, more than fifteen years ago, Gérard Perrot failed in his attempts to demonstrate the existence of memory distorsion in a standard amplifier made by JVC.
Thanks for your links. I've been searching these patents for a long time.
Hi Dimitri,
===I propose another method. Have a DC power supply which output of is modulated by a 3 Hz sine amplitude of some volts. The device under test is submitted to a very classical but very sensible THD test at, say, 1V 1000 Hz. If the output has some temperature dependence, the THD value will show variations at a rate of 3 Hz.===
---No, by this way you will measure something different.---
What will I get ?
I find Christer's idea (input transistors of two different amplifiers on a same die) a good way to concretely show the real influence of thermal effects. If I remember well, more than fifteen years ago, Gérard Perrot failed in his attempts to demonstrate the existence of memory distorsion in a standard amplifier made by JVC.
peufeu said:
True. It will matter in some circuits but not in others.
It is not clear to me what causes this effect. My semiconductor physics book does not say anything about it, although it obviously recognizes it by showing an example diagram where hFE varies with temperature. Perhaps the reason is that one shall realize this as a consequence of two other effects, temp. dependence of base leakage current and temp. dependence of high injection, both which affect hFE a lot.
phase_accurate said:
Really? I obviously never read that, or forgot it because it seems counterintuitive. I stand corrected.
It seems that thermal effects can cause two not entirely different effects. One is that a sufficiently low-frequency signal modulates the Q point due to temperature variations. The other is that a sudden transient will cause the Q point to shift for thermal reasons.
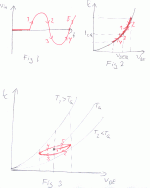
I have been thinking a bit about these and I think it might be helpful to view the effects from a slightly different point of view, more precisely by looking at what happens in the Vbe-Ic diagram when a transient signal is applied. I first tried to figure out what ought to happen, and came up with the following, which have followed up by simulations soon to be posted.
Fig. 1 shows the input signal, which is 0 V until a we suddenly apply a sine wave, ie. we have a transient.
Fig. 2 shows what happens in a class A CE stage when thermal effects are not considered. We will simply move along the transfer curve around the Q point, as expected.
Fig. 3 is an attempt to envision what happens if we also take thermal effects into consideration. For clarity, I have added to extra transfer curves to show what happens to the transfer curve when the temperature varies. Now, consider applying the transient sine from Fig. 1. As long as the input is 0 V, we stay at the Q point (assuming we have had time to reach thermal equilibrium). As soon as the voltage starts to increas because of the onset of the sine, both Vbe and Ic will increase, just as in Fig. 2. However, since the power will decrease simultaneusly, so will the temperature and we will move a long a transfer curve that is sliding from the Tq one towards the T2 one. We will thus not follow the transfer curve for Tq but move towards the transfer curve for T2 while Vbe and Ic increase. When Vbe and Ic stats to decrease again, the power will slowly increase and we will move towards the transfer curve for Tq and eventually towards the one for T1. However, when passing Vbeq, the temperature will still be lower than Tq, so we pass below the Q point!When Vbe and Ic finally starts to increase again to conclude the first cycle, the transistor will be warmer than Tq, so we will pass above the Q point, which means the cycle does not end where it started! Further cycles will continue to "draw" a somewhat curved elliptical shape that encircles the Q point. Since the average power with a cyclic signal is lower than the Q power, the ellipsis will pass closer to the Q point on its upper path than on its lower path.
Hugh talked about hysteresis before, and this seems to have some similarities to hysteresis. In fact, the behaviour in Fig. 3 resembles the virgin curve for a magnetic material.
Simulation results will follow.
I have been thinking a bit about these and I think it might be helpful to view the effects from a slightly different point of view, more precisely by looking at what happens in the Vbe-Ic diagram when a transient signal is applied. I first tried to figure out what ought to happen, and came up with the following, which have followed up by simulations soon to be posted.
Fig. 1 shows the input signal, which is 0 V until a we suddenly apply a sine wave, ie. we have a transient.
Fig. 2 shows what happens in a class A CE stage when thermal effects are not considered. We will simply move along the transfer curve around the Q point, as expected.
Fig. 3 is an attempt to envision what happens if we also take thermal effects into consideration. For clarity, I have added to extra transfer curves to show what happens to the transfer curve when the temperature varies. Now, consider applying the transient sine from Fig. 1. As long as the input is 0 V, we stay at the Q point (assuming we have had time to reach thermal equilibrium). As soon as the voltage starts to increas because of the onset of the sine, both Vbe and Ic will increase, just as in Fig. 2. However, since the power will decrease simultaneusly, so will the temperature and we will move a long a transfer curve that is sliding from the Tq one towards the T2 one. We will thus not follow the transfer curve for Tq but move towards the transfer curve for T2 while Vbe and Ic increase. When Vbe and Ic stats to decrease again, the power will slowly increase and we will move towards the transfer curve for Tq and eventually towards the one for T1. However, when passing Vbeq, the temperature will still be lower than Tq, so we pass below the Q point!When Vbe and Ic finally starts to increase again to conclude the first cycle, the transistor will be warmer than Tq, so we will pass above the Q point, which means the cycle does not end where it started! Further cycles will continue to "draw" a somewhat curved elliptical shape that encircles the Q point. Since the average power with a cyclic signal is lower than the Q power, the ellipsis will pass closer to the Q point on its upper path than on its lower path.
Hugh talked about hysteresis before, and this seems to have some similarities to hysteresis. In fact, the behaviour in Fig. 3 resembles the virgin curve for a magnetic material.
Simulation results will follow.
Attachments
- Status
- Not open for further replies.