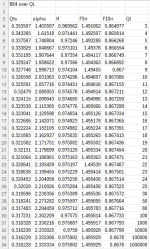
These alignments are talked about in the Loudspeaker design cookbook and described as "Discrete alignments" that basically wiork only for one Qts value for any given Ql or box loss factor. I computed the values of Qts, alpha=Vas/Vb, H=Fb/Fx, F3m=F3/Fs, F10n=F10/Fs for values of Ql from 3 to 1Million 😉 for both bessel and butterworth alignments.
As a bonus, I compare the values given by QB3 and SC4 alignments for woofers with a Q of 0.32 - similar to a Bessel aligned woofer. FWIW, QB3 , SC4, and B4 alignments are all identical the B4 value of Qts.
The value of the Bessel alignment is that it has maximally flat delay and a lower F10 than either QB3 or SC4.
As a bonus, I compare the values given by QB3 and SC4 alignments for woofers with a Q of 0.32 - similar to a Bessel aligned woofer. FWIW, QB3 , SC4, and B4 alignments are all identical the B4 value of Qts.
The value of the Bessel alignment is that it has maximally flat delay and a lower F10 than either QB3 or SC4.
Attachments
All loudspeaker crossovers must strike a balance between frequency domain and time domain performance. In this regard, all Bessel crossovers of any order have very good time domain characteristics but poor frequency domain selectivity, meaning that they roll off very slowly even when the order is high. For this reason they make for a poor choice in a crossover type or an alignment for a closed box application.
In any case, nice work.
In any case, nice work.
Charlie, these are for bass reflex alignments. For a sealed alignment, bessel is just Qtc=0.577, and there is nothing really unsatisfactory about that.
For Ql=7 as a comparison:
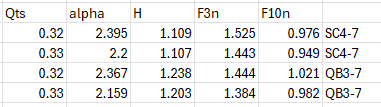
B4 is Qts=0.4, Vb=Vas/1.06, Fb=Fs, F3=Fs and F10=0.76*Fs
BE4 is Qts=0.33 Vb=Vas/1.9, Fb=0.97*Fs, F3=1.5*Fs and F10=0.87*Fs
SC4 is Qts=0.33 Vb=Vas/2.2, Fb=1.1*Fs, F3=1.4*Fs and F10=0.95*Fs
QB3 is Qts=0.33 Vb=Vas/2.2, Fb=1.2*Fs, F3=1.4*Fs and F10=0.98*Fs
Bessel has a bigger box, is tuned lower and rolls off slower than either SC4 or QB3 at the same Qts.
Bessel has maximally flat group delay.
Butterworth is maximally flat amplitude
Bessel can be used on crossover filters, but they don't sum flat like L-R filters do, AFAIK. They are probably better applied if you just need a band limiting highpass or lowpass, but you are the filter guy...
For Ql=7 as a comparison:
B4 is Qts=0.4, Vb=Vas/1.06, Fb=Fs, F3=Fs and F10=0.76*Fs
BE4 is Qts=0.33 Vb=Vas/1.9, Fb=0.97*Fs, F3=1.5*Fs and F10=0.87*Fs
SC4 is Qts=0.33 Vb=Vas/2.2, Fb=1.1*Fs, F3=1.4*Fs and F10=0.95*Fs
QB3 is Qts=0.33 Vb=Vas/2.2, Fb=1.2*Fs, F3=1.4*Fs and F10=0.98*Fs
Bessel has a bigger box, is tuned lower and rolls off slower than either SC4 or QB3 at the same Qts.
Bessel has maximally flat group delay.
Butterworth is maximally flat amplitude
Bessel can be used on crossover filters, but they don't sum flat like L-R filters do, AFAIK. They are probably better applied if you just need a band limiting highpass or lowpass, but you are the filter guy...
Last edited:
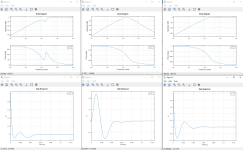
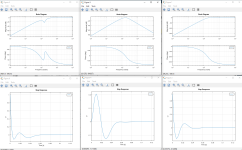
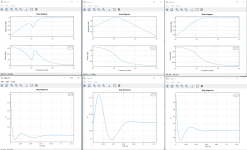
Here is some more simulation stuff on alignments: All of these have an F3 of 30Hz The B4 has a Qts of 0.4048, all of the other alignments used a Qts~0.33 to be comparable to the Bessel. Included are Butterworth4, Bessel4, QB3, SC4 and BB4 The component responses are from left to right, Diaphragm , port and summed (box) response. The top plot is the bode plot in radians per second 30cycles/sec*2*pi rad/cycle ~188 rad/s. The bottom plot is Step response. The Bessel and BB4 have smoother step response plots, and indeed are close in volume and tuning.
Attachments
They were created by Thiele, Small & others at a time when there were no personal calculator or computer software available for flexible modelling. So they were in certain regards an advance over prior methods since the tabulated values provided in various papers & articles allowed builders to hit a consistent alignment -although Bullock for one always advocated oversizing enclosures by at least 10% & sometimes up to 25% to account for variations in real-world driver parameters -most commonly suspension compliance. Not a lot changes in that regard... 😉
The 'discrete alignments' like B4 were actually the first to be worked up, only afterward followed by the more flexible QB3 etc., since they're mathematally simpler requiring a fixed Qt value to be achieved (remember the object of the original papers was to present the theory & underlying maths rather than a freeform design method as such).
The 'discrete alignments' like B4 were actually the first to be worked up, only afterward followed by the more flexible QB3 etc., since they're mathematally simpler requiring a fixed Qt value to be achieved (remember the object of the original papers was to present the theory & underlying maths rather than a freeform design method as such).
Last edited:
They are mathematical abstractions. Butterworth 4th order is Maximally Flat amplitude response, Bessel 4th order is maximally flat delay. Both can be arrived at by minimizing or maximising a particular response function. C4 is Chebychev 4th order, which has an equal ripple response characteristic and SC4 is an extension of that for low Q drivers. QB3 is a quasi 3rd order alignment which happens to have the lowest cutoff relative to box size of all of these alignments. BB4 (attributed to Hoge) is a very simple function alpha=1/4*(1/Qts-1/Ql) (Thanks Claus!) and Fb=Fs. Because the tuning tends to be lower than other alignments, it tends to have a better step response, but it rolls off early with low Q drivers.
All of these alignments still have considerable merit today as starting points in design, but there is no reason to slavishly stick to them as recommended in older texts. Basically there are two degrees of freedom in a vented box system, Box volume and tuning frequency. You can select Fb from maybe 1/2 to 2x the Fs and Box volume from perhaps 1/3 to 3X the Vas (just to pick some numbers) and the alignments I have shown are only a few points in that 2D space. There is a lot more freedom in design, but you may find that the areas with acceptable response tend to be near the alignments that people say are now "useless".
YMMV.
These alignments cannot even be applied to a full lumped ported box / passive radiator model with port, box, and leakage damping because there is a coefficient in front of the s^4 term in the denominator. That's OK though, because a model with Just Ql does most things pretty well and has teh advantage that it models sealed by setting Fb=0.
All of these alignments still have considerable merit today as starting points in design, but there is no reason to slavishly stick to them as recommended in older texts. Basically there are two degrees of freedom in a vented box system, Box volume and tuning frequency. You can select Fb from maybe 1/2 to 2x the Fs and Box volume from perhaps 1/3 to 3X the Vas (just to pick some numbers) and the alignments I have shown are only a few points in that 2D space. There is a lot more freedom in design, but you may find that the areas with acceptable response tend to be near the alignments that people say are now "useless".
YMMV.
These alignments cannot even be applied to a full lumped ported box / passive radiator model with port, box, and leakage damping because there is a coefficient in front of the s^4 term in the denominator. That's OK though, because a model with Just Ql does most things pretty well and has teh advantage that it models sealed by setting Fb=0.
My intent when posting this info on discrete alignments was to follow up fairly quickly with the step response stuff I posted today just to see how much better Bessel was than Butterworth. I was curious what the other component responses (diaphragm and port) looked like, and the other alignement types, so I added those as well. It might help someone somewhere, or might just be general interest, maybe nobody cares 😉
The following table was taken from Thiele's 1961 journal paper "Loudspeakers in Vented Boxes." It seems apparent that the B4 and QB3 alignments were published together.
Bessel aliments have maximally delay only for low pass alignments.
Anyone remember John Bau of Spica? Interesting interview with him about 4th order Bessel low-pass filter + 1st order Butterworth high-pass to optimize group delay in the sloped-box TC-50. https://www.stereophile.com/interviews/694/index.html