H
HAYK
I have confused with another type of filter, I don't know which, that limits to 80khz.
This means 4db S/N gain because of paralleled.
This means 4db S/N gain because of paralleled.
On a side note: could a hardware-based random dither generator be retrofitted in a multibit DAC, i.e. adding dither to the LSB? Has anybody done it?
You could make a thing that accepts a 24 bit or 32 bit I2S or S/PDIF signal, rounds it to 14 or 16 bit with selectable dither and again outputs it as I2S or S/PDIF. Some of it could probably be done with an ASRC chip in bypass mode.
You are welcome Hayk
A warning. Comparing noise figures from different sources is doomed to provide false results, unless all test parameters are published and meticulously duplicated.
This test I did, wasn't meant to and does not qualify for testing noise reduction due to paralleling DAC chips.
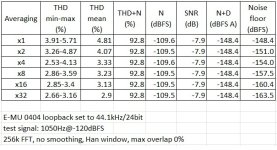
Please notice that for that test I had opted for no averaging, thus THD figures are highish.
Also, THD numbers shown on results window of the software are not definite-fixed numbers. They vary over time if someone watches the screen, even after the number of selected averaging has passed. I had done a test to check the effect of averaging over THD and noise numbers, see attachment.
What changes predictably is the noise floor (-3 db for each average doubling).
THD fluctuates but the mean drops (this operation has to be done manually).
All other reported properties don't change
The measured effect is significant well below -60dB (for 16 bit data). The perceived audible effect varies among listeners.
The topic was hot btn 2015 and 2017 in this forum. Search for "dither" and "Scott Wurcer" or "gpapag"
George
Attachments
If the 8 dither options in the PMD100 are not to your liking you can always roll your own in a DSP chip.
H
HAYK
I made some simulation about A weighted and George's measurements 850hz to 21khz.
This in blue is the A filter.
I generated a white noise by zener and filter it approximately.
The total noise for 20khz BW is 510uv.
When measured without filter but from 850hz to 21khz as George did I have 770uv
This gives 3.64db difference.
Now can conclude that 4x// increased the S/N by 4.64db.
This in blue is the A filter.
I generated a white noise by zener and filter it approximately.
The total noise for 20khz BW is 510uv.
When measured without filter but from 850hz to 21khz as George did I have 770uv
This gives 3.64db difference.
Now can conclude that 4x// increased the S/N by 4.64db.
H
HAYK
He measured CD players by test CD with different DACs.
By selecting among 50 pcm56, he could find miraculous one with no distortion.
But what amazing, is the Teac ZD700, also very clean but with some extra noise, probably dithered.
Post in thread 'New DAC measurement' https://www.diyaudio.com/community/threads/new-dac-measurement.49778/post-616228
He will study it in more detail.
https://www.diyaudio.com/community/threads/strange-teac-dac.56902/
The confusing element is the decimation is also applied which makes difficult to see the real reason of so low distortion.
This what comes out from the DAC pcm53.
And this is noise generator probably.
I don't understand how the circuit generates both noise and decimate.
Decimating is similar to 2xoversampling by stuffing zeros between samples.
Last edited by a moderator:
Well, I haven't read Bernhard's thread yet, TEAC uses PCM53 in time-multiplexed manner, one period is signal+dither and the other is sole dither to subtract them after DA conversion. (and L+R channel multiplex for single DAC model)
The sole dither is sample-hold-ed and then subtracted on signal +dither period. The subtracted signal (No.7) is still time-multiplexed thus need another sample-hold.
On some models, TEAC used 2DAC par channel, signal with opposite polarity dither to eliminate time-multiplexing and sample-hold.
The sole dither is sample-hold-ed and then subtracted on signal +dither period. The subtracted signal (No.7) is still time-multiplexed thus need another sample-hold.
On some models, TEAC used 2DAC par channel, signal with opposite polarity dither to eliminate time-multiplexing and sample-hold.
I built a sine generator that sends 440 Hz -60 dB I2S signal, digitally generated. I measured a TDA1541A in-situ, and the THD was around 0.15%. The 3rd harmonic component dominated.
I did not apply any dither, because the 440 Hz sine and the 44100 Hz sampling rate are not harmonically related.
It is said that non-harmonically related tones and sample rates make quantizing distortion harder to hear. Along those lines, the argument can go on to say that since music rarely consists of constant tones harmonically related to the sample rate, then no dither should be needed for music recordings.
Notwitstanding for the moment the issue of extending bit-depth with dither, of course the above extended argument about not needing dither is known to be false because some people can hear quantizing distortion in the few old undithered 16-bit recordings of music. Some people can even hear triangular dither noise, and object to the sound of it. For that reason much more sophisticated adaptive noise-shaped dithers are often used for CD mastering in today's world.
Is it possible to reduce the "fur" in the spectrum? I tried 1k + 150pF filter in the digital inputs with very minimal effect.
When I stop the input, the spectrum is clean.
When I stop the input, the spectrum is clean.
There is going to be noise, or something that looks like noise on an FFT. If you start with something like a 24-bit signal, then dither it properly, then truncate to 16-bits, you should effectively have a little more than 16-bits but with the lower bits buried in noise. Then the noise floor appearance should be reduceable by decreasing bin width (increasing FFT size), and the noise floor can also be LP filtered by averaging multiple spectrums. In theory, then you may be able to see waveforms reconstructed with a little better than 16-bits of resolution. This assumes your output stage can reconstruct all the effective bits including those buried in noise.
ESS dacs are not known for being spur free. Depends on MCLK frequency, etc.
Other than that, proper dither adds noise at the LSB and below the LSB (before truncation) The noise below the LSB sort of adds with the test signal below the LSB, such that some of the test signal summed with noise percolates up above the LSB some of the time (i.e. when summing of dither noise and low-level signal produces a carry bit, with the carry bit affecting at or above the post-truncation LSB). Hence some of the low level test signal information will still be there after truncation, its just buried in noise. It means that proper dithering actually reduces distortion, it doesn't just "hide" the quantizing distortion.
Other than that, proper dither adds noise at the LSB and below the LSB (before truncation) The noise below the LSB sort of adds with the test signal below the LSB, such that some of the test signal summed with noise percolates up above the LSB some of the time (i.e. when summing of dither noise and low-level signal produces a carry bit, with the carry bit affecting at or above the post-truncation LSB). Hence some of the low level test signal information will still be there after truncation, its just buried in noise. It means that proper dithering actually reduces distortion, it doesn't just "hide" the quantizing distortion.
Last edited:
They have a beat frequency of 20 Hz: 22 times 20 Hz and 2205 times 20 Hz. You see all multiples of 20 Hz in your measured spectrum due to the lack of dithering.
Even without any such relation, you would still get harmonic distortion when you don't use dither. Just imagine you pass a small sine wave through a coarse quantizer without any sampling: you change it from a sine to a periodic staircase-shaped waveform, which has harmonics in its Fourier series.
How do I implement the dither? I generate the I2S with ESP8266. Is it a random 1-bit amplitude digital noise?
You calculate the sine with high accuracy, and then you add n independent uniformly distributed random or pseudorandom signals with a peak-peak value of 1 LSB of 16 bit before rounding the sum off to 16 bit. You also have to make sure you don't get overflows.
n = 1 suffices to get rid of the distortion peaks in the spectrum (the ones due to rounding to 16 bit that is, not those due to DAC imperfections), but n = 2 is more usual for audio because it also makes the RMS value of the requantization noise independent of the signal.
Have you got a uniform pseudorandom number generator at your disposal?
n = 1 suffices to get rid of the distortion peaks in the spectrum (the ones due to rounding to 16 bit that is, not those due to DAC imperfections), but n = 2 is more usual for audio because it also makes the RMS value of the requantization noise independent of the signal.
Have you got a uniform pseudorandom number generator at your disposal?
