some drivers have quite deep cone profiles and other have a lot shallower ones, what sonic differencies will this make? i have the impression that shallower cones sounds more open or big then deep cones, is there really any difference in "wave front"?
The cone profile also affects the cone break-up behaviour and frequency response. This pdf may be a useful point of reference:
http://resources.prismsound.com/tm/LOUDSOFT_Cones_stiffness_ALMA_2003.pdf
http://resources.prismsound.com/tm/LOUDSOFT_Cones_stiffness_ALMA_2003.pdf
... and some have a straight profile, while others have a parabolic or eliptical flare profile. A driver designer balances many aspects, so there are no hard and fast rules.
An ideal infinitely stiff cone will have a cancellation null on axis when the distance from the bell of the cone to the base of the cone is equal to approximately 1/2 wavelength... this is simplifying it somewhat, but basically this is when it starts. Off axis it is more complicated and it starts at a lower frequency. The deeper the cone profile, the lower the frequency when this starts.
For paper, pulp, and polymer cones, this may not matter much because the first mode resonance will probably be lower than the start of the cancellation null. If the cone is not moving in unison as a piston, there may be little cancellation null.
A deeper cone is stiffer than a shallower cone. A deeper cone has more mass than a shallow cone. A flared cone may go into breakup in a more gentle way than a straight sided cone.
There is a lot going on with cone shape.
An ideal infinitely stiff cone will have a cancellation null on axis when the distance from the bell of the cone to the base of the cone is equal to approximately 1/2 wavelength... this is simplifying it somewhat, but basically this is when it starts. Off axis it is more complicated and it starts at a lower frequency. The deeper the cone profile, the lower the frequency when this starts.
For paper, pulp, and polymer cones, this may not matter much because the first mode resonance will probably be lower than the start of the cancellation null. If the cone is not moving in unison as a piston, there may be little cancellation null.
A deeper cone is stiffer than a shallower cone. A deeper cone has more mass than a shallow cone. A flared cone may go into breakup in a more gentle way than a straight sided cone.
There is a lot going on with cone shape.
I always though that there may me something to dome diaphragms just because of the Atc sm75-150s (and volts dome mid) success....and the success of it with tweeters as well...
Cone apex angle influences a cone rigidity and a loudspeaker upper limit frequency. Below is a quote from old Russian book (D.Shifman, Gromgogovoriteli (Loudspeakers), but you can use yandex or google translation service if you interesting in details) [/URL]
The deeper cone is (=lower apex angle), the higher upper limit frequency is. But at the same time by lowering apex angle you lower rigidity of the cone, thus the cone break-up frequency also become lower. So, there is the trade off between the cone structural rigidity and the upper limit frequency.
Last edited:
New trick is to use variable stiffness, older tricks are eg. slits and glue rims
Speaker Cones — Fabrication, Materials, and Performance | audioXpress
https://www.klippel.de/fileadmin/_migrated/content_uploads/KLIPPEL_Sound_Radiation_Poster_01.pdf
LoudSoft Updates FINECone 2020 Simulation Software | audioXpress
FINECone Demo - YouTube
Speaker Cones — Fabrication, Materials, and Performance | audioXpress
https://www.klippel.de/fileadmin/_migrated/content_uploads/KLIPPEL_Sound_Radiation_Poster_01.pdf
LoudSoft Updates FINECone 2020 Simulation Software | audioXpress
FINECone Demo - YouTube
I remember reading it somewhere, perhaps in a paper. The closest I could find is a section in this book which is describing hypothetical dome diaphragm behavior. The same principle would apply to cones.
"High Performance Loudspeakers" by Martin Colloms, 7th edition, 2018, John Wiley & Sons Ltd. Page 88.
For normal practical cones, this consideration is pretty esoteric: almost all cones will reach first resonance breakup before reaching the cancellation null. Even the SB17CAC35, which for a 6.5 inch driver has an amazingly high first mode of 7 kHz, the cancellation null is around 6.5 kHz (based on my estimate of cone depth).
Attachments
Thanks ! I remember seeing graphics very similar to the Klippel documentation in some paper or book in the distant past...
I can offer you something better: frequency response curves *showing* it 😀
Not often clearly seen in the Hi Fi World because speaker cones are either too thick, which dampens it, are crossed over below that critical frequency , or both, BUT an unavoidable fact in Guitar speakers, where cones are very thin *and* are used "full range" , meaning no crossovers or tweeters.
*All* 12" Guitar speakers, no matter which brand, type, "American" or "English", old or new, show the same distinctive 1200-1500 something Hz dip caused by flexible "wave", I am not talking acoustic "air" wave but the flexible whip type one reaching cone edge and being reflected back towards voice coil causing a deep notch.
Edge impregnation with a thick flexible paste which never hardens dampens the effect somewhat (it´s the difference between doped and undoped cones) but never kills it:
Celestion G12T75:
Jensen C12N
Eminence Legend 1258
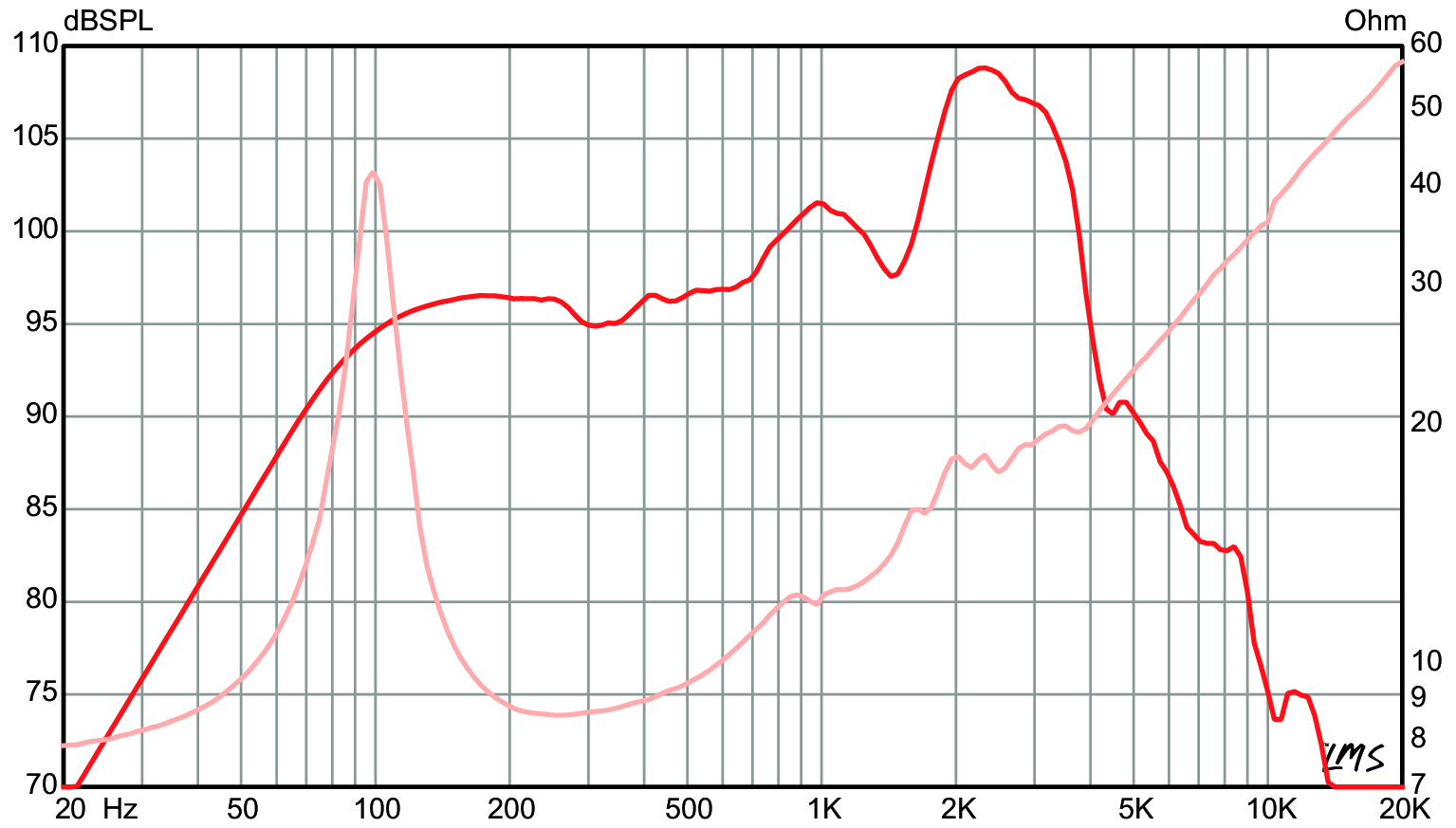
1950's style (Jensen P12N) undoped, underdamped/high Q (weakish Alnico magnet), paper thin cone, showing that dip in all its glory:
Another source here: David Ralph's Speaker Pages - Projects
Go down to the section titled "A Phase-Shield Primer".
So what would happen with an elliptical speaker, would the difference between the path of the wave on the long side cancel each other out? I guess two different sized drivers could have opposing peaks and dips, but would beam - perhaps right at the 2,000 to 3,000 Hz hump shown in the frequency graphs of the guitar/ pa drivers.
These matters are the concern when designing a phase plug for a cone driven waveguide (sorry I didn't pick that up yesterday).
I'd add then, when this matters it can lead to higher order modes in the waveguide, diffractions and internal reflections as the wavefront isn't in step with itself or with the waveguide.
As to what this can do to the sound there are now axial inconsistencies and secondary sources.
I'd add then, when this matters it can lead to higher order modes in the waveguide, diffractions and internal reflections as the wavefront isn't in step with itself or with the waveguide.
As to what this can do to the sound there are now axial inconsistencies and secondary sources.