Andrew,
Apart from core area also core mass / magnetic path length have (minor) influence.
For the smaller SU48 core you see that the b version not quite manages the higher power relative to the a version; here core mass is more important.
As the cores get bigger you see that core mass gets a bit more important; the SU90b yields more power than the SU90a proportional to core area.
However for the bigger cores the core area / power relationship stays within 2%; I call this very linear for practical purposes.
Apart from core area also core mass / magnetic path length have (minor) influence.
For the smaller SU48 core you see that the b version not quite manages the higher power relative to the a version; here core mass is more important.
As the cores get bigger you see that core mass gets a bit more important; the SU90b yields more power than the SU90a proportional to core area.
However for the bigger cores the core area / power relationship stays within 2%; I call this very linear for practical purposes.
Andrew,
OK, let's try it another way:
SU48b should yield 3,47/2,19 x 26,5 = 42 W; P = 44,5 W, so 6% "more" than SU48a.
SU60b should yield 5,3/3,5 x 75,4 = 114 W; P = 116 W, so a bittle less than 2% "more"than SU60a.
SU75b should yield 9,01/5,63 x 188 = 301 W; P = 297 W, so a bit over 1% "less"than SU75a.
SU90b should yield 13,4/7,99 x 370 = 620 W; P = 610 W, so less than 2% "less"than SU90a.
Conclusion: the smaller cores benefit from the shorter magnetic path length, i.e. with the same path length doubling of core area yields a bit more than double the power. For the big cores (about 300W and bigger) the longer magnetic path length goes at the cost of power. A core of over 2000 W comes 4% "short" comparing different core areas.
Please note that these values are for this particular grade of silicon steel and core geometry (c-cores).
Don't you agree that for practical purposes the relationship can be regarded being linear??
OK, let's try it another way:
SU48b should yield 3,47/2,19 x 26,5 = 42 W; P = 44,5 W, so 6% "more" than SU48a.
SU60b should yield 5,3/3,5 x 75,4 = 114 W; P = 116 W, so a bittle less than 2% "more"than SU60a.
SU75b should yield 9,01/5,63 x 188 = 301 W; P = 297 W, so a bit over 1% "less"than SU75a.
SU90b should yield 13,4/7,99 x 370 = 620 W; P = 610 W, so less than 2% "less"than SU90a.
Conclusion: the smaller cores benefit from the shorter magnetic path length, i.e. with the same path length doubling of core area yields a bit more than double the power. For the big cores (about 300W and bigger) the longer magnetic path length goes at the cost of power. A core of over 2000 W comes 4% "short" comparing different core areas.
Please note that these values are for this particular grade of silicon steel and core geometry (c-cores).
Don't you agree that for practical purposes the relationship can be regarded being linear??
looking at the datasheets on toroid dot com, i make the following observations.
va/s per pound of transformer (includes copper, as they didn't specify core size)
increased from 50 to 60 va/lb, from 150 watts to 1000.
At the same time, full load temperature rise dropped from 70C to 50C.
the surface area of the transformers only increased from 47 square inches to 130 (surface area calculated as a cylinder, not as a toroid)
thus the watts per square inch of surface area increased from 3.5 w/in^2 to 7.5 w/in^2
rated Va's per watt of core loss didn't have a trend, but varied between 160 and 290.
had the smaller transformers been derated to keep the same temperature rise, watts per pound would have climbed much faster.
the actual core loss was insignificant, at about 1 watt per kilogram of iron.
the 1 kva transformer had 5.3 watts core loss, and weighs a total of 17 pounds.
va/s per pound of transformer (includes copper, as they didn't specify core size)
increased from 50 to 60 va/lb, from 150 watts to 1000.
At the same time, full load temperature rise dropped from 70C to 50C.
the surface area of the transformers only increased from 47 square inches to 130 (surface area calculated as a cylinder, not as a toroid)
thus the watts per square inch of surface area increased from 3.5 w/in^2 to 7.5 w/in^2
rated Va's per watt of core loss didn't have a trend, but varied between 160 and 290.
had the smaller transformers been derated to keep the same temperature rise, watts per pound would have climbed much faster.
the actual core loss was insignificant, at about 1 watt per kilogram of iron.
the 1 kva transformer had 5.3 watts core loss, and weighs a total of 17 pounds.
Jo,
could you refer me to the data.
I am trying to understand what you have observed and I can't reach any conclusions because too much is missing.
However I leave a couple of thoughts.
If you double the size of a transformer it's volume goes up by a factor of 8. By similarity the weight should also go up by a factor of 8.
The smallest transformer showing xVA @ yLBS
The largest transformer showing cVA @ dLBS.
Take the cube root of the weight. Square that root.
Now calculate VA/cuberoot(weight) and calculate VA/ [cuberoot(weight)] ^2
Do either of these show a linear relationship?
could you refer me to the data.
I am trying to understand what you have observed and I can't reach any conclusions because too much is missing.
However I leave a couple of thoughts.
If you double the size of a transformer it's volume goes up by a factor of 8. By similarity the weight should also go up by a factor of 8.
The smallest transformer showing xVA @ yLBS
The largest transformer showing cVA @ dLBS.
Take the cube root of the weight. Square that root.
Now calculate VA/cuberoot(weight) and calculate VA/ [cuberoot(weight)] ^2
Do either of these show a linear relationship?
AndrewT, I have not been following closely, just wanted to interject, double the core material, double the power, ignoring wire, surface area, blah blah. You got that far by now right?
You may find through a lot of research that there is Tons of fudge in the world of transformer design unless you want to go all the way back to Maxwell's equations and spend all day calculating a transformer that doesn't actually do what was calculated because a bunch of factors' tolerance stacks up to be well over 10% off.
You may find through a lot of research that there is Tons of fudge in the world of transformer design unless you want to go all the way back to Maxwell's equations and spend all day calculating a transformer that doesn't actually do what was calculated because a bunch of factors' tolerance stacks up to be well over 10% off.
Last edited:
here is a program that will assist you in finding out primary turns of power transformers:
Silvio Klaic's electronic section
i have used it with much success, it gives you a good idea how to make your own traffos..
Silvio Klaic's electronic section
i have used it with much success, it gives you a good idea how to make your own traffos..
The J & P transformer book: a ... - Google Books
there's a short history in there, about 15 pages long.
pete millete's on-line books: they are free to download,
Technical books online
another very helpful site:
http://www.turneraudio.com.au/powertranschokes.htm
my work:
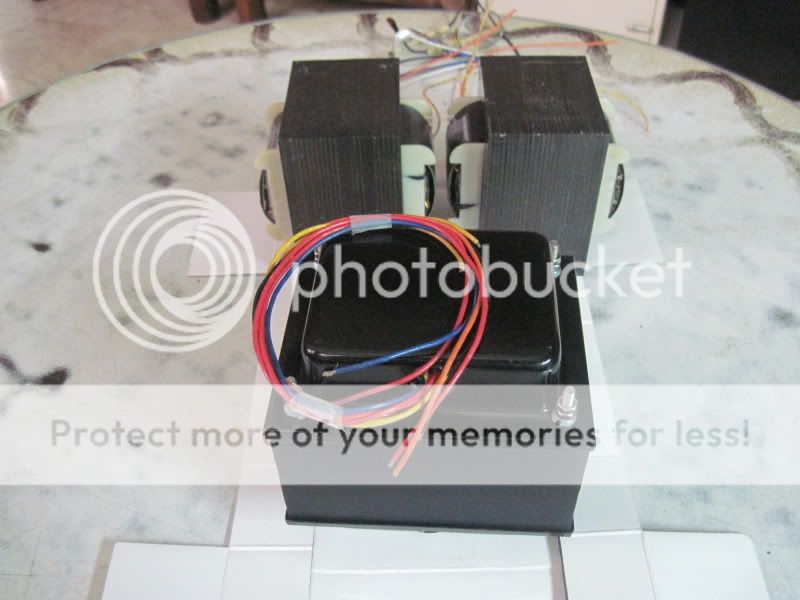
this is a plate traffo for my upcoming tube amp, it has 1 3/4 in x 3 inch core on a DEECO bobbin, endbells from FETRONICS:
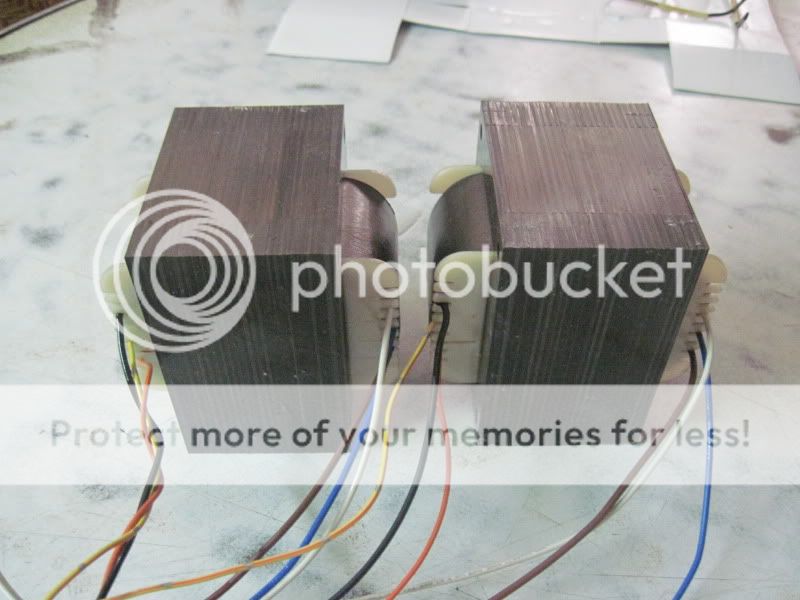
the 2 smaller traffos are OPT's , 3k plate to plate to 4/8 ohm secondaries:
Technical books online
another very helpful site:
http://www.turneraudio.com.au/powertranschokes.htm
my work:
this is a plate traffo for my upcoming tube amp, it has 1 3/4 in x 3 inch core on a DEECO bobbin, endbells from FETRONICS:
the 2 smaller traffos are OPT's , 3k plate to plate to 4/8 ohm secondaries:
Last edited:
- Status
- This old topic is closed. If you want to reopen this topic, contact a moderator using the "Report Post" button.