SPL function of speed or acceleration

[JohnR]

Re: Re: SPL function of speed or acceleration

2.) A speaker cone is driven by a triangle wave signal and the cone follows the signal perfectly.

But it doesn't. The force on the cone (and hence the acceleration) is proportional to the current through the voice coil.

 

[DanWiggins]

According to JE Benson, who was Richard Small's (of Thiele/Small renown) PHD thesis advisor, in his book "Theory and Design of Loudspeaker Enclosures":

p(s) = eg(s)*rho*Sd*ad(s)/(4*pi*r)

p(s) is pressure
eg(s) is generator voltage
ad(s) is diaphragm acceleration
r is distance from the speaker
rho is air density
Sd is diaphragm area

SPL = 20*log10(p(s)/pref)

I think that's pretty conclusive - SPL is a function of acceleration.

Thanks Ron!

Dan Wiggins
Adire Audio

 

[SY]

The SPL is related to many factors, but it is the cone velocity that directly causes the particle displacement and therefore SPL at a given point.

Rodd, here's my goofy way of looking at it. SPL is a pressure function. Pressure is force per unit area. Force is mass times acceleration.

 

[roddyama]

Re: Re: Re: SPL function of speed or acceleration

Roddyama: I think you are just repeating yourself and not considering what we say. Could you please do?

It only seems like I haven't read your post because I am repeating myself.😉 I'm repeating myself because I don't believe I'm making my point very well because you still believe SPL to dependent on the magnitude of the cones acceleration.🙂

If mounted in a box it is proportional to the acceleration.

The pressure from a point source is:

p=(U*rho0*w)/(4*pi*r)

where U is the volume flow (m3/s), rho0 is the density of air (=1.2 m3/s), w is the angular frequency (rad/s), r is the distance. Free field conditions are assumed. For low frequencies, a loudspeaker can be approcimated by a point source, and volume flow U is:

U=Sd*v

where Sd is the equivalent piston area of the speaker, and v is the velocity of the cone.

This is the same equation Ron uses which was used by Beraneck, Theile, Benson, Small and other. This equation is the special case of sinusodal pressure from a loudspeaker driven by an amplifier. Olson sites the more general case in, Music, Physics, and Engineering :

The relation between sound pressure and particle velocity is given by
P = pcu
where
P = sound pressure in dynes per square cm
p = density of air, in grams per square cm
c = velocity of sound, cm per second
u = particle velocity, in cm per second
...
The intensity, in ergs per second per square cm, of a plane sound wave is
I = (P^2)/pc = pu = pcu^2
where
P = sound pressure in dynes per square cm
p = density of air, in grams per square cm
c = velocity of sound, cm per second
u = particle velocity, in cm per second

As can be seen in the first equation, sound pressure is directly proportional to particle velocity. This can be seen again in the second equation for intensity or SPL.

So p is oproportional to w*v which in turn is equal to the acceleration (with a 90 degree phase shift).

p is proportional to w and v and their product, not acceleration.

But it doesn't. The force on the cone (and hence the acceleration) is proportional to the current through the voice coil.

This was a poor attempt on my part to indicate a cone with linear motion (constant velocity) in a purely conceptual situation.

 

[SY]

This equation is the special case of sinusodal pressure from a loudspeaker driven by an amplifier.
(snip)
p is proportional to w and v and their product, not acceleration.

OK, that's interesting. Now if something is true for a sinusoid of any arbitrary frequency, shouldn't it be then true for any waveform (Fourier decomposition)?

And if that's true, isn't acceleration then proportional to the product of cone velocity and frequency?

Let's not conflate particle velocity with cone velocity.

 

[roddyama]

So if we go back to the original question:

Hello Mathematicians out there.

Is Sound Pressure Level generated by a transducer proportional to speed of the cone or acceleration of the cone.

F

This is a case of sinusodal sound generation. Also the wording used is "proportional".

OK, that's interesting. Now if something is true for a sinusoid of any arbitrary frequency, shouldn't it be then true for any waveform (Fourier decomposition)?

And if that's true, isn't acceleration then proportional to the product of cone velocity and frequency?

So I would concede, given the original question that SPL will be "proportional" to acceleration and velocity.

Let's not conflate particle velocity with cone velocity.

particle velocity should be the same as cone velocity minus the effects of the radiation resistance.

 

[Svante]

Re: Re: Re: Re: SPL function of speed or acceleration

It only seems like I haven't read your post because I am repeating myself.😉 I'm repeating myself because I don't believe I'm making my point very well because you still believe SPL to dependent on the magnitude of the cones acceleration.🙂

This is the same equation Ron uses which was used by Beraneck, Theile, Benson, Small and other. This equation is the special case of sinusodal pressure from a loudspeaker driven by an amplifier. Olson sites the more general case in, Music, Physics, and Engineering :

As can be seen in the first equation, sound pressure is directly proportional to particle velocity. This can be seen again in the second equation for intensity or SPL.

p is proportional to w and v and their product, not acceleration.

This was a poor attempt on my part to indicate a cone with linear motion (constant velocity) in a purely conceptual situation.

Ah, so now I see where you got it from! It is true that sound pressure is proportional to particle velocity in a plane wave.
A plane wave is something that occurs at a far distance from a source. This particle velocity (at far distance) is something completely different from the velocity of the membrane. It is true that the particle velocity next to the cone is identical to the cone velocity, but there the equation for the plane wave is not valid since it is not far from the source.

Second, the equations used above are not specific for sinusoidal oscillation. Since any waveform can be built from several sinusoids, any waveform can be treated by these equations.

Me stating that p ~ w*v is proof of that p ~ a might need an explanation. I left out the sinusoidal part, the more complete description would be:

v=sin(w*t) gives a = d/dt sin(w*t) = w*cos(w*t) =w*sin(w*t+90 degrees)={ignoring the phase}=w*v

ie the description implied sinusoidal waveform (As most equations containing an w)

 

[SY]

Sines Of The Times, or, Off On A Tangent

Svante, sometimes it's easier to see this when you represent the wave as an exponential- that way, the proportionality and the phase come flopping out immediately when you differentiate. Completely mathematically equivalent to what you're saying, just a different way to represent it.

 

[Pjotr]

Just my :

Anyone can notice from al those calculations around to calculate speaker boxes that cone displacement lowers at 12 dB/okt at rising frequency and cone velocity lowers 6 dB/okt …

Displacement is the integral of speed over time en what is speed? Yes the integral of acceleration over time …

I am not going to do the math here but for the ideal case where a rigid moving piston with negligible diameter compared to the wavelength (thus radiating into a sphere) you will end up with constant acceleration for constant SPL. No need for Fourier here and a sinusoid is just a special case 😉

Beraneck wasn't that crazy 😀

Cheers 😉

 

[Svante]

Re: Sines Of The Times, or, Off On A Tangent

Svante, sometimes it's easier to see this when you represent the wave as an exponential- that way, the proportionality and the phase come flopping out immediately when you differentiate. Completely mathematically equivalent to what you're saying, just a different way to represent it.

Yes, sure, I do that all the time, but when I am uncertain of the "audience" I try to take it back to sines and cosines.

cos (wt) = re[exp(jwt)]
sin (wt) =im[exp(jwt)]

so if v=exp(jwt) then a = d/dt v =jw*exp(jwt) = jw * v

where the j automagically takes care of the phase shift of 90 degrees.

But that way of thinking requires complex math (which is easier once you've got used to it), which is usually learned at a later stage.

 

[Svante]

Just my :

Anyone can notice from al those calculations around to calculate speaker boxes that cone displacement lowers at 12 dB/okt at rising frequency and cone velocity lowers 6 dB/okt …

Displacement is the integral of speed over time en what is speed? Yes the integral of acceleration over time …

Right, and acceleration is 0 dB/octave. A differentiation tilts the spectrum by 6 dB/octave. That is the result of the w popping out in the differentiation of the sin(wt).

Beraneck wasn't that crazy 😀

He still isn't. 😉

 

[Svante]

According to JE Benson, who was Richard Small's (of Thiele/Small renown) PHD thesis advisor, in his book "Theory and Design of Loudspeaker Enclosures":

p(s) = eg(s)*rho*Sd*ad(s)/(4*pi*r)

p(s) is pressure
eg(s) is generator voltage
ad(s) is diaphragm acceleration
r is distance from the speaker
rho is air density
Sd is diaphragm area

SPL = 20*log10(p(s)/pref)

There is something wrong with this equation, I think the eg(s) should be left out.

A dimension analysis sais that N/m2 should be equal to

Volt*kg/m3*m2*m/s2* 1/m =Volt * kg/(m*s2) = Volt * kg*m/s2 / m2 =Volt * N / m2

 

[Ron E]

You are right. I was waffling between posting equations 67 and 68 and I forgot to take the eg out of the equation....

p(s) = rho*Sd*ad(s)/(4*pi*r)

or

p(s) = eg(s)*rho*Sd/(4*pi*r) * Bl/(Re*Mdt) *G(s)

Where G(s) is the transfer function.

 

[454Casull]

*head explodes*

 

[tool49]

I was just about to call my therapist and tell her that I was now fine and that we didn't need anymore sessions, but after reading this thread, I think I will persist in therapy!

 

[gary f]

Well, this one was fun to read!

Thanks to all
F

 

[roddyama]

Hello Mathematicians out there.

Is Sound Pressure Level generated by a transducer proportional to speed of the cone or acceleration of the cone.

F

DUH!! It only took me 10 years to come up with my argument here😀

The answer to the question is the SPL generated by a transducer is proportional to both cone speed and acceleration.

This is true in the special case of a transducer (speaker) cone with sinusoidal motion because the derivative of sine is cosine which are proportional to each other.

However, in the more general case of non sinusoidal sound generation we find that the pressure in SPL is created by, and therefore proportional to particle velocity as I quoted from Olsen in post 24.

 

[david crewe]

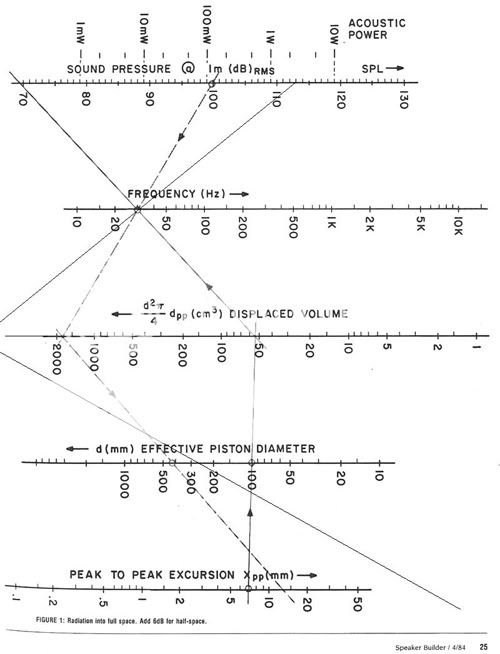
Pictures, pictures, pictures,
Seems to me that SPL is based on three things. Piston diameter, p-p excursion, and frequency. Speed and acceleration are mute until such point that sufficient acceleration can not be achieved (undershoot), or force (m*a) becomes excessive (overshoot). Other than that they are just components that are dependent on the applicable sin waves.
Just sayin,
David

 

[Ron E]

DUH!! It only took me 10 years to come up with my argument here😀

The answer to the question is the SPL generated by a transducer is proportional to both cone speed and acceleration.

This is true in the special case of a transducer (speaker) cone with sinusoidal motion because the derivative of sine is cosine which are proportional to each other.

However, in the more general case of non sinusoidal sound generation we find that the pressure in SPL is created by, and therefore proportional to particle velocity as I quoted from Olsen in post 24.

All sound is made of sines and cosines, so your last paragraph is wrong.
If you ignore phase, acceleration is just velocity times angular frequency. I wouldn't call this "proportional" in the general sense. By your argument, you could also say that spl is proportional to displacement, but then there is another angular frequency factor to multiply in...

Certainly if you ignore frequency, you get more SPL if you have more displacement, velocity or acceleration, but which one has a simple constant of proportionality, such that a doubling of it gives a doubling of sound pressure? That would be acceleration.....

 

[roddyama]

All sound is made of sines and cosines, so your last paragraph is wrong.

Hi Ron,

You've been well I hope.

Actually no, sound is not made up of sines and cosines. Us humans devised a way to mathematically (transforms, Fourier in this case) describe sound using sines and cosines.

The proportionality of SPL to speed or acceleration is as I quoted Olsen. It is proportional to speed (particle velocity) and to acceleration as so far as one wishes to describe sound in terms of sines and cosines.