Yeah, I know that... but that's.... EASY.... and I don't get to play with the amps.
My idea is to get away with the least amount of output resistance, hopefully to get it less than with a bridging configuration.
I think I'll go buy some resistors tomorrow.
OK... Two Amplifier Channels. Double The Current when in Parallel.
As opposed to Two Amplifier Channels. Double the Voltage when Bridged.
Either way I'm quadrupling the voltage... subject to the limits of the power supply and heat sink.
Is that so hard to understand? And it's late here.
V=IR, P=V^2/R. You haven't changed V or R, so I and P are unchanged. You can't force more current through a given impedance with a given voltage than Ohm's law states.
And no you are not quadrupling the voltage. You are doubling it when bridging, and leaving it unchanged when in parallel.
This is rather basic.
And no you are not quadrupling the voltage. You are doubling it when bridging, and leaving it unchanged when in parallel.
This is rather basic.
Last edited:
Is Ohm's law so hard to understand?
Go ahead, you'll learn from experimenting, though I guarantee that the laws of physics predict that you'll neither get the extra power you need, nor the damping factor you desire. You won't find anyone here disagreeing with that, so please post your results when you've done the wiring and the measurements.
OK, folks.... let's get something straight. I am doubling the current.
Anyhow, so the question for me now is what is the minimum load I have to put in series to prevent the differences from either channel to drive the other?
Given that I plan on paralleling two channels of a stereo amp, hence getting a theoretical perfect match, practically the best possible match.
Given that I can calculate the output impedance based on the load ( 400 at 8 ohms -> 0.02 ohms..)... which is ( 6 ohms / 0.02 ohm -> 300 ) and so on. (*),
Then, it would seem like adding to the load will actually decrease the damping factor. Unfortunately this also means that I will dissipate heat on the additional load.
So, my guess is that if I can find a .1 ohm resistor, it might be enough ( 6.1 / 0.02 -> 305 ) damping factor and only use up (assuming 40 watts per channel amp... that implies about 0.656 watts ). So, if I can get a 0.1 ohm, 1 watt resistor I ought to be fine, not burn too much power and affect the damping too much).
Or course, I need to figure out a couple more things, but that can wait until mañana.
Good night. Thanks.
(*) Interestingly, this means that the higher the load, the higher the damping factor. Is this why my 16 ohm Acoustic Energy AE1 have such a tight bass?
Anyhow, so the question for me now is what is the minimum load I have to put in series to prevent the differences from either channel to drive the other?
Given that I plan on paralleling two channels of a stereo amp, hence getting a theoretical perfect match, practically the best possible match.
Given that I can calculate the output impedance based on the load ( 400 at 8 ohms -> 0.02 ohms..)... which is ( 6 ohms / 0.02 ohm -> 300 ) and so on. (*),
Then, it would seem like adding to the load will actually decrease the damping factor. Unfortunately this also means that I will dissipate heat on the additional load.
So, my guess is that if I can find a .1 ohm resistor, it might be enough ( 6.1 / 0.02 -> 305 ) damping factor and only use up (assuming 40 watts per channel amp... that implies about 0.656 watts ). So, if I can get a 0.1 ohm, 1 watt resistor I ought to be fine, not burn too much power and affect the damping too much).
Or course, I need to figure out a couple more things, but that can wait until mañana.
Good night. Thanks.
(*) Interestingly, this means that the higher the load, the higher the damping factor. Is this why my 16 ohm Acoustic Energy AE1 have such a tight bass?
Last edited:
Essentially, you have three constraints that can limit the amplifier output signal level:
A. |vout| <= Vout, max
B. |iout| <= Iout, max
C. vout = iout ZLS
You are changing the second constraint, but for ZLS = 6 ohm, it's actually the first and third constraints that limit the output signal level.
A. |vout| <= Vout, max
B. |iout| <= Iout, max
C. vout = iout ZLS
You are changing the second constraint, but for ZLS = 6 ohm, it's actually the first and third constraints that limit the output signal level.
It's the available current you're doubling, not the delivered current. In order to double the delivered current you need to either put two speakers in parallel, or increase the voltage. And if you've got two speakers to put in parallel you're better off driving each one from its own amp, thereby eradicating the need for series resistors and knackered damping factor.
It's very late. Go to bed.
Have another think about I=V/R in the morning...I learned this in year 7 physics. 50 years later I haven't seen Ohms Law disproven.
It's very late. Go to bed.
Have another think about I=V/R in the morning...I learned this in year 7 physics. 50 years later I haven't seen Ohms Law disproven.
so lets say you have 10Vrms at some signal into your speaker. You place your beloved parallel amps together... and still get 10Vrms into the same load... how is that doubling any current?
you only get some benefit at near max output power, where the output stage starts to struggle... or do you? will the voltage rails struggle and not be able to keep up? perhaps it is the drop in voltage rail already limiting the max power even with one singla channel... hmmm
you only get some benefit at near max output power, where the output stage starts to struggle... or do you? will the voltage rails struggle and not be able to keep up? perhaps it is the drop in voltage rail already limiting the max power even with one singla channel... hmmm
There is another thing.... I'm doubling the number of amplifiers.
It's like this... take a look at a big amplifier, look at all of those output devices, they are all in parallel.
Similar thing...
No. You are doubling the available current. You cannot double the current into an impedance without doubling the voltage or halving the impedance. You can stack up as many power amplifiers in parallel as you like, but you cannot change Ohm's law.
The question is whether you are going to realize your objective by paralleling the amplifiers, and you won't unless you change speakers.
Or else you need to bridge.
How many times does all this need to be stated?
It isn't like this. Take a look at the voltage rails. They are higher. More voltage. That dictates the need for more current capacity, and that dictates the need for more power devices.
No it isn't.
You are arguing with the laws of electricity.
Each channel sees the same load.
Actually, it sees the load PLUS the load from the other channel.
Hopefully if the channels are operating alike, they offset each other and it works. The point of the additional output resistors is to ensure that any offsets are taken care of. Being a stereo amp, you'd figure they have the same gain characteristics and the inter channel differences are minor.
So in a parallel configuration each channel sees the same load. Unlike in bridging where each channel sees HALF the load.
The total current into the load is the sum of the current from both channels.
It's actually very early.
Once again, Ohm's law is not the only one at play here. You got to take into account the equations for power and the electronic equations for the circuit.
It's my amps.... it's my speakers.... I'm gonna get my resistors, just in case I will have a fire extinguisher.
In any event, I'm gonna get this thing cranked to 11.
Running our of power supply juice on just one channel? That's a non sequitur... come on. It's not like I'm gonna drive this to 12... only 11.
Also look at the high rail voltages, they are used because of constraint A from post #26.
Bridging also tackles constraint A. Bridging and paralleling would tackle constraints A and B.
Actually there is only one load to see. The speaker load. If there is now more load magically appearing from somewhere, there will be even less current, by Ohm's law, not that this 'PLUS' made any sense in the first place. Unless you're talking about the other channel's 0.2 ohm output impedance, which is neither here nor there.
So now you're contradicting yourself.
The total current into the load is determined by I=V/R, and it is not dependent on the number of parelleled channels.
They all say the same thing, as everyone has been telling you. V=IR, I=V/R, P=V^2/R, P=I^2R.
You have a fundamental misunderstanding.
"Ohm's law is not the only one at play here"
Ohm's law trumps all.
Ohm's law trumps all.
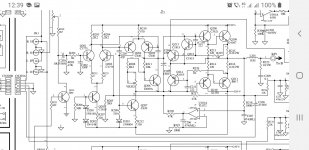
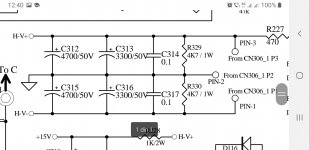
Judging by the internal schematic of Parasound Z3, if I had 4 of them and no possibility to upgrade to a biamp system, I'd do a bold move : change the symmetrical supply to single supply(simply use 2 rectifier diodes of the bridge and no negative) , bias the amp's input at 1/2 v supply, change the input capacitors to nonpolar ones(that depends on the type of bridge type of drive) , probably dump the dc offset U101 or work around r219 refference .... and make it bridged .
That will get you almost the same theoretical output power, but better thermal management and transient power delivery in the low register, also colder amps mean less offset so less need for dc offset compensation. You could even make use of a higher bias approaching class A operation so less crossover distortions if carefully biased . Input stage slew rate doubles, but the final transistor Early effect worsens so you don't really get better speed unless you change the final transistors with faster ones ,but that's gonna be rather difficult to do as 2sa1047 with just 20Mhz ft actually has 120pF BC capacitance, 50pf less than 2sc5248...so the final stage is already pretty fast at lower supply voltages making it good for lower supply bridged operation.
That will get you almost the same theoretical output power, but better thermal management and transient power delivery in the low register, also colder amps mean less offset so less need for dc offset compensation. You could even make use of a higher bias approaching class A operation so less crossover distortions if carefully biased . Input stage slew rate doubles, but the final transistor Early effect worsens so you don't really get better speed unless you change the final transistors with faster ones ,but that's gonna be rather difficult to do as 2sa1047 with just 20Mhz ft actually has 120pF BC capacitance, 50pf less than 2sc5248...so the final stage is already pretty fast at lower supply voltages making it good for lower supply bridged operation.
Attachments
Last edited:
Well here we have Ohm's law and Tony's law... Laugh my *** off
Here is how Quad do it with the 405
https://www.dadaelectronics.eu/uplo...Documents/Quad-405-Monoblock-Instructions.pdf
This allows a doubling of power but only if you half the impedance
Stuart
https://www.dadaelectronics.eu/uplo...Documents/Quad-405-Monoblock-Instructions.pdf
This allows a doubling of power but only if you half the impedance
Stuart
Tony,
I think that all of the members who have replied to your posts understand where you are coming from and some have provided very good explanations as to why you will not get the results that you desire. You seem to be, as yet, unconvinced. Let me see if I can help.
In your defense, if you are successful in coming up with a current sharing scheme for two (or more) amplifiers without incurring too much loss in the additional resistors, you may be able to eke out a small increase in speaker volume. This is because each amplifier will be supplying less current and its internal power supply rails will sag less under load. Thus, the amplifiers will be able to swing a somewhat higher output voltage which is necessary to supply additional current to the speaker. How much additional voltage swing can be obtained is dependent on how stiff the power supplies are. I suspect the increase would be inaudible.
The point that you seem to be missing is that you cannot pick which "laws" of physics that you want to apply. You must satisfy all at the same time. Ohms law (V = R*I) is, perhaps, the most fundamental equation in electrical engineering. In order to force additional current into a fixed resistive load, you must apply additional voltage across its terminals. There is no way around this.
I hope this helps,
Bruce
I think that all of the members who have replied to your posts understand where you are coming from and some have provided very good explanations as to why you will not get the results that you desire. You seem to be, as yet, unconvinced. Let me see if I can help.
In your defense, if you are successful in coming up with a current sharing scheme for two (or more) amplifiers without incurring too much loss in the additional resistors, you may be able to eke out a small increase in speaker volume. This is because each amplifier will be supplying less current and its internal power supply rails will sag less under load. Thus, the amplifiers will be able to swing a somewhat higher output voltage which is necessary to supply additional current to the speaker. How much additional voltage swing can be obtained is dependent on how stiff the power supplies are. I suspect the increase would be inaudible.
The point that you seem to be missing is that you cannot pick which "laws" of physics that you want to apply. You must satisfy all at the same time. Ohms law (V = R*I) is, perhaps, the most fundamental equation in electrical engineering. In order to force additional current into a fixed resistive load, you must apply additional voltage across its terminals. There is no way around this.
I hope this helps,
Bruce
- Status
- Not open for further replies.