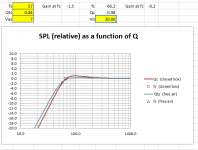
Say I have a woofer and its Vas is 7L, Qts is 0.84. If it is put in a 20L sealed enclosure will the total speaker Q be just slightly over 0.84?
For a sealed box,
Qc = Qts * sqrt[(Vas/Vb) + 1]
For the above case:
= (0.84) * sqrt[(7/20) + 1]
= 0.98
Qc = Qts * sqrt[(Vas/Vb) + 1]
For the above case:
= (0.84) * sqrt[(7/20) + 1]
= 0.98
Very nice, thanks for the formula! I'll add it to my book of good things lol.
Do you know off hand, if the driver's free air resonance was 57Hz, what it'd be in that 20L box? Or does that require multiple TS parameters?
(I don't have many of them, and I could measure current while slowly sweeping between 30 and 80Hz if it's complex - I'm asking is for EQ purposes - I can't bring and bury my speaker flush with the ground in a field (for frequency response measurement) for a bit of time now, I'm looking for a rational starting point. The purpose of this EQ is to be able to enable lowering the overall frequency response by exactly one octave, keeping the speaker's Q the same. Actually, with the bump being in the 35-55Hz range instead of the 70-110Hz, the extra dB might not be ideal.. If it's not, I'd probably like to deal with that using a separate peaking filter to pull down the slight bump probably centered on 45Hz (boomy))
Do you know off hand, if the driver's free air resonance was 57Hz, what it'd be in that 20L box? Or does that require multiple TS parameters?
(I don't have many of them, and I could measure current while slowly sweeping between 30 and 80Hz if it's complex - I'm asking is for EQ purposes - I can't bring and bury my speaker flush with the ground in a field (for frequency response measurement) for a bit of time now, I'm looking for a rational starting point. The purpose of this EQ is to be able to enable lowering the overall frequency response by exactly one octave, keeping the speaker's Q the same. Actually, with the bump being in the 35-55Hz range instead of the 70-110Hz, the extra dB might not be ideal.. If it's not, I'd probably like to deal with that using a separate peaking filter to pull down the slight bump probably centered on 45Hz (boomy))
Replace Qts with Fs, and Qc with Fc in the formula in post 2.
Fc will be about 66 Hz.
Fc will be about 66 Hz.