I heard from a discussion about driver measurements. The topic was about generating xover filters, and about the best compromise measuring distance. I consider this an interesting playfield, and this motivated me to perform some simulations. It’s all about anechoic, direct sound and the influence of the measuring distance on the baffle diffraction step (BDS).
Assume a linear monopole point source on a circular baffle. When the pressure wave reaches the rim of a baffle, its’ room geometry changes from half-space to full-space. This is a change by a factor of 2.
In the time domain, this change theoretically will cause a negative impulse. As the room geometry changes by a factor of 2, the magnitude of this negative impulse might be half the amplitude of the initial positive impulse. In the frequency domain the response will show the well-known BDS and some comb filtering towards higher frequencies with a first minimum corresponding to the baffle width. This is widespread common knowledge.
Most models and graphs for the BDS assume an observation point in infinity. For the infinity case, in time domain the negative impulse occurs after the time needed for the soundwave to propagate from the centrepoint of the baffle to the periphery.
Instead, theoretically approaching the observation point to null distance from the baffle plane into it’s centre will double this time: The sound has to travel to the periphery first, and then return to the centre where it gets assessed. In time domain the negative impulse occurs delayed by a factor of 2 compared to the one assessed at Infinity. In frequency domain, this leads to a combined squeeze/shift of the typical frequency response along the frequency scale. The first minimum of the comb filtering pattern will occur one octave lower than in the infinite case.
In analogy to these both extreme cases, it becomes evident, that even without taking any room response (such as first reflections, echos and the like) into consideration, measuring at 1m and listening at 3m will not be the same in terms of frequency response. Therefore, relying to a measurement from 1m distance to optimize a set of xover-filters, and then later on listen to the result at 3m may lead to a mismatch between the filters and the actual frequency responses and thus to an awkward and unwanted experience.
Let’s do some simulations to explore this one. For the following graphs sampling rate was set to 192kHz. For the first three graphs the baffle diameter was assumed to be 34.3cm which corresponds to a wavelength corresponding to a frequency of 1kHz.
Curves show measurement distances of
green: 0, theoretical measure flush on the baffle's center, e.g. spot-on the point sound source
brown: 1 == 0.343 measuring distance (in baffle diameter length, 1 == 34.3cm)
blue: 2.9 == 1.0m measuring distance
grey: 8.7 == 3.0m measuring (=hearing) distance
black: Infinity theoretical measuring distance
Red individually shows the resulting magnitude difference between two test distances.
The time domain graph. The negative impulses of the baffle diffraction occur with different delays from the initial positive impulse:

Frequency domain: Red shows theoretical null distance vs. infinity BDS model distance

Frequency domain: Red shows the frequency response difference between 1m vs. 3m measuring distance:

Frequency domain: Difference 34.cm vs. 3m measuring distance … measuring very close is no good idea at all …

And now, what about a more narrow baffle at 17.15cm diameter, e.g. half the size of the former one?
Time domain for 1m vs. 3m measurement:

Same 17.15cm baffle, but in frequency range
Red 1m - 3m
Blue at 1m (5.8 times the baffle diameter)
Grey at 3m (17 times the baffle diameter )

Small baffles, as expected by geometry/trigonometry logic, show less severe shifts at the typical measuring/listening ranges of 1m/3m than large baffles. It’s evident, because relative to the smaller baffle width, the distance/bafflewidth quotient get bigger. By the way, this might be a contributing reason why baby baffles sound more pleasing at any listening distance than adult ones.
Caution: Take all these simulations all with a grain of salt. These graphs show a theoretical workout of the subject, highlighting a possible issue. In practice you will ideally not have to deal with central point sources on circular baffles. But the take-home message seems clear: Do not measure too close in a false idea to best possibly exclude room interferences. This will jam your measuring validity range. The closer you measure, the messier the result gets for more distant locations. Same goes not only for x-over filters, but also for whole speaker system measurements.
Assume a linear monopole point source on a circular baffle. When the pressure wave reaches the rim of a baffle, its’ room geometry changes from half-space to full-space. This is a change by a factor of 2.
In the time domain, this change theoretically will cause a negative impulse. As the room geometry changes by a factor of 2, the magnitude of this negative impulse might be half the amplitude of the initial positive impulse. In the frequency domain the response will show the well-known BDS and some comb filtering towards higher frequencies with a first minimum corresponding to the baffle width. This is widespread common knowledge.
Most models and graphs for the BDS assume an observation point in infinity. For the infinity case, in time domain the negative impulse occurs after the time needed for the soundwave to propagate from the centrepoint of the baffle to the periphery.
Instead, theoretically approaching the observation point to null distance from the baffle plane into it’s centre will double this time: The sound has to travel to the periphery first, and then return to the centre where it gets assessed. In time domain the negative impulse occurs delayed by a factor of 2 compared to the one assessed at Infinity. In frequency domain, this leads to a combined squeeze/shift of the typical frequency response along the frequency scale. The first minimum of the comb filtering pattern will occur one octave lower than in the infinite case.
In analogy to these both extreme cases, it becomes evident, that even without taking any room response (such as first reflections, echos and the like) into consideration, measuring at 1m and listening at 3m will not be the same in terms of frequency response. Therefore, relying to a measurement from 1m distance to optimize a set of xover-filters, and then later on listen to the result at 3m may lead to a mismatch between the filters and the actual frequency responses and thus to an awkward and unwanted experience.
Let’s do some simulations to explore this one. For the following graphs sampling rate was set to 192kHz. For the first three graphs the baffle diameter was assumed to be 34.3cm which corresponds to a wavelength corresponding to a frequency of 1kHz.
Curves show measurement distances of
green: 0, theoretical measure flush on the baffle's center, e.g. spot-on the point sound source
brown: 1 == 0.343 measuring distance (in baffle diameter length, 1 == 34.3cm)
blue: 2.9 == 1.0m measuring distance
grey: 8.7 == 3.0m measuring (=hearing) distance
black: Infinity theoretical measuring distance
Red individually shows the resulting magnitude difference between two test distances.
The time domain graph. The negative impulses of the baffle diffraction occur with different delays from the initial positive impulse:
Frequency domain: Red shows theoretical null distance vs. infinity BDS model distance
Frequency domain: Red shows the frequency response difference between 1m vs. 3m measuring distance:
Frequency domain: Difference 34.cm vs. 3m measuring distance … measuring very close is no good idea at all …
And now, what about a more narrow baffle at 17.15cm diameter, e.g. half the size of the former one?
Time domain for 1m vs. 3m measurement:
Same 17.15cm baffle, but in frequency range
Red 1m - 3m
Blue at 1m (5.8 times the baffle diameter)
Grey at 3m (17 times the baffle diameter )
Small baffles, as expected by geometry/trigonometry logic, show less severe shifts at the typical measuring/listening ranges of 1m/3m than large baffles. It’s evident, because relative to the smaller baffle width, the distance/bafflewidth quotient get bigger. By the way, this might be a contributing reason why baby baffles sound more pleasing at any listening distance than adult ones.
Caution: Take all these simulations all with a grain of salt. These graphs show a theoretical workout of the subject, highlighting a possible issue. In practice you will ideally not have to deal with central point sources on circular baffles. But the take-home message seems clear: Do not measure too close in a false idea to best possibly exclude room interferences. This will jam your measuring validity range. The closer you measure, the messier the result gets for more distant locations. Same goes not only for x-over filters, but also for whole speaker system measurements.
Last edited:
Yeah surprise! Last time I this I had issues trying to measure close as well. I ended up going out to 1 meter and it all worked out in the end.
Rob 🙂
Rob 🙂
Conclusion - mic distance should be at at least twice the baffle's shortest diameter (preferably 3xd)
Meanwhile I found out that you have also to consider the target frequency range of the driver. Even in my former example of the circular baffle of 34.3cm with a ratio of 1 everything is fine for frequencies < 1000Hz. In this restricted range, you have a delta only of some few 1.5dB between the measurements @ 34.3cm and 3m !
Look at the 2nd graph which matches your recommendation (2.9xd == 1m for the 34.3cm baffle) vs. 3m: As can be seen, for the lower frequency range below 1000Hz, the correlation between both measurement points, 1m and 3m, is perfctly fine with a delta < 0.5dB. It's above 1kHz that things get worse ... Between 2kHz and 3.5kHh, the delta grows to 5dB (+2dB @ 2kHz ... -3dB @ 3.5kHz) offset between the 1m and the 3m measuring distances. And at 10kHz the delta reaches 14dB (+-7dB) around 10kHz.
Along with the 17.15cm baffle, the delta is <0.5dB up to 3kHz, and a delta of 5dB is reached well above 10kHz. A delta of 14dB is reached above 40kHz. Therefore, the deeper you go with the target frequency range, the bigger you can build, and the closer you may go measuring. Let's get berserk and mount the point source into a 68.3cm diameter baffle instead into the theenywheeneone:
Even measuring relatively very closely at 1xd only == 68.6cm you will get a delta within 1.5dB for all frequencies < 500Hz. This is all the same as in the case of the 34.3cm baffle/measurement, but only scaled down by a factor of 2. Which seems logical.
This makes for an important addendum to my first post: Also consider the target frequency range in your simulations !!! The deeper your target frequency range, the closer you may measure without severe consequences for the homogenity of the results at the measuring and at the hearing location.
Last edited:
This makes for an important addendum to my first post: Also consider the target frequency range in your simulations !!! The deeper your target frequency range, the closer you may measure without severe consequences for the homogenity of the results at the measuring and at the hearing location.
Ok I found that to be the complete opposite. I tried in close on a 15' woofer and it just wasn't right. Really hard to explain but looking at "published" measurements it was out of family. It wasn't till I increased the distance I had confidence my measurements were "correct". Using the longer distance measurements I was able to get repeatable measurements I could not get in close,
Rob 🙂
I tried in close on a 15' woofer and it just wasn't right.
I really hope that was a 15" woofer. Well, if it was really 15', then I guess it just wasn't right after all. 😉
Ok I found that to be the complete opposite. I tried in close on a 15' woofer and it just wasn't right. Really hard to explain but looking at "published" measurements it was out of family. It wasn't till I increased the distance I had confidence my measurements were "correct". Using the longer distance measurements I was able to get repeatable measurements I could not get in close,
Rob 🙂
I think it's quite easy to explain what you observed.
1. At low frequencies you measure and hear a lot of room response which is not included in the modelling I presented. In general, any point in a room will show you it's individual and unique frequency response at low frequencies, depending on acoustical laws.
For a primer, have a look here.
2. Especially at low frequencies, you will have to deal with walls interaction changing the propagation impedance for a sound source and thus the resulting SPL in relation
- to placement (acoustically) near to walls
and
- to frequency.
And keep in mind that in an average room, you mostly will have to deal with 6 distinct walls.
So have fun and freely/wisely choose your very own, most confident "correct" measurement ...
Last edited:
Good sources of information
https://kimmosaunisto.net/ (manuals for taking measurements with different software)
https://www.artalabs.hr/support.htm
https://www.audioholics.com/loudspeaker-design/loudspeaker-measurement-standard
https://www.klippel.de/know-how/literature/standards.html
Important variables are
1. environment (preferably anechoic)
2. mic distance (2 meters can be considered standard for home hifi speakers, spl at 1m is standard too)
Nearfield (a few millimeters) is necessary to analyze a driver's performance in the box and farfield 3D (360deg) to see how the system works.
Since ideal conditions are often not possible we must use several methods and combine data, and recognize artefacts
https://kimmosaunisto.net/ (manuals for taking measurements with different software)
https://www.artalabs.hr/support.htm
https://www.audioholics.com/loudspeaker-design/loudspeaker-measurement-standard
https://www.klippel.de/know-how/literature/standards.html
Important variables are
1. environment (preferably anechoic)
2. mic distance (2 meters can be considered standard for home hifi speakers, spl at 1m is standard too)
Nearfield (a few millimeters) is necessary to analyze a driver's performance in the box and farfield 3D (360deg) to see how the system works.
Since ideal conditions are often not possible we must use several methods and combine data, and recognize artefacts
When you measure at only a few millimeters you may see response irregularities at the upper end of a driver's passband that are not acually there but caused by different path lengts between microphone and cone:

A part of the irregularities above 1 kHz may also be from diffraction but I guess a large part of it is caused by by path differences due to the close-up measuring (about 1 cm on a 8" woofer).
Regards
Charles
A part of the irregularities above 1 kHz may also be from diffraction but I guess a large part of it is caused by by path differences due to the close-up measuring (about 1 cm on a 8" woofer).
Regards
Charles
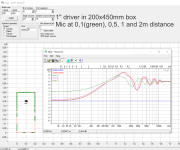
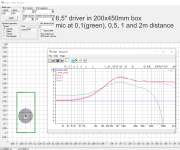
Driver size vs. baffle has remarkable effect to edge diffractions, which look different at varying mic distance too!
View attachment 1348051 View attachment 1348052 View attachment 1348053
This is an important fact in real-world speakers and for the higher frequency range!
It may contribute to the pleasant reproduction of small-sized baffles, where the width of the baffle roughly corresponds with the diameter of the driver(s). This also goes for dipole baffles, where in the high frequency range it is best to operate the drivers naked, or on a very slim baffle: https://linkwitzlab.com/models.htm#A3. And by the way, horn loudspeakers with baffleless horn mouths also might show no or very low BDS artefacts. In horns, the hopefully well-shaped horn mouth is quasi it's own baffle.
Therefore, it seems wise to minimize the baffle sizes to not substantially be larger than the largest speaker attached to it. And also, to gradually slim down the baffle to match the smaller structures of the the mid driver(s) and the tweeter (except if you use a large waveguide). Speaking of waveguides, you even might design a small waveguide for the tweeter, not so to even out polar response towards high frequencies, but to match the outer diameter of the tweeter itself at the mouth of the wavegide. This would also even out the dome diameter vs. the baffle width mismatch a bit.
Last edited:
I fail to understand the objective, also find some points in the op to be not supported by evidence. On the Arta site is a publication showing the effect of distances. Vituixcad baffle diff tool i use a lot also shows a phase effect, and shows very clearly the effect of measurement distance.
There is sufficient results published that shows that rounding helps in damping the diffraction effects correlated with measurements. The baffle step effect for rectangular shaped baffles is max about 7 dB at 3000mm in my experience and compared to 1000mm distance the difference is less than 1 dB lower at 50hz, and less than 1 dB higher at ~ 1000hz., and null dB at ~400hz.
Note i make a distinction between baffle step and baffle diffraction, because the effects are in different frequency ranges.
Baffle step in my Gaya2 case is mostly below 400 hertz, and a little bit above. Diffraction is upwards from ~700 hertz with a bump at frequency ~box width, followed by a dip etc. Shaping the baffle smoothens the diffraction effects, and in fact can be used to shape the driver response a bit.
Baffle step is a given. In my case at ~400 hertz the baffle step responses at 330mm 1000mm and 3000mm cross each other.
Measurements below ~ 400hertz at distances of more than very close is practical always compromised because of boundaries present, but combining measurements has shown to be close enough for the amplitude (mic close, or in box). The phase behaviour is in my view a bit more difficult, but doable. I use f.i. Acourate to combine arta measurement and vituixcad baflle step result to convolve into a reasonable amplitude and phase/time response. But i also see that Arta or Vituixcad give not exactly same results.
I am using Acourate to create amplitude and time linear 3-way filter, and although using measurements at about 330mm the result measured at 1000mm is correct.
I am working on the time/phase behaviour below 400 hertz, the results sofar vary by some 30-50 degrees in phase. Not yet clear to me what is the cause. It can well be the applications in use ( the math used for f.i. minimum phase) , or the microphone (again here how its phase response is defined) . But relative to the normal (not corrected) phase of the speaker it is minor.
There is sufficient results published that shows that rounding helps in damping the diffraction effects correlated with measurements. The baffle step effect for rectangular shaped baffles is max about 7 dB at 3000mm in my experience and compared to 1000mm distance the difference is less than 1 dB lower at 50hz, and less than 1 dB higher at ~ 1000hz., and null dB at ~400hz.
Note i make a distinction between baffle step and baffle diffraction, because the effects are in different frequency ranges.
Baffle step in my Gaya2 case is mostly below 400 hertz, and a little bit above. Diffraction is upwards from ~700 hertz with a bump at frequency ~box width, followed by a dip etc. Shaping the baffle smoothens the diffraction effects, and in fact can be used to shape the driver response a bit.
Baffle step is a given. In my case at ~400 hertz the baffle step responses at 330mm 1000mm and 3000mm cross each other.
Measurements below ~ 400hertz at distances of more than very close is practical always compromised because of boundaries present, but combining measurements has shown to be close enough for the amplitude (mic close, or in box). The phase behaviour is in my view a bit more difficult, but doable. I use f.i. Acourate to combine arta measurement and vituixcad baflle step result to convolve into a reasonable amplitude and phase/time response. But i also see that Arta or Vituixcad give not exactly same results.
I am using Acourate to create amplitude and time linear 3-way filter, and although using measurements at about 330mm the result measured at 1000mm is correct.
I am working on the time/phase behaviour below 400 hertz, the results sofar vary by some 30-50 degrees in phase. Not yet clear to me what is the cause. It can well be the applications in use ( the math used for f.i. minimum phase) , or the microphone (again here how its phase response is defined) . But relative to the normal (not corrected) phase of the speaker it is minor.
True. But not exactly big news. This has been common wisdom for a few decades. Take a look at things like the "Spinorama" and power response. What you hear is the soundfield in the room....it becomes evident, that even without taking any room response (such as first reflections, echos and the like) into consideration, measuring at 1m and listening at 3m will not be the same in terms of frequency response. Therefore, relying to a measurement from 1m distance to optimize a set of xover-filters, and then later on listen to the result at 3m may lead to a mismatch between the filters and the actual frequency responses and thus to an awkward and unwanted experience.
The driver itself is also a baffle.This is an important fact in real-world speakers and for the higher frequency range!
It may contribute to the pleasant reproduction of small-sized baffles, where the width of the baffle roughly corresponds with the diameter of the driver(s). This also goes for dipole baffles, where in the high frequency range it is best to operate the drivers naked, or on a very slim baffle: https://linkwitzlab.com/models.htm#A3. And by the way, horn loudspeakers with baffleless horn mouths also might show no or very low BDS artefacts. In horns, the hopefully well-shaped horn mouth is quasi it's own baffle.
Therefore, it seems wise to minimize the baffle sizes to not substantially be larger than the largest speaker attached to it. And also, to gradually slim down the baffle to match the smaller structures of the the mid driver(s) and the tweeter (except if you use a large waveguide). Speaking of waveguides, you even might design a small waveguide for the tweeter, not so to even out polar response towards high frequencies, but to match the outer diameter of the tweeter itself at the mouth of the wavegide. This would also even out the dome diameter vs. the baffle width mismatch a bit.
Yeah, holy grail would be not to have physical driver. Until that, we have drivers and associated support structures alias speakers, that interact with sound and that's we have to deal with, the reality 🙂
So, optimize the structure acoustically and the measurement problems go away. Actually these are not measurement problems but problems with the device physical size and shape that the measurements show. Most speakers are made visually attractive, or certain size and shape for many reasons like ease and cost, or blindly optimized with box simulator for good bass which deals only with bass (driver main resonance), while the outside dimensions are even more important for mids and highs. Basically what is good for bass (long wavelength) is bad for mids and highs (short wavelength) so be careful what to optimize as there is always a trade-off elsewhere. Same goes the otherway around, good stuff for highs dowsn't work for lows. Everything is due to sound wavelength being very long on lows and very short on highs, and our physical structures are static size and not changing per frequency, so the structure works nicely only for some relatively narrow bandwidth. That's why we have multiway speakers with various sized drivers. Make sure the physical structure is appropriate for the wavelengths as well.
Kind of basic stuff, very philosophical as well, and not easy to see or understand at beginning of hobby as we cannot see sound, other than from measurements, and it takes time to make mental connection between sound and how it interacts with physical objects and visual graphs of it. If and when one starts to pay attention to this stuff it's quaranteed speakers sound bit better after. Not quaranteed by me, but you the diyperson, as you now pay attention to this stuff and juggle the compromises better payibg more attention to trade-offs.
So, optimize the structure acoustically and the measurement problems go away. Actually these are not measurement problems but problems with the device physical size and shape that the measurements show. Most speakers are made visually attractive, or certain size and shape for many reasons like ease and cost, or blindly optimized with box simulator for good bass which deals only with bass (driver main resonance), while the outside dimensions are even more important for mids and highs. Basically what is good for bass (long wavelength) is bad for mids and highs (short wavelength) so be careful what to optimize as there is always a trade-off elsewhere. Same goes the otherway around, good stuff for highs dowsn't work for lows. Everything is due to sound wavelength being very long on lows and very short on highs, and our physical structures are static size and not changing per frequency, so the structure works nicely only for some relatively narrow bandwidth. That's why we have multiway speakers with various sized drivers. Make sure the physical structure is appropriate for the wavelengths as well.
Kind of basic stuff, very philosophical as well, and not easy to see or understand at beginning of hobby as we cannot see sound, other than from measurements, and it takes time to make mental connection between sound and how it interacts with physical objects and visual graphs of it. If and when one starts to pay attention to this stuff it's quaranteed speakers sound bit better after. Not quaranteed by me, but you the diyperson, as you now pay attention to this stuff and juggle the compromises better payibg more attention to trade-offs.
Last edited:
Something philosophical.
When i studied Naval architecture, i was in the first class (1977) allowed to use an electronic calculator instead of a side rule in the final exams. In my year of practical during the study i was at the delft universiteit in the ship structure lab. There they made typical steel structures scaled and deformed these under load. The sole reason for this was that students were so focused on use of computer for simulations, they forgot to correlate with reality.
I was once a chief engineer working on the walrus submarine class doing detailed design of the steel structure. The design criteria we had to meet athough simple were based on enless tests and well thought through observations of what happens under shock loads by underwater explosions.
No fem etc, just these simple criteria a slide rule and a desktop calculater limited to a 4x4 matrix. The mission of a sub is in very short form "silent kill".
Ask the US navy how well these subs are. ;-)
Given the limitations we had to in our mind live through these shock loads and apply the criteria and the philosophy behind these.
As a lasting effect even today i always want to understand what i observe when doing f.i. speaker design, and then see how to correlate sims with reality. It is very easy to make a small mistake or to leave out something.
We have fine tools today: Arta, Vituixcad, Acourate, Excel, microphones, etc to name a few, however to make the results match with reality is the key.
And then the final observation: what we hear ;-)
When i studied Naval architecture, i was in the first class (1977) allowed to use an electronic calculator instead of a side rule in the final exams. In my year of practical during the study i was at the delft universiteit in the ship structure lab. There they made typical steel structures scaled and deformed these under load. The sole reason for this was that students were so focused on use of computer for simulations, they forgot to correlate with reality.
I was once a chief engineer working on the walrus submarine class doing detailed design of the steel structure. The design criteria we had to meet athough simple were based on enless tests and well thought through observations of what happens under shock loads by underwater explosions.
No fem etc, just these simple criteria a slide rule and a desktop calculater limited to a 4x4 matrix. The mission of a sub is in very short form "silent kill".
Ask the US navy how well these subs are. ;-)
Given the limitations we had to in our mind live through these shock loads and apply the criteria and the philosophy behind these.
As a lasting effect even today i always want to understand what i observe when doing f.i. speaker design, and then see how to correlate sims with reality. It is very easy to make a small mistake or to leave out something.
We have fine tools today: Arta, Vituixcad, Acourate, Excel, microphones, etc to name a few, however to make the results match with reality is the key.
And then the final observation: what we hear ;-)
Thank you for all this input. I would like to point out once again that my simulations are of bare theoretical nature and so they show worst-case. And I am talking about consequences for the direct sound, not including any room interference. In practice and because a real driver is no point source, and because a real driver will show some beaming towards higher frequencies (thus no more spreading the same sound pressure to the periphery), the consequences of the baffle artefacts will be substantially smoother than what is showed in the graphs. And of course the room will contribute to the overall response at the listening position.
Last not least: Sorry for the title, which got a bit too intriguing and boulevard journalistic-style. I am aware that here I do not present findings, but rather facts. So to say no new stuff, but rather newton stuff.
Nevertheless the graphs show what they show:
The following and hopefully last graphs are simulations for a 34.3cm circular baffle with a central point source, stepwise simulated for measuring distances at 1d (34.3cm), 2d (68.8cm), 4d (137.2cm) and 6d (205.8cm). Reference distance for the difference graph ist as before 8.7d == 3m. You may draw your own conclusions.
Time domain:

1d:

2d:

4d:

6d:

Last not least: Sorry for the title, which got a bit too intriguing and boulevard journalistic-style. I am aware that here I do not present findings, but rather facts. So to say no new stuff, but rather newton stuff.
Nevertheless the graphs show what they show:
- For "lower" frequencies you may measure quite close to the driver without any penalties for the results for the direct sound at the listening distance.
- For "higher" frequencies it is best to measure at a distance closer to the listening distance. You may then apply some windowing to the resulting pulse in order to null out everything that is influenced by the room response. E.g. the part of the impulse starting with the first reflection which probably will be the floor reflection.
The following and hopefully last graphs are simulations for a 34.3cm circular baffle with a central point source, stepwise simulated for measuring distances at 1d (34.3cm), 2d (68.8cm), 4d (137.2cm) and 6d (205.8cm). Reference distance for the difference graph ist as before 8.7d == 3m. You may draw your own conclusions.
Time domain:
1d:
2d:
4d:
6d:
Last edited:
Can you descibe the frequency pictures in more detail? I do not understand what they are showing.
.. You may draw your own conclusions.
Yeah, what you've shown in the thread is true and valid but I see you are on your personal path to final conclusion about the subject but not there yet, currently just noticing there is something going on and observing what it is on the graphs and I bet soon you'll get to some conclusion with it, if you already didn't.
I'll help, hopefully, by asking what's the difference if secondary sound comes 0.5ms or 0.6ms later as you show here? And the answer is not much, other than observing the interference ripple changes some, the graphs change when you move the mic but the secondary sound is still present there no matter where the mic is. The important bit is to realize that there is secondary sound source and it interferes with direct sound and while it's there it'll always interfere and there is no observation angle that is not affected by it. You have now identified a phenomenon and can now work with it as you understand it quite well, and can identify it from sim and measurement graphs.
Now, all you need to do is try and listen for it whether it matters, do you find it audible? does sound change if you move your ear?🙂 then you could do something about it, use the knowledge to reduce or eliminate the secondary sound source. Otherwise you can just ignore it regardless of mic position because you see from the graph there is diffraction present but you don't need to do anything about it and not care about where the ripple is at with any mic position.
So, if you are making some ultimate system it would be better to get rid of the secondary sound source altogether. As you'll find out the sound and secondary impulse would change if you moved the mic to any direction, not just with distance so you can never correct it with EQ except only to one observation angle. But, you can calculate from the impulses or interference where the secondary sound originates from and can do something about it, reduce it to fix all observation angles at once. But as we already know it's the baffle edge in this case you can condense it to simple rules of thumbs, like remove the edge, or make the edge as close to the transducer as possible, or as far as possible so that it mixes with all the room reflections, to effectively remove the secondary sound source 😉 And when you do, you'll likely start noticing other related stuff relating wavelength and how sound interacts with objects and soon you'll simulate simple systems in your head.
I bet you are having fun, I had the same phase with edge diffraction at some point and basically this post describes what happened to me, I don't know you or what your learning phase is so don't take the post personally. I still do this kind of personal discoveries with various aspects to speakers and sound which keeps this hobby much fun. Sometimes can't even sleep trying to imagine how things work and what it means perceptually 🙂 I hope you are having much fun as well!
ps. there are many offshoots from your posts, you likely now pay better attention how to measure things, how to utilize simulations to reason about things, perhaps mental simulation of multiple sound sources interact considering wavelength, and that interference is not just destructive but also constructive, what bafflestep is, why there is the "main diffraction hump" and how to utilize these to your advantage, and so on 😀 In general, how wavelength is fundamental basis to imagine acoustics and speakers.
Last edited:
- Home
- Loudspeakers
- Multi-Way
- One single driver with several frequency responses. What the heck?