If I have a woofer with QTC of 1.5 and I equalize it so the roll off looks like 0.7, will the damping behave like an uncompensated woofer with a QTC of 0.7?
I recall Floyd Toole saying electronic compensation of resonances in rooms also fixes the ringing in the time domain, so I assume this would be the same for woofers. It's the system that rings, am I right?
I recall Floyd Toole saying electronic compensation of resonances in rooms also fixes the ringing in the time domain, so I assume this would be the same for woofers. It's the system that rings, am I right?
You can easily eq the peaky response,but taming undamped ringing in pretty hard as far as I know,it'd be easier to invest in a nicer woofer.Actually as i was writing this I remembered you can use "resistive" enclosures,like a sealed enclosure with a lot of tiny holes in the back panel,but again,if you want rattling bass,better get a nicer woofer.
If you EQ the frequency response to get the same response as a Qtc=0.7 system, you get the same impulse response as a Qtc=0.7 system aswell, hence reduced ringing.
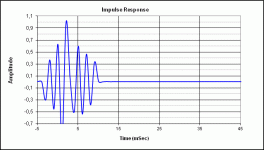
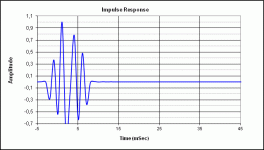
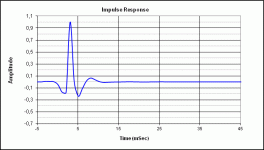
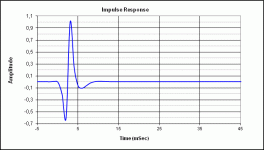
1. pic is Qtc 1.5
2. pic is Qtc 1.5 + 1 EQ to reduce the main hump caused by high Qtc
3. pic is Qtc 1.5 + more EQ to get more similar frequency response to Qtc 0.7, it's just a fast estimation on EQ to visualize the reduced ringing
4. pic is Qtc 0.7
Simulated with Jeff Bagby's Woofer Box Model and Circuit Designer v 5.0
Loudspeaker Design Software
1. pic is Qtc 1.5
2. pic is Qtc 1.5 + 1 EQ to reduce the main hump caused by high Qtc
3. pic is Qtc 1.5 + more EQ to get more similar frequency response to Qtc 0.7, it's just a fast estimation on EQ to visualize the reduced ringing
4. pic is Qtc 0.7
Simulated with Jeff Bagby's Woofer Box Model and Circuit Designer v 5.0
Loudspeaker Design Software
I've been told that that is true but it continues to defy my intuition.
A driver (mounted in a box or otherwise) has a fundamental bass resonance and the Q is attached to it. Like a bell, that resonance (with that Q) adheres to the driver (or driver-in-box) although it can be changed in lots of acoustic ways.*
With band-cut EQ, the music can stimulate the driver less at the resonance and so the FR is better and the impulse response is better, as denibeni shows.
But the box still rings at its inherent frequency and Q from any stimulation that may come its way, in the pure sound signal or otherwise.
Anyway, that's my ignorant intuition on the matter.
B.
* you can put an ESL inside a boxe filled with heavy gas, put a dab of heavy putty on a cone dust cap, or....
Last edited:
If you put a loudspeaker driver in a box you get a system which from now on are inseparable. The problem with high Qtc is that the driver does not have enough force to control the airspring enclosed in the box and begins to ring.
Actually to get the same response as 0.7 from 1.5 you need a lot of EQ boost and not cut. You need to pump much more power to the driver at the right frequencies to be able to compress the air in the box and thus reduce system ringing.
The only cut is need is where the SPL is more than with a 0.7 system, which is the main hump at higher frequencies before the roll-off begins.
Last edited:
If you want to view it from this perspective, then consider that the filter also rings. It just rings in such a manner that it cancels the ringing of the woofer+box. See the figures at the header '9 - 12 dB/oct highpass equalization ("Linkwitz Transform", Biquad)' on this website: Active Filters
Last edited:
If that is true and it it has any practical significance in addressing speaker resonance (which I doubt), it still is functioning only on the input-signal like EQ.
The tuned box is still standing in the corner with its characteristic sound.
Yes, in the perfect (read: textbook simplified) math world, you can perfectly counteract input signals that would activate the tuned box.
B.
As TBTL said, the EQ ringing counteract the box+driver ringing, and not only in the perfect mathematic world, but in real world too, but it needs a lot of power from the amp.
Yeah, if you put this box in the room's corner that's another story, but this can be EQ-ed again.
I think the main issue will be accuracy. The parameters of the box are only approximately known and the filter components have tolerances, so the filter's zeros will never exactly cover the poles of the box. Practically this means that there will be some Q = 1.5 ringing left, but with a much reduced level compared to the case without equalization.
Besides, bentoronto's remark about the response of the box to acoustical reflections is of course correct, so even with perfectly accurate box and filter parameters, there would still be some trace of the original Q = 1.5 response left. Then again, a real room will probably be full of high-Q resonances.
Besides, bentoronto's remark about the response of the box to acoustical reflections is of course correct, so even with perfectly accurate box and filter parameters, there would still be some trace of the original Q = 1.5 response left. Then again, a real room will probably be full of high-Q resonances.
C'mon now, you are not getting the point.
Anything to do to the EQ affects only the input signal. Kind engineering arrogance to believe that you control all the stimuli just because you control the electric signal to some degree.
And also MarcelvdG is right. Again, engineering arrogance to imagine you can know precisely how the speaker-in-the-room will behave on different days of the week just because your sim gives you an "answer" to three decimal places.
B.
Yes, in the real world, perfectly get Qtc 0.7 from 1.5 is very hard or impossible but when it comes to practicality, we have to mention how the end result would sound, and I don't think anyone would say after using a couple of adequate filters in the right place that the original Qtc was 1.5. Or seeing from the impulse response.
I may not be right, but it would be interesting to give it a try.
I may not be right, but it would be interesting to give it a try.
You forget that, if you alter the input signal you alter the power delievery to the speaker driver and the driver acts like a stronger driver if you want to reduce the Qtc with EQ and if the amp can push out that power.
@bentoronto : You are indeed correct that the signal does not change the response of the passive loudspeaker - it goes unchanged.
If you had a resonance peak at, say, 60Hz of 10dB, it means that whatever signal you send to it at 60Hz will be boosted by 10dB. What the electronic EQ-ing of the signal is doing is simply to dial down that frequency point by 10dB, so it sits at -10dB. Thus, the -10dB signal comes along, gets boosted by 10dB (the loudspeaker), yielding a sum of 0dB. This results in the total response at 60Hz being 0dB (A.K.A. flat).
Of course, the speaker's frequency response is a curve, and doing a complete job entails constructing an inverse curve to match the particular loudspeaker (i.e., more frequency points are adjusted).
Similar to the speaker, the room itself has its own response, so EQ-ing of the total system is best applied to a room measurement of said system.
If you had a resonance peak at, say, 60Hz of 10dB, it means that whatever signal you send to it at 60Hz will be boosted by 10dB. What the electronic EQ-ing of the signal is doing is simply to dial down that frequency point by 10dB, so it sits at -10dB. Thus, the -10dB signal comes along, gets boosted by 10dB (the loudspeaker), yielding a sum of 0dB. This results in the total response at 60Hz being 0dB (A.K.A. flat).
Of course, the speaker's frequency response is a curve, and doing a complete job entails constructing an inverse curve to match the particular loudspeaker (i.e., more frequency points are adjusted).
Similar to the speaker, the room itself has its own response, so EQ-ing of the total system is best applied to a room measurement of said system.
Last edited:
Yes, sorry I didn't make that clear. QTC does imply a sealed box, not a loose woofer.
Sorry, I responded inside the quote of your post, which is super confusing. Re posting what I said previously, since I can't edit the post above:
Thank you, this exactly what I was looking for.
What I wish to do is use a cheap midwoofer with a high Q (Vifa TC9FD) and cross that over to a sub around 200hz. The TC9FD has other good qualities like low distortion and good power handling. The enclosure size is constrained, so the Q must be high or I have to used another woofer. The response will be flat, but the roll off will be different than 0.7.
I'll try "Woofer Box Model and Circuit Designer v 5.0" and see if I can simulate it.
Yes.
A parametric equalizer will do it.
Set it at resonance frequency fc, Q at 1.5 and level at -6.5 dB.
It is easy to check it in simulation.
With two more parametric equalizations you can set new (apparent) fc, Qtc as you like just as a Linkwitz transform. I do it since years.
Last edited:
Thanks, I will try that. I am using a minidsp with manually programmed filter coefficients, I think I can use the parametric EQ in conjunction.
I was planning to use the high pass filter counteract the bump, and was assuming I would get something near flat with a steeper rolloff.