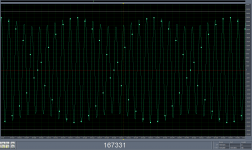
A waveform is the overall. The envelope is the height of the waveform over time.Thanks!
Ok so the "fish" would never "emerge" if you run the signal in slow-mo and watched a level meter follow the *envelope*! The envelope is that path you would travel if you "walked" the signal - right?
Hmm, still wondering... when you use waveform and envelope in the same sentence - must mean they are different for you - how?
//
The "fish" was used to illustrate that as the frequency of interest gets closed to the nyquist rate, the envelope information is no longer as easy to figure out. Remembermber, the fish input was a steady state sine.
Jn
It is indeed quite pleasurable. And the reason I may hold off retirement for a while.Perhaps you could get some funding for some cutting edge research? Think of the emotional pleasure it could bring.
Richard, we just got funded for a large machine, gonna be quite busy for a decade or so.
Jn
The problem is, my dear Jakob, that I can easily demonstrate that it is the speaker that creates IM. The amp IM is about 50dB better than that of the speaker.
2 plots, one with L channel playing 22kHz and R channel 27kHz. This shows that microphone is clean and does not create distortion.
2nd plot, one speaker plays 22+27kHz tones and we can see IMD products in audio band.
Dear Jakob, this is the issue that Oohashi did not take into account. He should have used 2 speakers instead of one, one for ultrasound, one for <20kHz programme.
You know I do not need weeks of discussions to prove somebody wrong.
That’s what I call a constructive contribution instead of just endless streams of words.
I was about to do this test myself, but your plots are saving me the trouble.
Thanks.
Hans
Thank you, Hans. As you know, I am not a friend of endless debates that often have not much point.
When are the sidebands created?Too much of a blanket.
This story contains two parts. First is the filter before the sampler. It prevents violating nyquist.
The math algorithm is a simple one which by definition, produced two pure sines.
The filter removes one. All this has nothing to do with sample rate. The filter sample rate needs to be high enough to NOT remove pertinent envelope information. If the filter worked at a gig rate but removes 22.5k, the same thing will happen.
Your I/O difference Gibbs plot shows exactly where the filter removes content, the Gibbs envelope might be used to determine if it's fundamental frequency content being removed (which we do not care about), or envelope created sidebands.
A waveform is the overall. The envelope is the height of the waveform over time.
The "fish" was used to illustrate that as the frequency of interest gets closed to the nyquist rate, the envelope information is no longer as easy to figure out. Remembermber, the fish input was a steady state sine.
Jn
Overall... Is the "fish" a waveform?
Is this a correct sentence? "The envelope had a certain waveform."
Sorry JN, just want to undertand you. Terminology is crucial.
//
When are the sidebands created?
When the signal amplitude is modulated.
The math for a sine modulation is simple and predictable. The math for strimikg a cymbal or xylophone, not so.
Jn
The fish is a signal with an envelope that looks like a fish as sampled. The problem is it was the result of sampling a constant sine near 2x.Overall... Is the "fish" a waveform?
Is this a correct sentence? "The envelope had a certain waveform."
Sorry JN, just want to undertand you. Terminology is crucial.
//
Don't overthink it.
Jn
Electrical, not acoustical?When the signal amplitude is modulated.
The math for a sine modulation is simple and predictable. The math for strimikg a cymbal or xylophone, not so.
Last edited:
Electrical, not acoustical?
He permanently complains on this (21k/44.1k), which is a reconstruction issue, not sampling issue. This about hundred times explained by Scott Wurcer.
Attachments
It is indeed quite pleasurable. And the reason I may hold off retirement for a while.
Richard, we just got funded for a large machine, gonna be quite busy for a decade or so.
Jn
🙂 😎 Great. More challenging problems to solve ! New ideas and solutions. wow.
I'm drooling.
-Richard
Last edited:
Incoherent John, you simply type too fast. There is no way a filter gets a sample rate before the sampler. And where is the second part? 😀... This story contains two parts. First is the filter before the sampler. It prevents violating nyquist.
... All this has nothing to do with sample rate. The filter sample rate needs to be high enough to NOT remove pertinent envelope information...
@ PMA & Hans Polak,
The main problem, dear PMA, is that you are simply wrong about Oohashi et al.'s experimental procedure.
Contrary to your assertion, they (for example in experiment 2) in fact used one speaker system for the audio band up to 22 kHz and an _additional_ "super tweeter" for the reproduction of the so-called ultrasonic content above 22 kHz.
Further as you, dear PMA, have expressed recently:
which seams quite reasonable as a hypothesis, so lets refer to Oohashi et al.'s publication where the authors state explicitely:
"None of the subjects recognized the HFC as sound when it was presented alone"
(note:the term "HFC" denotes high frequency content)
<snip>
2 plots, one with L channel playing 22kHz and R channel 27kHz. This shows that microphone is clean and does not create distortion.
2nd plot, one speaker plays 22+27kHz tones and we can see IMD products in audio band.
Dear Jakob, this is the issue that Oohashi did not take into account. He should have used 2 speakers instead of one, one for ultrasound, one for <20kHz programme.
You know I do not need weeks of discussions to prove somebody wrong.
The main problem, dear PMA, is that you are simply wrong about Oohashi et al.'s experimental procedure.
Contrary to your assertion, they (for example in experiment 2) in fact used one speaker system for the audio band up to 22 kHz and an _additional_ "super tweeter" for the reproduction of the so-called ultrasonic content above 22 kHz.
Further as you, dear PMA, have expressed recently:
The audibility is questionable. Yes in case of quiet environment and not too far from speakers. Probably not if masked by music programme.
which seams quite reasonable as a hypothesis, so lets refer to Oohashi et al.'s publication where the authors state explicitely:
"None of the subjects recognized the HFC as sound when it was presented alone"
(note:the term "HFC" denotes high frequency content)
Incoherent John, you simply type too fast. There is no way a filter gets a sample rate before the sampler. And where is the second part? 😀
Oh, ok. How does a brickwall in front of a sampler work?
Jn
@ PMA & Hans Polak,
The main problem, dear PMA, is that you are simply wrong about Oohashi et al.'s experimental procedure.
Contrary to your assertion, they (for example in experiment 2) in fact used one speaker system for the audio band up to 22 kHz and an _additional_ "super tweeter" for the reproduction of the so-called ultrasonic content above 22 kHz.
Further as you, dear PMA, have expressed recently:
which seams quite reasonable as a hypothesis, so lets refer to Oohashi et al.'s publication where the authors state explicitely:
"None of the subjects recognized the HFC as sound when it was presented alone"
(note:the term "HFC" denotes high frequency content)
So we have Nyquist deniers, Fourier deniers and Oohashi deniers.
I’m happy to belong to the 3rd category. 😀 😀
Hans
Your statement, you tell me. I told you I am no good at this. I only know everything before a sampler is analog, no sample nor sample rate.Oh, ok. How does a brickwall in front of a sampler work?...
Again, where is the second part?
Last edited:
So we have Nyquist deniers, Fourier deniers and Oohashi deniers.
I’m happy to belong to the 3rd category. 😀 😀
Hans
Nice one. 🙂
There are good reasons to have doubts wrt the conclusions from Oohashi et al. (I could supply some arguments myself), but I hope that we can agree that it is no justification to spread false assertions about their experimental process.
In signal processing, sampling is the reduction of a continuous-time signal to a discrete-time signal. A common example is the conversion of a sound wave (a continuous signal) to a sequence of samples (a discrete-time signal).
A sample is a value or set of values at a point in time and/or space. A sampler is a subsystem or operation that extracts samples from a continuous signal. A theoretical ideal sampler produces samples equivalent to the instantaneous value of the continuous signal at the desired points.
The original signal is retrievable from a sequence of samples, up to the Nyquist limit, by passing the sequence of samples through a type of low pass filter called a reconstruction filter.
WiKi high lights the words continuous (CW).
If you have non- continuous signal and transients instead, then a higher sampling rate is needed for accurate capture.
THx-RNMarsh
Sampling (signal processing - Wikipedia)
A sample is a value or set of values at a point in time and/or space. A sampler is a subsystem or operation that extracts samples from a continuous signal. A theoretical ideal sampler produces samples equivalent to the instantaneous value of the continuous signal at the desired points.
The original signal is retrievable from a sequence of samples, up to the Nyquist limit, by passing the sequence of samples through a type of low pass filter called a reconstruction filter.
WiKi high lights the words continuous (CW).
If you have non- continuous signal and transients instead, then a higher sampling rate is needed for accurate capture.
THx-RNMarsh
Sampling (signal processing - Wikipedia)
Last edited:
Correct me if I’m wrong but isn’t cd already going the way of the 8 track/cassette?
Streaming seems to be the future, recording engineers are starting to embrace the higher sample rates, no?
Downsampling new recordings to Redbook for the cd market will be the norm if it isn’t happening already.
I don’t see keeping up with technology as a bad thing......I haven’t used my CD player but a couple time in over a year after a/b’ing the cd to the same Redbook version of my streaming service.
Streamer won if that wasn’t obvious! 😎
Basically what I’m getting at is none of this really matters?
Edit.....and the newly recorded material done 24/96+ from the get go is awesome, trying to figure out what’s what is a pita though.
Streaming seems to be the future, recording engineers are starting to embrace the higher sample rates, no?
Downsampling new recordings to Redbook for the cd market will be the norm if it isn’t happening already.
I don’t see keeping up with technology as a bad thing......I haven’t used my CD player but a couple time in over a year after a/b’ing the cd to the same Redbook version of my streaming service.
Streamer won if that wasn’t obvious! 😎
Basically what I’m getting at is none of this really matters?
Edit.....and the newly recorded material done 24/96+ from the get go is awesome, trying to figure out what’s what is a pita though.
Last edited:
The original signal is retrievable from a sequence of samples, up to the Nyquist limit, by passing the sequence of samples through a type of low pass filter called a reconstruction filter.
Is it the reconstruction filter that makes the envelope created sidebands jn is referring to?
Last edited:
- Status
- Not open for further replies.
- Home
- Member Areas
- The Lounge
- John Curl's Blowtorch preamplifier part III