GedLee comments a lot on the shape of the nonlinearity correlating to it's audibility. So my question would be "what is the shape of the nonlinearity?" The next logical question would be "How is it measured?" then "what would be the worst possible shape?"
Thanks! Danke! Dalu! Merci! Grazie! Arigato! Mahalo!
Thanks! Danke! Dalu! Merci! Grazie! Arigato! Mahalo!
I am not Dr. Geddes, but I would surmise that what you are asking about is the "GedLee Metric". Think you have to buy his book to get closer to it... hopefully he will give us some more useful data and information on this...
Don't know if it's the GedLee Metric, but from the sounds of it he must have exclusive knowledge of it?  maybe he's not sharing... Guess we'll see, but it's useless to all if we can't see it to paraphrase his own words from another thread.
maybe he's not sharing... Guess we'll see, but it's useless to all if we can't see it to paraphrase his own words from another thread.
 maybe he's not sharing... Guess we'll see, but it's useless to all if we can't see it to paraphrase his own words from another thread.
maybe he's not sharing... Guess we'll see, but it's useless to all if we can't see it to paraphrase his own words from another thread.anything that deviates from a perfect sine wave:
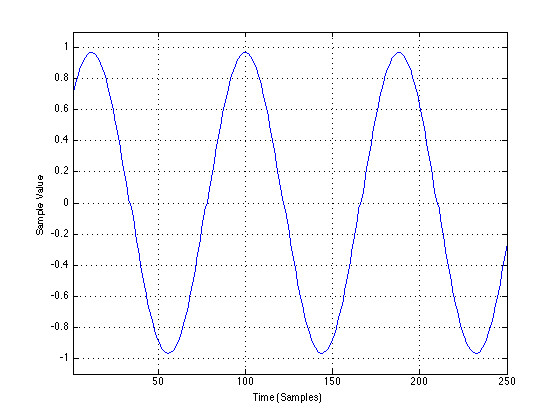
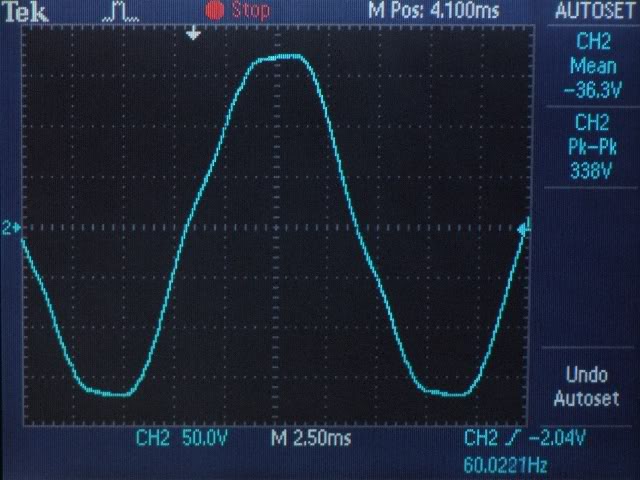
The distortion is made up of harmonic content (multiples of the original frequency). It can be measured by hooking up the output of the amp to the input of a PC soundcard and analysing the spectrum. Of course an attenuator has to be used (to prevent blowing up the soundcard) and care taken to ensure that the ground loop isn't made and that grounds are isolated if need be.
Total harmonic distortion - Wikipedia, the free encyclopedia
http://www.vitalstates.org/diy/amplifiers/simple-thd-measurement.pdf


The distortion is made up of harmonic content (multiples of the original frequency). It can be measured by hooking up the output of the amp to the input of a PC soundcard and analysing the spectrum. Of course an attenuator has to be used (to prevent blowing up the soundcard) and care taken to ensure that the ground loop isn't made and that grounds are isolated if need be.
Total harmonic distortion - Wikipedia, the free encyclopedia
http://www.vitalstates.org/diy/amplifiers/simple-thd-measurement.pdf
The shape of the non-linearity, as I understand him, is simply the distribution and strength of the distortion components. For example, a sine wave with only 20% F2 (2nd harmonic) looks completely different than a sine wave with only 20% F5, although both have 20% THD. This is important because you can't tell from the THD number alone how audible the distortion is. In this example, if the sine wave has 40 Hz, the 20% F2 are hardly audible, but the 20% F5 are audible for sure.
That's the main aspect of the GedLee metric, weighting higher order harmonics more than lower order*. Additionally, there's a weighting towards lower levels, because at higher levels our ear becomes less sensitive to distortion (although I think that at very low levels the audibility of distortion is lower than at low to medium levels; maybe there's still room for improvements in the metric).
*The GedLee metric does not evaluate the harmonics, but the non-linear transfer function.
That's the main aspect of the GedLee metric, weighting higher order harmonics more than lower order*. Additionally, there's a weighting towards lower levels, because at higher levels our ear becomes less sensitive to distortion (although I think that at very low levels the audibility of distortion is lower than at low to medium levels; maybe there's still room for improvements in the metric).
*The GedLee metric does not evaluate the harmonics, but the non-linear transfer function.
Last edited:
Harmonic masking is also a factor, as alluded to in the post above. There is research on that going back to before any of us were born.
One thing to take into account though is that HD at sub frequencies are much more audible compared to the main tone. Equal-loudness contour - Wikipedia, the free encyclopedia
It wasn't until I got a better sub that I could play and hear pure enough 20-30 hz sine waves and then realize that they sound very little. On my inferior speaker I probably listened to the distortion which was much more audible.
It wasn't until I got a better sub that I could play and hear pure enough 20-30 hz sine waves and then realize that they sound very little. On my inferior speaker I probably listened to the distortion which was much more audible.
It's not just subs. Many stage bass guitar combos cannot generate much bottom "E" and are mainly doubling ie 2nd harmonic
read up on the work of D.E.L. Shorter, BBC, he precedes GedLee, by several decades, and has somewhat of a similar idea. The GedLee method is not published, that I am aware, although much information is available from Dr. Geddes. My sense of it is that if you have higher math at your fingertips, his published information might be sufficient to lead you to a place where you could figure much of it out. Otherwise, I think he uses this as part of his business, so it is likely proprietary. He does acoustics and design for a living.
_-_-
_-_-
This article is related and compares the D.E.L. Shorter and GedLee weightings
Euphonic Distortion: Naughty but Nice? | Stereophile.com
Euphonic Distortion: Naughty but Nice? | Stereophile.com
wow every once in a long while something of real use and interest is published in Stereophile! thanks for bringing this article to my/our attention.
Of course I missed this one by more than half a decade... hmmm...
_-_-
Of course I missed this one by more than half a decade... hmmm...
_-_-
Keith Howard has his own website AudioSignal
with some interesting freeware for adding simulated harmonic distortion and tracing distortion as well as other toys
with some interesting freeware for adding simulated harmonic distortion and tracing distortion as well as other toys
I was never referring to the GedLee metric, just the "shape" of the nonlinear transfer characteristic. The GedLee Metric is published and the papers are on my website.
I ceased to have interest in it after I used it to show that nonlinearity was not nearly as audible as we all (myself included) had surmised. In fact, for the most part, non linearity in a loudspeaker is a non-issue - many studies have shown this and it is Dr. Toole's opinion as well. So I became more interested in what it was that we heard when we listened to say a bad horn. It sure sounded like nonlinear distortion - but it wasn't. That too has been studied and shown. Then, logically, I became interested in how to make devices - loudspeakers - that did not suffer from the effects that I had found to be the culprit. That's what I do now.
So I do not use the GedLee metric per-see, but its development led me on a path that has completely changed the way I view audibility problems in loudspeakers.
I have used concepts form the GedLee metric to show how different amplifiers that measure the same can sound different, but amps are not my interest so I did not pursue this line any further (although it was extensively discussed here at DIY). The "first watt" concept is quite close to what I concluded on this subject.
I ceased to have interest in it after I used it to show that nonlinearity was not nearly as audible as we all (myself included) had surmised. In fact, for the most part, non linearity in a loudspeaker is a non-issue - many studies have shown this and it is Dr. Toole's opinion as well. So I became more interested in what it was that we heard when we listened to say a bad horn. It sure sounded like nonlinear distortion - but it wasn't. That too has been studied and shown. Then, logically, I became interested in how to make devices - loudspeakers - that did not suffer from the effects that I had found to be the culprit. That's what I do now.
So I do not use the GedLee metric per-see, but its development led me on a path that has completely changed the way I view audibility problems in loudspeakers.
I have used concepts form the GedLee metric to show how different amplifiers that measure the same can sound different, but amps are not my interest so I did not pursue this line any further (although it was extensively discussed here at DIY). The "first watt" concept is quite close to what I concluded on this subject.
Earl, I agree that not too much efforts should be placed in reducing speaker distortion to values far below currently achieved. But there are still drivers (or speakers with them) available, that have audible distortion - a plateau 1% @ 1 kHz @ 90 dB IS audible, although you need a good comparison to hear it with real music and not only sinusoidal test tones.
Here in Germany we have two printed DIY magazines, who measure distortion (separated by components) at 90 dB (one magazine) and 85 dB/95 dB (the other mag). Sometimes I see drivers who cost hundreds of Euros because they have a nifty super-stiff cone, but lack a shorting ring to cancel the flux modulation created by the insanely high inductance. Thats the reality. The easiest way to improve this situation: do not buy those drivers. Of course, I know many drivers whose distortions are well below audibility. And cost less than a hundred Euros. May have drawbacks, though, like a not-so-popular name, or the cone is made from paper... :rolleyes
With amplifiers it is a completely different situation. I have always believed that an amplifiers distortion which is somewhere in the range of 0.01% THD is not audible. But after reading your and others work, and thinking about what happens in an amplifier, especially those with feedback loops, I started to doubt. These feedback amplifiers tend to create very high orders of distortion which are a) not measured seperately and/or b) added to the THD number where the unhearable F2 or F3 dominates. I don't know yet if those high order distortions are really audible, but maybe they are. There are now a few solid state concepts available which have only moderate feedback, therefore comparably high lower order distortions (still below perception threshold), but lack the extremely high order distortions. Possibly only one of these HighEnd trends...
Here in Germany we have two printed DIY magazines, who measure distortion (separated by components) at 90 dB (one magazine) and 85 dB/95 dB (the other mag). Sometimes I see drivers who cost hundreds of Euros because they have a nifty super-stiff cone, but lack a shorting ring to cancel the flux modulation created by the insanely high inductance. Thats the reality. The easiest way to improve this situation: do not buy those drivers. Of course, I know many drivers whose distortions are well below audibility. And cost less than a hundred Euros. May have drawbacks, though, like a not-so-popular name, or the cone is made from paper... :rolleyes
With amplifiers it is a completely different situation. I have always believed that an amplifiers distortion which is somewhere in the range of 0.01% THD is not audible. But after reading your and others work, and thinking about what happens in an amplifier, especially those with feedback loops, I started to doubt. These feedback amplifiers tend to create very high orders of distortion which are a) not measured seperately and/or b) added to the THD number where the unhearable F2 or F3 dominates. I don't know yet if those high order distortions are really audible, but maybe they are. There are now a few solid state concepts available which have only moderate feedback, therefore comparably high lower order distortions (still below perception threshold), but lack the extremely high order distortions. Possibly only one of these HighEnd trends...
That it is possible to have a loudspeaker with audible nonlinearity is absolutely the case. That the opposite is also true (no audible nonlinearity in the drivers) is also the case. I always buy the later, so for me, nonlinearity in a loudspeaker is not an issue. All of my woofers use shorting rings, just to confirm your point. I would not use a woofer without one.
High feedback can be shown to move the residual harmonics upward in order. This means that lowering the THD by using more feedback may just be making the situation worse. The whole issue is complex and not at all like what people have been assuming for decades.
High feedback can be shown to move the residual harmonics upward in order. This means that lowering the THD by using more feedback may just be making the situation worse. The whole issue is complex and not at all like what people have been assuming for decades.
I was never referring to the GedLee metric, just the "shape" of the nonlinear transfer characteristic.
<snipped>
The "first watt" concept is quite close to what I concluded on this subject.
Again, the OP's question is what do you mean by "shape"?
Perhaps an example or two or image to illustrate?
How do you measure the "shape"?
A simple transfer curve or more complex visualization means?
This is intriguing.
_-_-bear
"Shape" is defined by a polynomial in x. The "order" of the harmonic is correlated with (but not identical to) the order of the polynomial. The lowest order (x) is actually the gain, the second order (x^2) is the first term to describe the shape and it will generate only a second harmonic. The third term (x^3) generates a third harmonic, but also changes the level of the fundamental - the fourth generates a fourth harmonic but also modifies the second, etc. etc.
The higher harmonics are more audible and come from the higher orders in the polynomial. Basically the sharper the transfer characteristic the more audible it is - like clipping. But clipping is (or should be) seldom if ever activated. But picture crossover distortion. It happens on every cycle and its effect is greater the lower the signal level. This kind of nonlinearity is extremely audible even at .001% THD.
So you see, its all about the shape.
Loudspeakers cannot have sharp changes in their transfer characteristics (for the most part) because it takes very high forces to do this. Hence the large second and third order nonlinearity for which they are know are basically inaudible even at 10% and above.
The higher harmonics are more audible and come from the higher orders in the polynomial. Basically the sharper the transfer characteristic the more audible it is - like clipping. But clipping is (or should be) seldom if ever activated. But picture crossover distortion. It happens on every cycle and its effect is greater the lower the signal level. This kind of nonlinearity is extremely audible even at .001% THD.
So you see, its all about the shape.
Loudspeakers cannot have sharp changes in their transfer characteristics (for the most part) because it takes very high forces to do this. Hence the large second and third order nonlinearity for which they are know are basically inaudible even at 10% and above.
Last edited:
Wow, this turned into a good thread. My math skills are probably not among the top ten contributors in this thread, but I grasped the concept. Thank you Dr. Geddes! I wish there was a simple "do or don't" list of drivers and amplifiers based on this Metric.
Thanks David! I'm going to scope out that article to see what it has to say. I hope to be adle to pin it down. Pictures are always helpful. 😉
Thanks David! I'm going to scope out that article to see what it has to say. I hope to be adle to pin it down. Pictures are always helpful. 😉
in amps some zero-crossing defects simply can't be fixed by feedback
we prefer to call such designs "broken" - its unfair to use them to bash negative feedback
if the output stage, its biasing and drive circuits can't deliver the required output I,V due to deadbands, dynamic defects - negative feedback can't do any more than to try to push it faster thru the holes in its output capability
but with a little attention even Class AB outputs can avoid gross defects - then once negative feedback levels rise to a very modest level, additional increases in negative feedback push all harmonics of the output distortion spectrum down
we prefer to call such designs "broken" - its unfair to use them to bash negative feedback
if the output stage, its biasing and drive circuits can't deliver the required output I,V due to deadbands, dynamic defects - negative feedback can't do any more than to try to push it faster thru the holes in its output capability
but with a little attention even Class AB outputs can avoid gross defects - then once negative feedback levels rise to a very modest level, additional increases in negative feedback push all harmonics of the output distortion spectrum down
I would agree that any amp that is audible is "broken". There is no need for anything in the amplifier to be audible. Thats not to say that there are no audible amps or that "broken" amps don't exist in the marletplace because they do. But these days the technology is well understood such that any audible defects in the amp can be avoided.
I would say the same thing for loudspeakers. It is possible to make a loudspeaker systen such that audible nonlinear defects can be avoided in the entire range of reasonable SPL (including say 110-120 dB). It is not possible eliminate all frequency response aberations hat exist in a loudspeaker even with complex EQ systems. This makes speakers a tough problem whose flaws cannot be fixed by electronics alone.
If you want to consider those things that are and will always be audible in an audio system, look to the 3D frequency response characteristics of the loudspeakers. That's where the most differences in design choices are to be found.
There is the thermal problem as well and the maximum output linitations (which fall in the nonlinear domain but won't be seen with THD measurements), but those can be dealt with as well - its just that they usually aren't.
I would say the same thing for loudspeakers. It is possible to make a loudspeaker systen such that audible nonlinear defects can be avoided in the entire range of reasonable SPL (including say 110-120 dB). It is not possible eliminate all frequency response aberations hat exist in a loudspeaker even with complex EQ systems. This makes speakers a tough problem whose flaws cannot be fixed by electronics alone.
If you want to consider those things that are and will always be audible in an audio system, look to the 3D frequency response characteristics of the loudspeakers. That's where the most differences in design choices are to be found.
There is the thermal problem as well and the maximum output linitations (which fall in the nonlinear domain but won't be seen with THD measurements), but those can be dealt with as well - its just that they usually aren't.
- Status
- Not open for further replies.
- Home
- Loudspeakers
- Multi-Way
- Nonlinearity, what's the SHAPE of it?